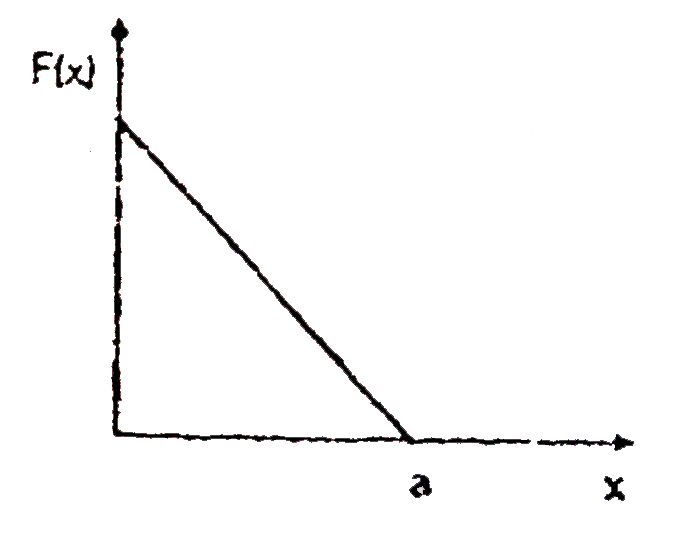
Potential Energy U(x) and associated force F(x) bears the relation F(x)=−ddxU(x).
Dependence of potential energy of a two−particle system separation x between them is shown in the following figure.
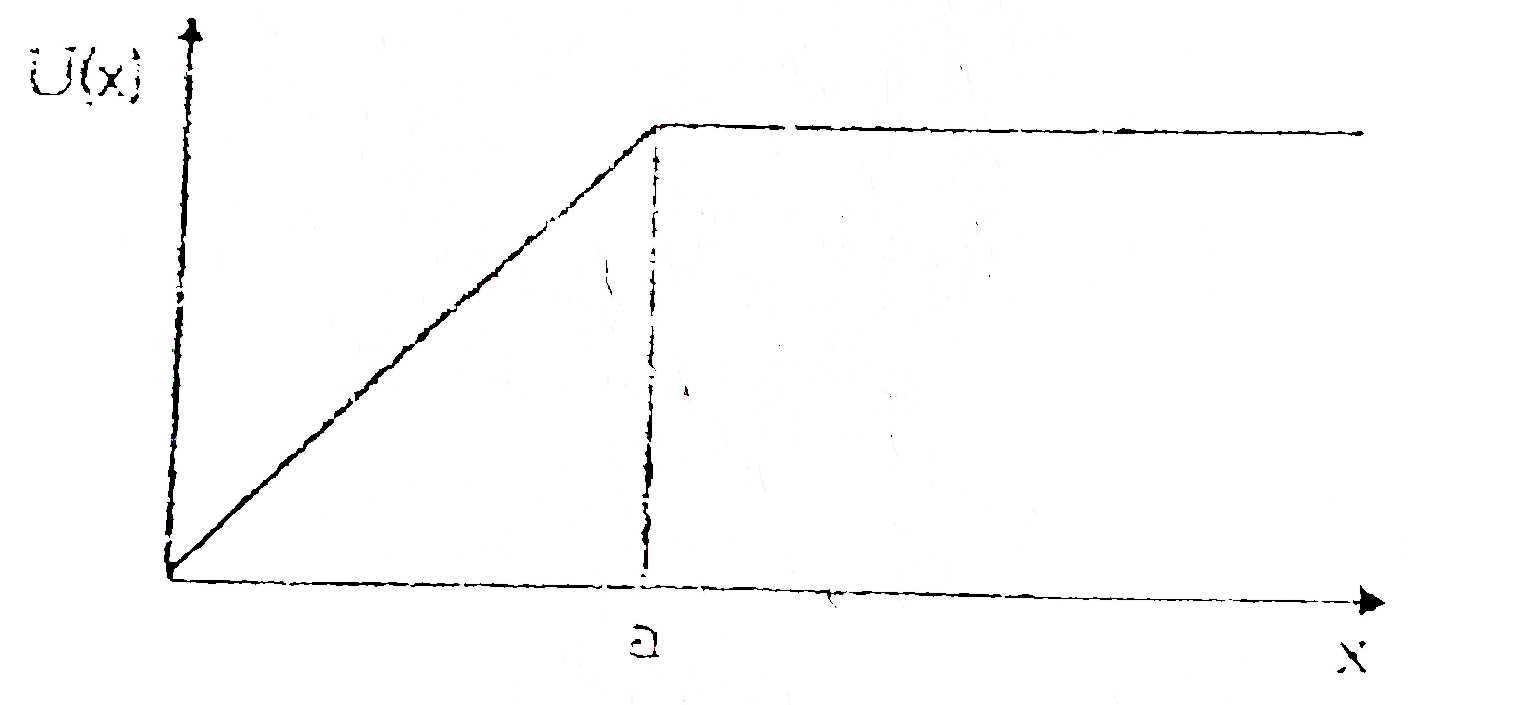
Which of the following graphs shows correct variation in assoicated force F(x) with x?
A
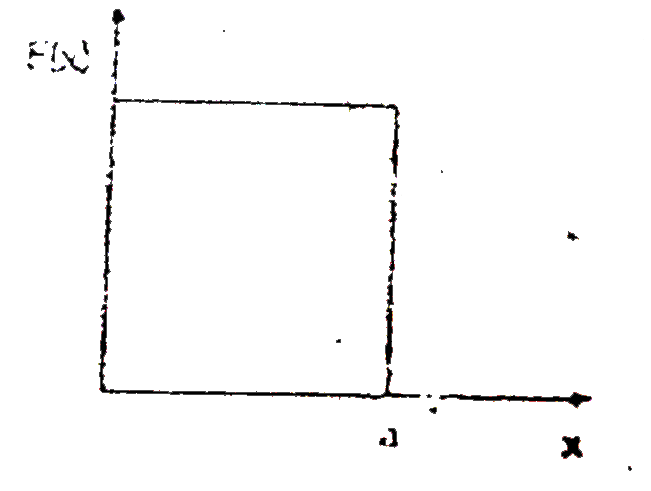
B
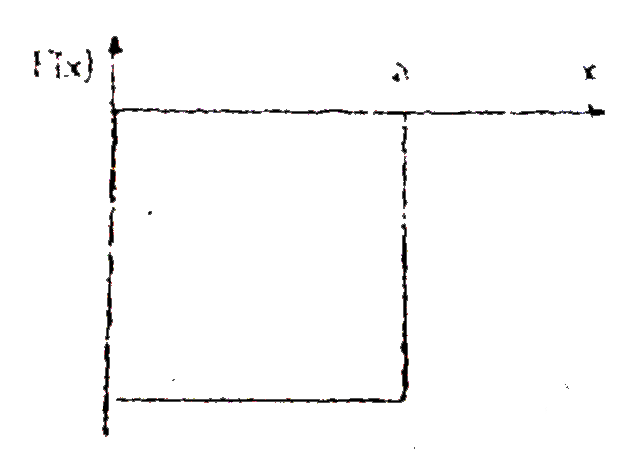
C
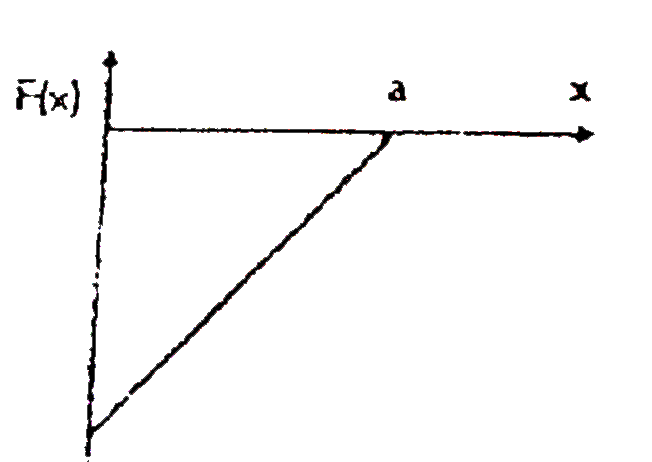
D
Video Solution
Text Solution
The correct Answer is:B
F(x)=−dU(x)dt=−slope of U(v)/(s)x curve
Intially slope is constant and then it becomes zero.
|
Topper's Solved these Questions
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
- TEST PAPER 1Book:ALLENChapter:TEST PAPER 1Exercise:PHYSICS
Explore 11 Videos
Similar Questions
Knowledge Check
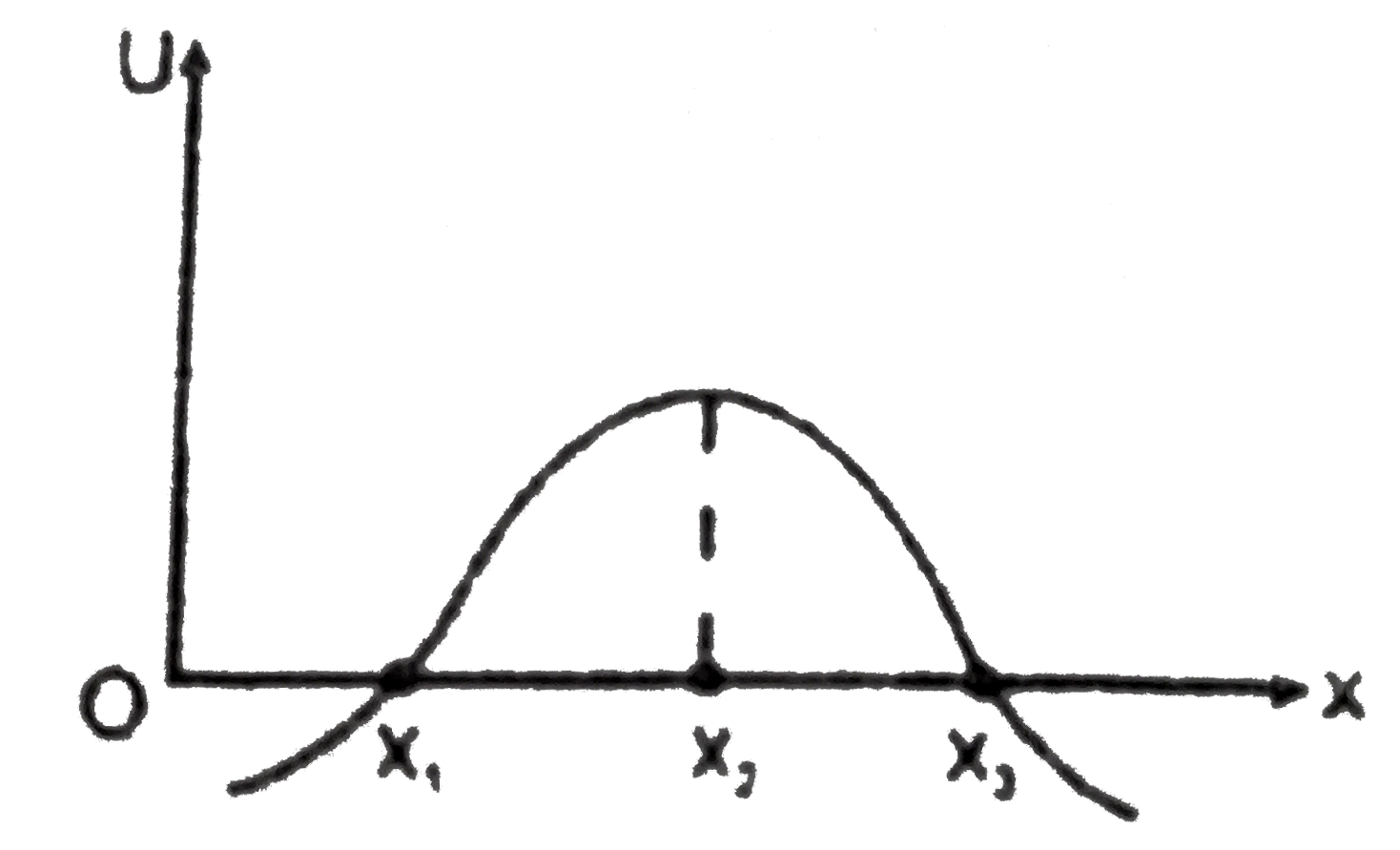
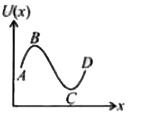
In the figure shown the potential energy U of a particle is plotted its position 'x' from origin. Then which of the following statement is correct A particle at :
 Ax1 is in stable equilibriumBx2 is in stable equilibriumCx3 is in stable equilibriumDnone of these
Ax1 is in stable equilibriumBx2 is in stable equilibriumCx3 is in stable equilibriumDnone of theseThe potential energy U(x) of a particle moving along x−axis is shown in figure. Which one of the following graphs represents the variation of kinetic energy of this particel if its total mechanical enery is E0
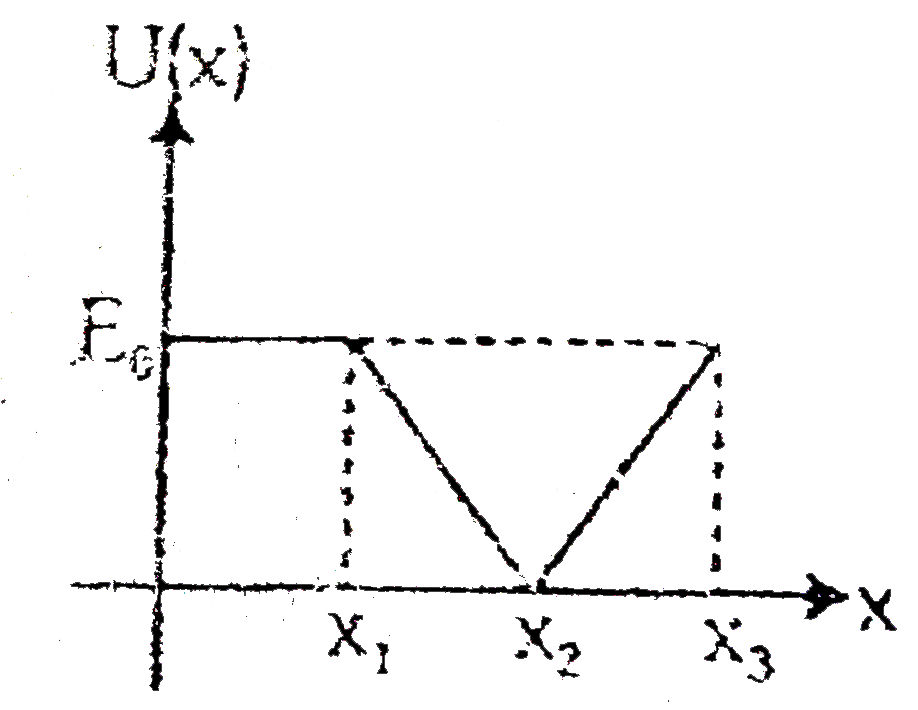 A
A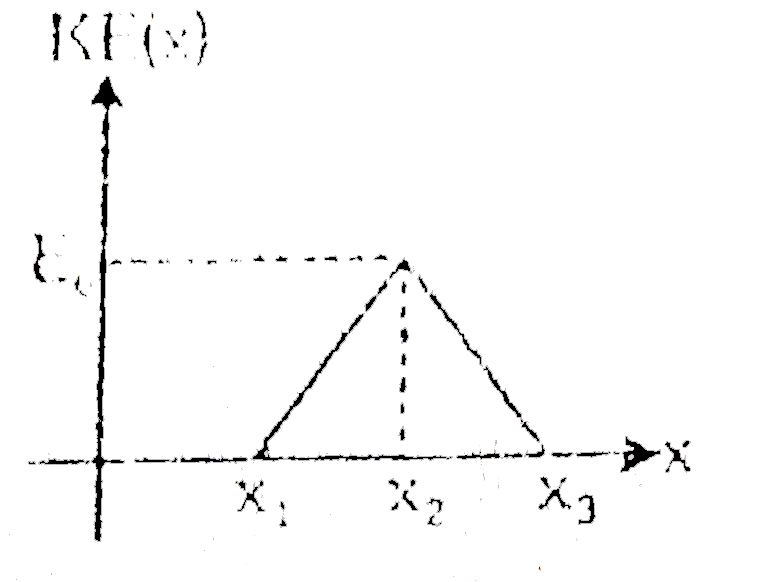 B
B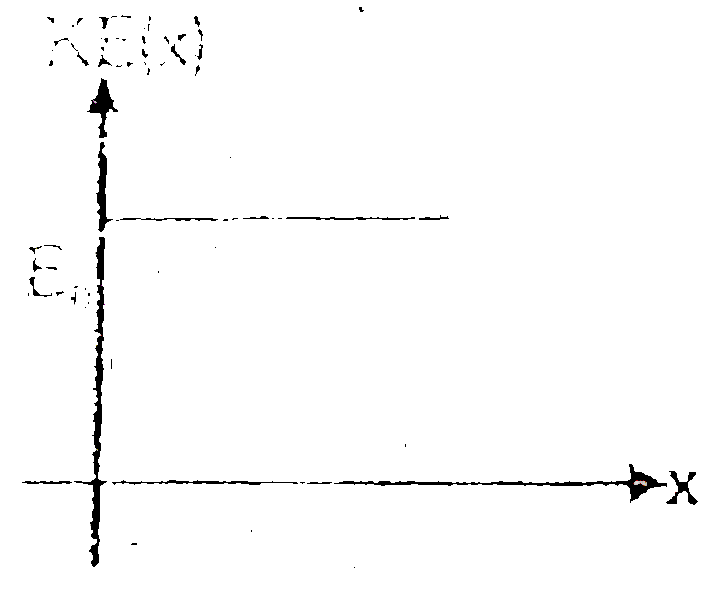 C
C D
D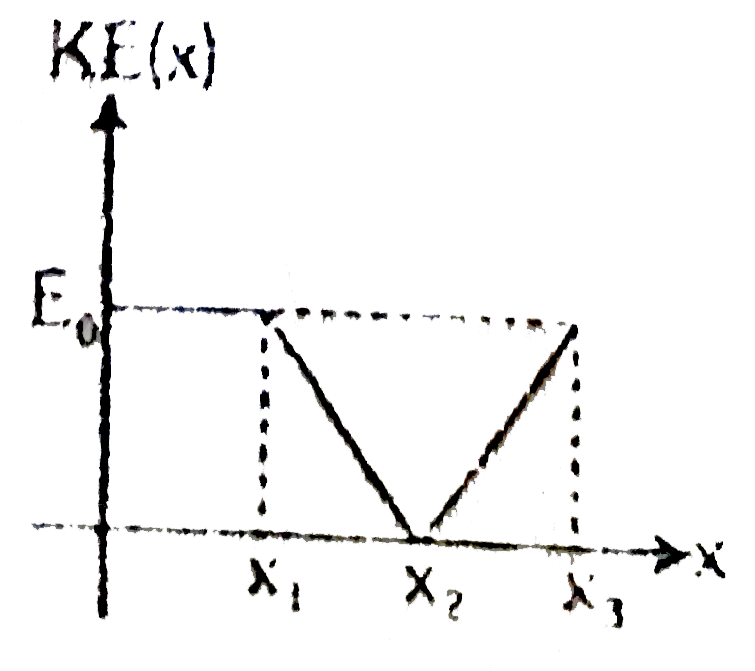
The potential energy U between two molecules as a function of the distance X between them has been shown in the figure. The two moleucles are
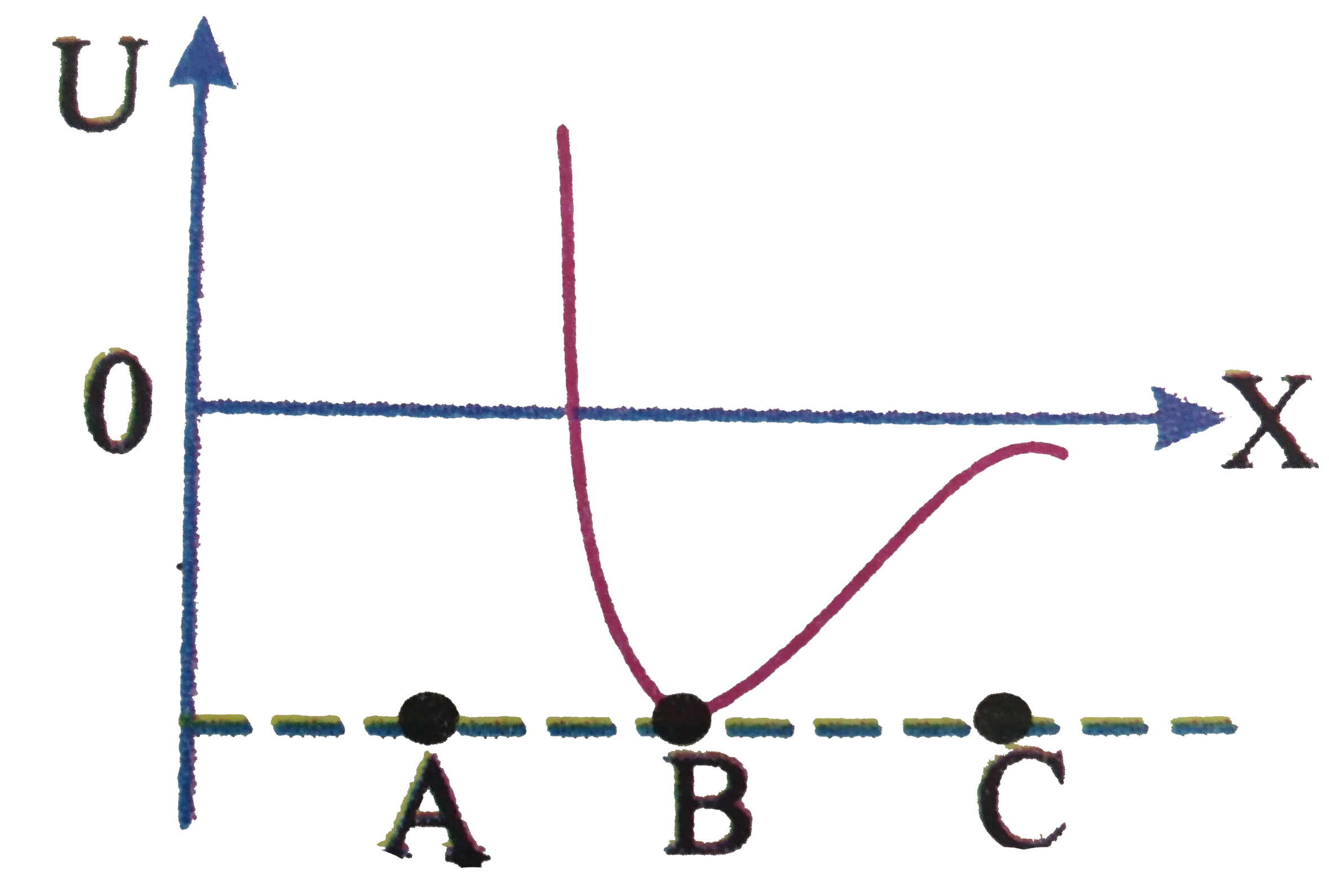 Aattracted when X lies between A and B and are replled when X lies between B and CBattracted when X lies between B and C and are repelled when X lies between A and BCattracted when they reach BDrepelled when they reach B
Aattracted when X lies between A and B and are replled when X lies between B and CBattracted when X lies between B and C and are repelled when X lies between A and BCattracted when they reach BDrepelled when they reach B
The graph of y=f(x) is as shown in the following figure. Draw the graph of y=[f(x)].
View SolutionThe potential energy U between two molecules as a function of the distance X between them has been shown in the figure. The two molecules are

View Solution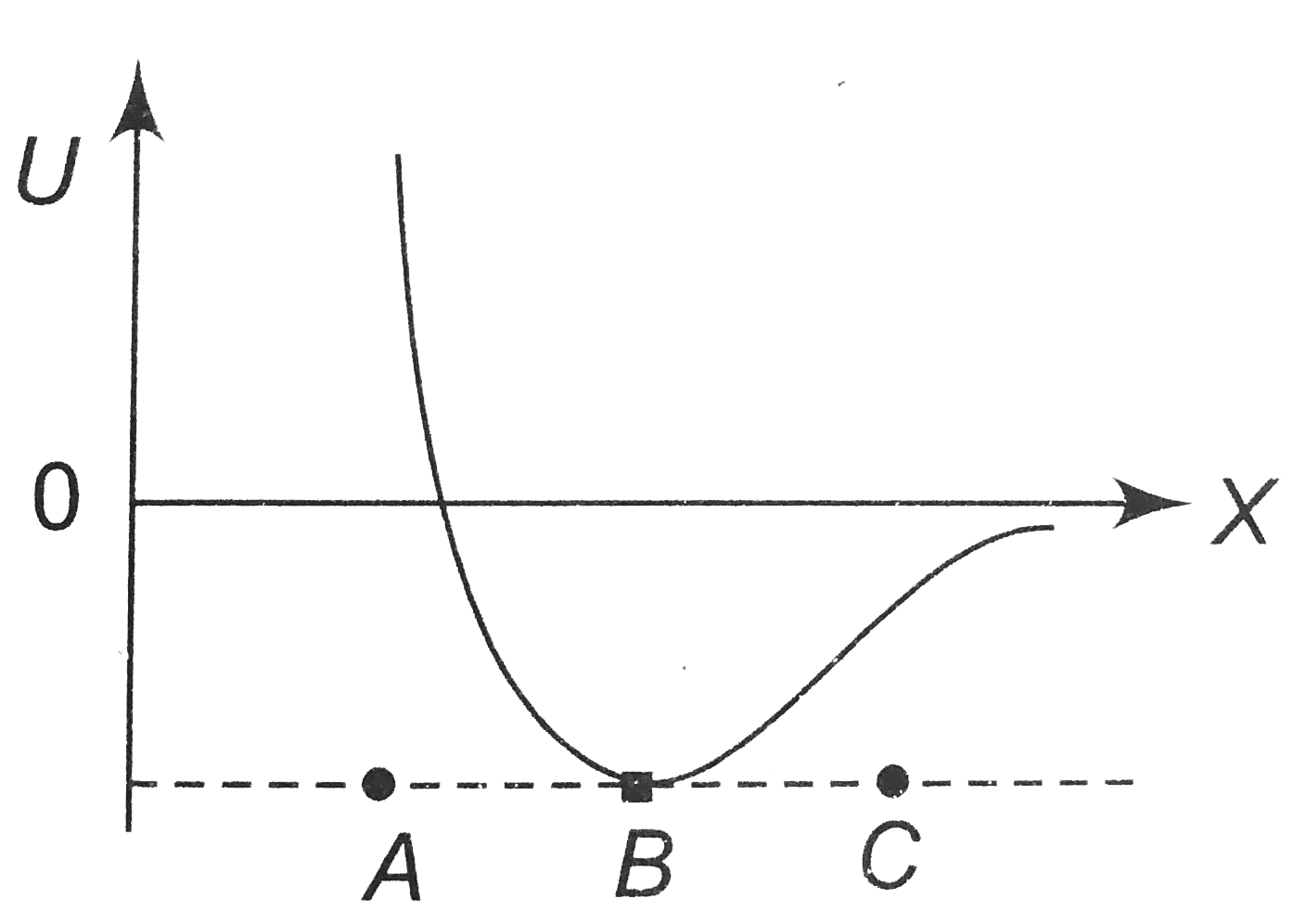
The potential energy U between two molecules as a function of the distance X between them has been shown in the figure. The two molecules are
View SolutionPotential energy of a particle at position x is given by U=x2−5x. Which of the following is equilibrium position of the particle?
View SolutionThe potential energy of a particle varies with distance x as shown in the graph. The force acting on the particle is zero at
View Solution