A particle of mass m is moving with constant speed in a vertical circle in X−Z plane. There is a small both at some distance on Z−axis. The maximum distance of the shadow of the particle on X−axis from origin equal to :−
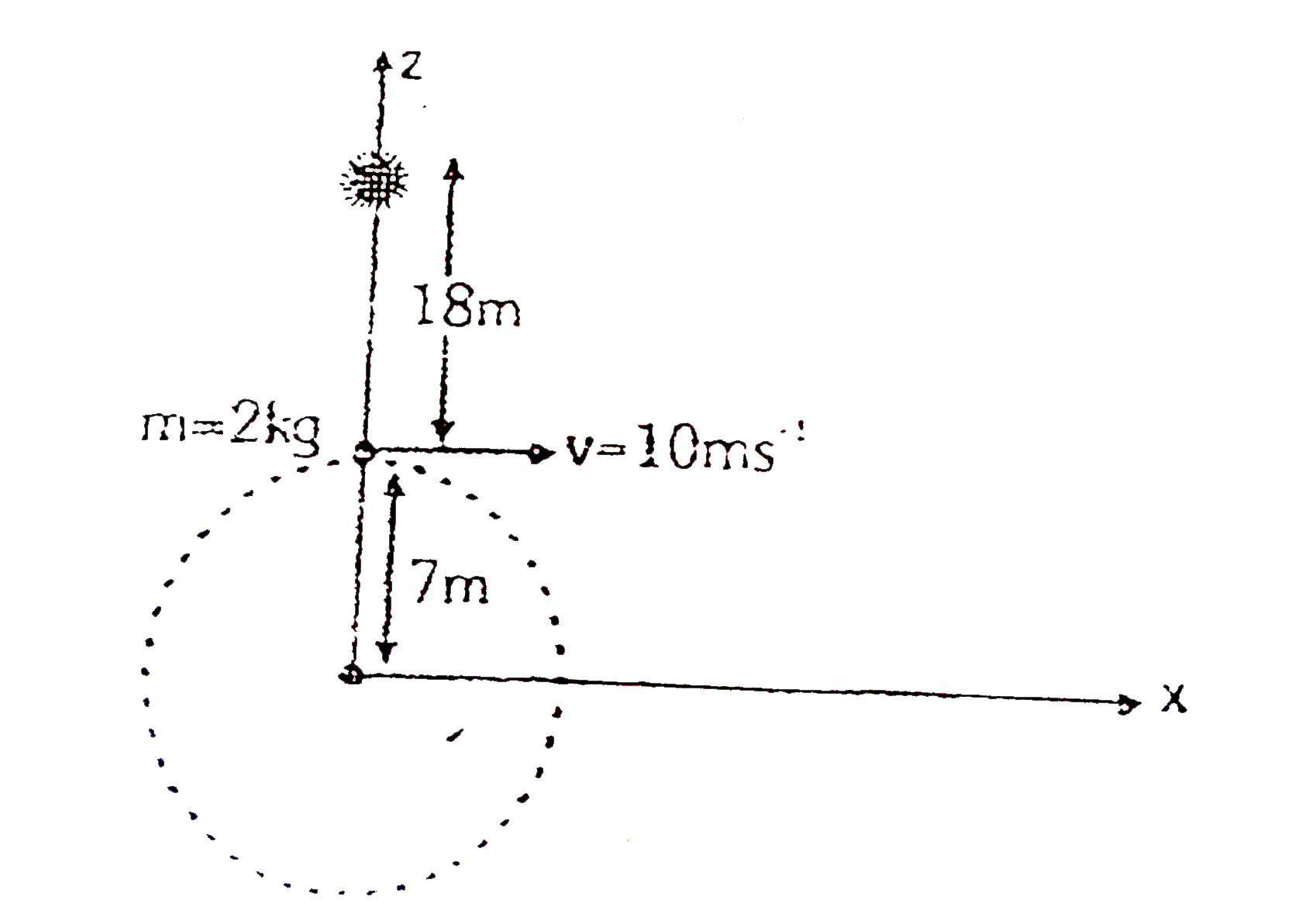
17524m
12524m
25m
24m
The correct Answer is:A
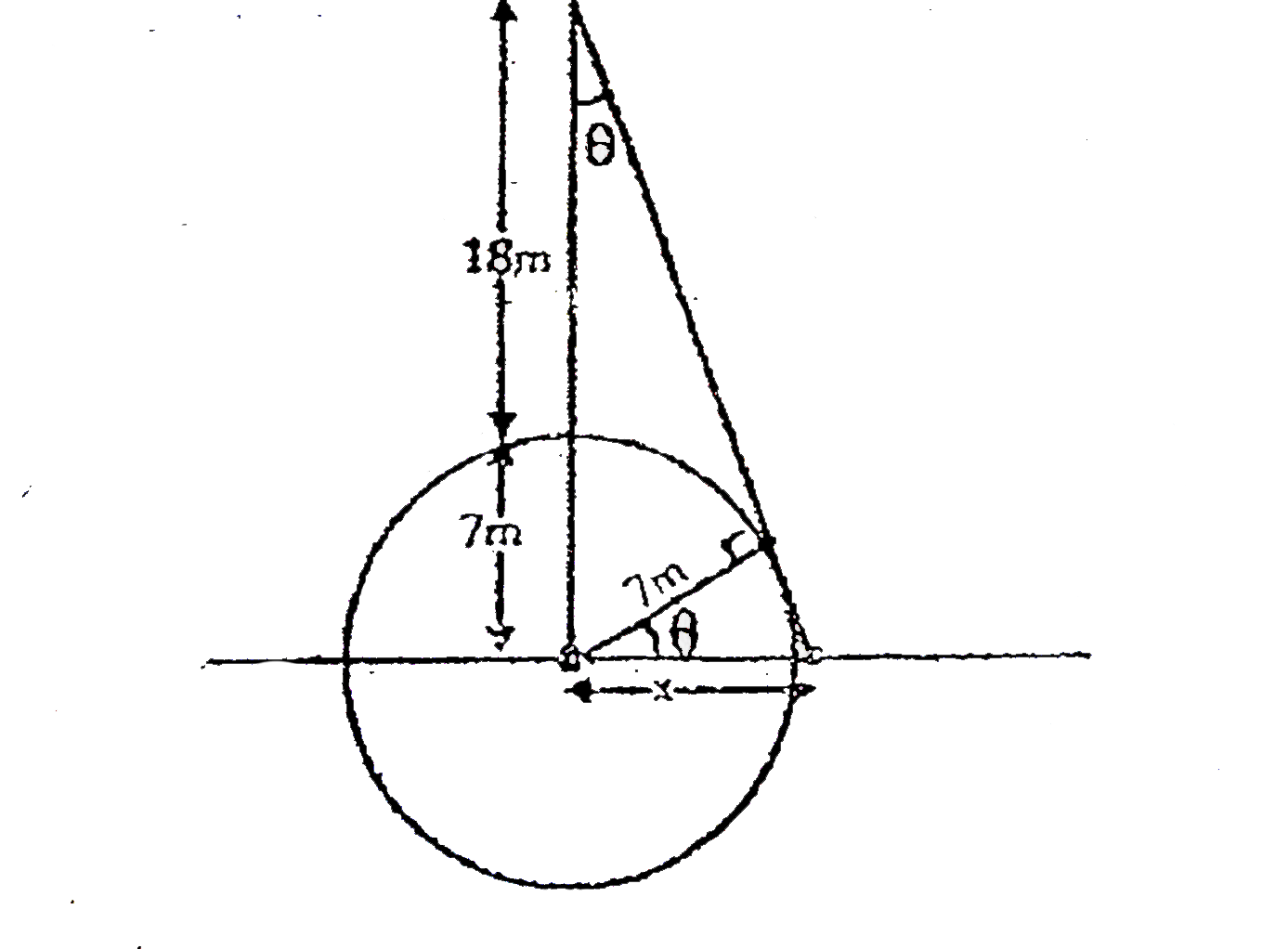
sinθ=725,cosθ=2425
xcosθ=7⇒x=7cosθ=7×2524=17524m
Topper's Solved these Questions
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
- TEST PAPER 1Book:ALLENChapter:TEST PAPER 1Exercise:PHYSICS
Explore 11 Videos
Similar Questions
A particle of mass m is moving with constant speed in a vertical circle in x−z plane. There is a small bulb at some distance on z -axis. The maximum distance of the shadow of the particle on x -axis is found to be α(2524). Find the value of α.
'(##CEN_KSR_PHY_JEE_C07_024_Q13##)'
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View Solution
Knowledge Check
If a particle moves with a constant speed, the distance-time graph is
Astraight lineBcircleCstairlike lineDpolygonIf a particle moves with a constant speed, the distance-time graph is
Astraight lineBcurved lineChorizontal line to time axisDparallel line to velocity axisA particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is
 AmvbBmvaCmv√a2+b2Dmv(a+b)
AmvbBmvaCmv√a2+b2Dmv(a+b)
A particle of mass m is moving with speed u. It is stopped by a force F in distance x if the stopping force is 4 F then
View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
View SolutionIn the previous question, if the pitch of the helical path is equal to the maximum distance of the particle from the x-axis
View SolutionIf a particle of mass m is moving with constant velocity v parallel to x-axis in x−y plane as shown in fig. Its angular moment with respect to origin at any time t will be
View SolutionDistance of plane x+y+z=3 from origin is
View Solution
