Two 20g worms climb over a 10cm high, very then wall. One worm is thin and 20cm long the other is fat and only 10cm long. What is the ratio of the potential energy (w.r.t. the base of wall) of the thin worm as comparses to that of the fat worm when each is half way over the top of the wall as shown?
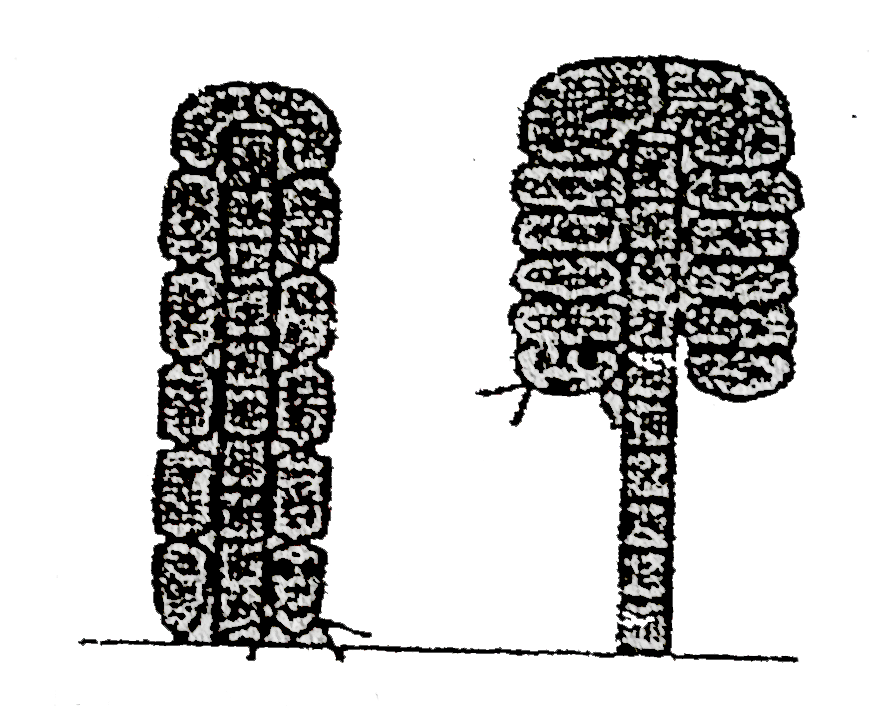
1:1
2:1
2:3
1:2
The correct Answer is:C
Ans.(3)
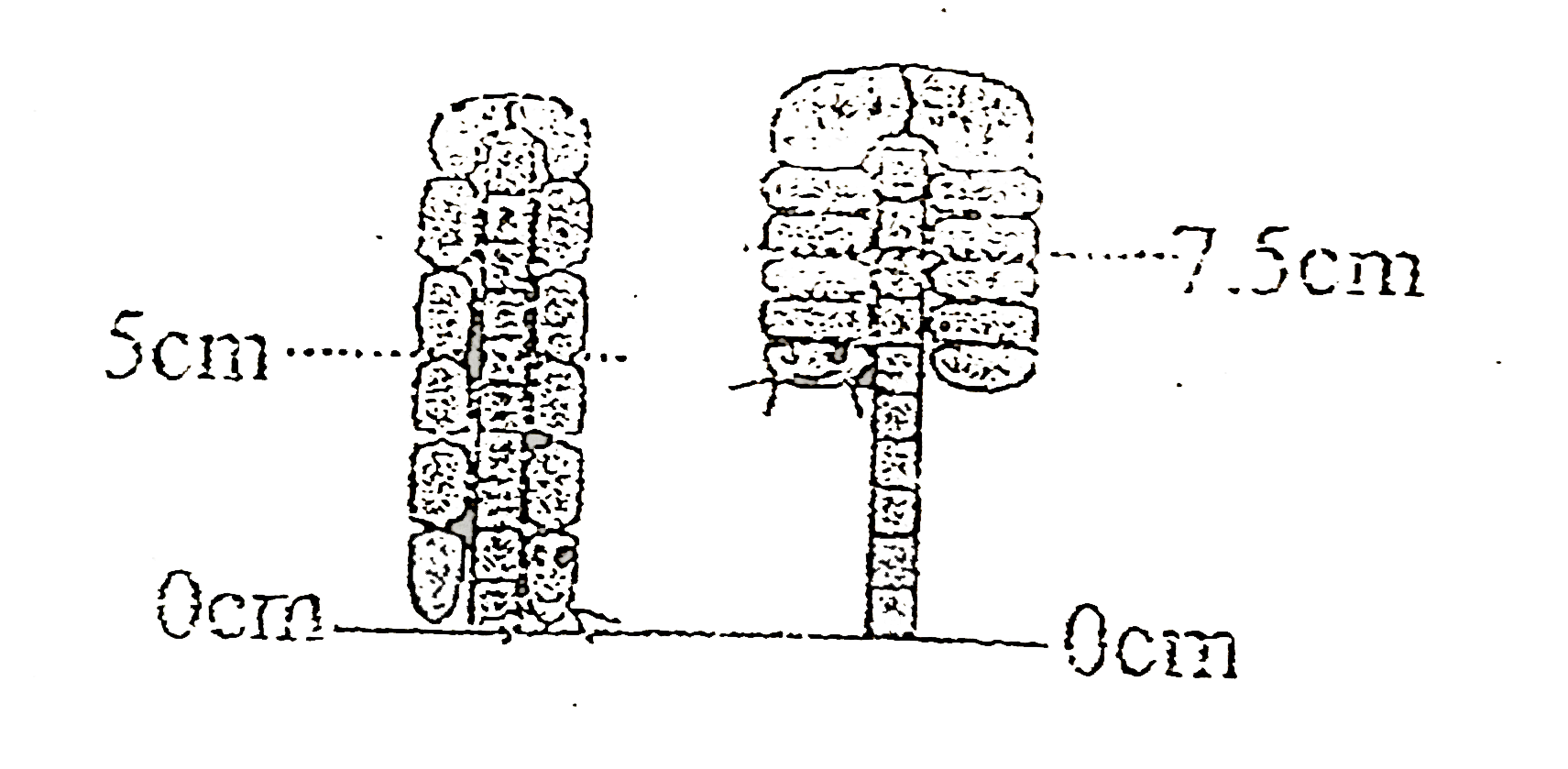
Required ratio=5cm7.5cm=23
Topper's Solved these Questions
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
- TEST PAPER 1Book:ALLENChapter:TEST PAPER 1Exercise:PHYSICS
Explore 11 Videos
Similar Questions
Two cylinder cans have bases of the same size. The diameter of each is 14cm. One of the canes is 10cm high and the other is 20cm high. Find the ratio of their volumes.
View SolutionTwo balls of the same radius and weight are suspended on threads so that their surface are in contact. A charge of q0=4×10−7C is given to the balls which makes them repel each other and diverge to an angle of 60°. Find the mass of the balls if the distance of balls from the point of suspension to the centre of ball is 20cm. Find the density of the material of the balls if the angle of divergence becomes 54° when the balls are immersed in kerosene of density 800kgm−3 dielectric constant ofkerosene is εr=2[1.592g,255K/gm3]
View Solution
Knowledge Check
Two 20g flatworms climb over a veruy thin walll, 10 cm high.One of the wrom is 20 cm long, the other is wider and only 10 cm long. Which of the following statement is correct reagarding them?
A20 cm wormk has done more work against gravityB10 cm worm has done more work against gravityCBoth worms have done equal work against gravityDratio of work donw by both the worms is 4:5Two cylinder cans have bases of the same size. The diameter of each is 14cm. One of the canes is 10cm high and the other is 20cm high. Find the ratio of their volumes.
A1:2B1:3C2:1DNone of these
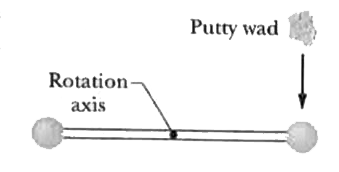
Two 2.00 kg balls are attached to the ends of a thin rod of length 50.0 cm and negligible mass. The rod is free to rotate in a vertical plane without friction about a horizontal axis through its center. With the rod initially horizontal (Fig. 10-87), a 50.0 g wad of wet putty drops onto one of the balls, hitting it with a speed of 3.00 m/s and then sticking to it. (a) What is the angular speed of the system just after the putty wad hits? (b) What is the ratio of the kinetic energy of the system after the collision to that of the putty wad just before? ( c) Through what angle will the system rotate before it momentarily stops?
View SolutionAladder of 5 m long rest with one end against a vertical wall of height 3 m and the other end on the lower ground. If its top slides down at the rate of 10 cm/sec, find the rate at which the foot of the ladder is sliding.
View SolutionSuppose you have to pile up uniform bricks to erect an inclined wall by offsetting each brick by a constant fraction ,1n , of a brick length l over the beneath. How many bricks can be piled by before the wall collapse ? How high a wall can erect if the size of each bricks is 7.5cm×12.5cm×25cm and the offset is only 110 of the length of each bricks? What is the total offset you get ?
[Hints: The vertical line through CG of the wall must pass through the base. The moment it goes beyond the base brick, the wall will collapse.]
View SolutionA thin pipe having outside diameter of 3 cm is to be covered with two layers of insulation each having thickness of 2.5 cm. The thermal conductivity of one material is five times that of the other. Assuming that the inner and outer surface temperatures of the composite wall are fixed, find the percentage reduction in heat transfer when the better insulating material is next to the thin pipe than when it is outside.
View SolutionTwo straight thin bars, one of brass and the other of steel are joined togethre side by side by short steel cross-pieces at 0∘C, one cm long the centre lines of the bars being one cm apart. When heated to 100∘C, the composite bar becomes bent into the arc of a circle. calculate the radius of this circle.
α for brass =19×10−6 per .∘C
and α for steel =11×10−6 per .∘C
View SolutionA large squre container with thin transparent vertical walls and filled with water ( refractive index 43) is kept on a horizontal table . A student holds a thin straight wire vertically inside the water 12cm from one of its corners, as shown schematically in the figure . Looking at the inside the water 12 cm from one of its corners, as shown schematically in the figure . Looking at the wire from this corner, another student sees two images of the wire , located symmetrically on each side of the line of sight as shown. THe separation (in cm) between these images is _______ .
View Solution