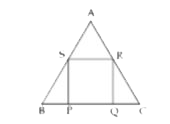
A uniform wire frame is in the shape of an equilateral triangle in plane with centroid at the orgin. Then

If is removed, the centre of mass of the remaining figure is in fourth quadrant.
If is removed, the centre of mass of the remaining figure is on the positive axis.
If is removed, the centre of mass of the remaining figure is in third quadrant.
All of above.
The correct Answer is:D

When is removed, frame is symmetric about axisCOM shifts towards i.e. on axis only.
When is removed frame is symmetric about shifts towards When AC is removed, frame is symmetric about COM shifts towards
Topper's Solved these Questions
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
- TEST PAPER 1Book:ALLENChapter:TEST PAPER 1Exercise:PHYSICS
Explore 11 Videos
Similar Questions
Knowledge Check
A wire is looped in the form of a circle of radius 21 cm. It is rebent in the shape of an equilateral triangle. Find the area of the triangle. (Take π=227)
A484cm2B484√3cm2C308cm2D308√3cm2A wire frame in the shape of an equilateral triangle is hinged at one vertes so that it can swing freely in a vertical plane, with the plane of the Δ always remaining vertical. The side of the frame is 1/√3m. The time period in secods of small oscillations of the frmae will be-
Aπ√2Bπ√2Cπ√6Dπ√5Find the ratio of the areas of an equilateral triangle ABC and square EFGC, if G is the centroid of the triangle ABC.
 A27:16B1:4C4:3√3D3√3:4
A27:16B1:4C4:3√3D3√3:4
A piece of wire 132 cm long is bent successively in the shape of an equilateral triangle, a square and a circle. Then area will be longest in shape of:
View SolutionConstruct an equilateral triangle ABC of side 6cm.
View SolutionA piece of wire is bent in the shape of an equilateral triangle each of whose sides measures8 cm. This wire is rebent to form a circular ring. What is the diameter of the ring?
View SolutionA wire of length 20m is to be cut into two pieces. One of the places will be bent into shape of a square and the other shape of an equilateral triangle. Where the wire should be cut so that the sum of the areas of the square and triangle is minimum?
View SolutionABC is an equilateral triangle and O is its circumcentre, then the ∠AOCis
View Solution