A conducting rod (OP) of length L rotates in form of a conical pendulum with an angular velocity $ω$ about a fixed vertical axis passing through its end O. There is a uni- form magnetic field B in vertically downward direction. The rod makes an angle $θ$ with the direction of the magnetic field. Calculate the emf induced across the ends of the rod. Which end is positive?

Video Solution

Text Solution

Verified by Experts

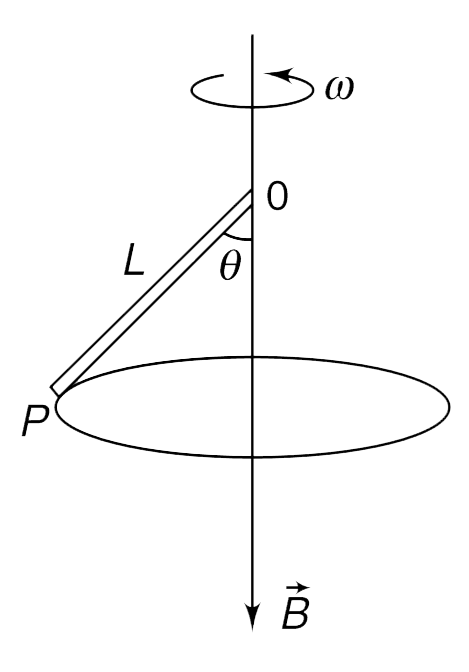
The correct Answer is: $\frac{1}{2} B ω L^{2} {sin}^{2} θ; O$ is positive

|

Answer

Step by step video, text & image solution for A conducting rod (OP) of length L rotates in form of a conical pendulum with an angular velocity omega about a fixed vertical axis passing through its end O. There is a uni- form magnetic field B in vertically downward direction. The rod makes an angle theta with the direction of the magnetic field. Calculate the emf induced across the ends of the rod. Which end is positive? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A uniform rod of mass $m$ and length $l$ rotates in a horizontal plane with an angular velocity $ω$ about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A $\frac{1}{2} m ω^{2} x$
B $\frac{1}{2} m ω^{2} (1 - \frac{x^{2}}{l})$
C $\frac{1}{2} m ω^{2} l (1 - \frac{x^{2}}{l^{2}})$
D $\frac{1}{2} m ω^{2} l [1 - \frac{x}{l}]$
Question 2 - Select One
A rod of length $L$ rotates in the form of a conical pendulum with an Angular velocity $ω$ about its axis as shown in Fig. 3.165. The rod makes an angle $θ$ with the axis. The magnitude of the motional emf developed across the two emf of the rod is

A(a) $\frac{1}{2} B ω L^{2}$
B(b) $\frac{1}{2} B ω L^{2} {tan}^{2} θ$
C( c) $\frac{1}{2} B ω L^{2} {cos}^{2} θ$
D(d) $\frac{1}{2} B ω L^{2} {sin}^{2} θ$
Question 3 - Select One or More
A thin uniform rod of mass $m$ and length $L$ rotates with the constant angular velocity $ω$ about .the vertical axis passing through the rod's suspensjon point $O$ . It describes a carried surface, then:
Acentrifugal force acting on rod is $m \frac{L}{2} sin θ ω^{2}$ and will pass through centre of mass
Bcentrifugal force acting on rod is $m \frac{L}{2} sin θ ω^{2}$ and will not pass through centre of mass
C $θ = {cos}^{- 1} (\frac{3 g}{2 ω^{2} L})$
D $θ = {cos}^{- 1} (\frac{g}{ω^{2} L})$

ARIHANT-ELECTROMAGNETIC INDUCTION-Level 2

Home
Ask Doubt
Profile