ABC is a triangular frame made of a conducting wire and is right angled at B. Its side BC is vertical and AB is horizontal. The frame is placed in a uniform magnetic field B in vertically upward direction. The frame is rotated about its side BC with constant angular speed ω. Resistance of the entire frame is R. Neglect gravity. Length AB=L,BC=2L.
(a) Find the torque needed to keep the frame rotating with constant angular speed.
(b) Find the potential difference between points A and C.
The correct Answer is:(a) Zero (b) 12BωL2
Step by step video, text & image solution for ABC is a triangular frame made of a conducting wire and is right angled at B. Its side BC is vertical and AB is horizontal. The frame is placed in a uniform magnetic field B in vertically upward direction. The frame is rotated about its side BC with constant angular speed omega. Resistance of the entire frame is R. Neglect gravity. Length AB = L, BC = 2L. (a) Find the torque needed to keep the frame rotating with constant angular speed. (b) Find the potential difference between points A and C. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - ARIHANTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2
39 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - ARIHANTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 3
12 Videos
- CURRENT ELECTRICITYBOOK - ARIHANTCHAPTER - CURRENT ELECTRICITYEXERCISE - Current Electricity
105 Videos
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 3
59 Videos
Similar Questions
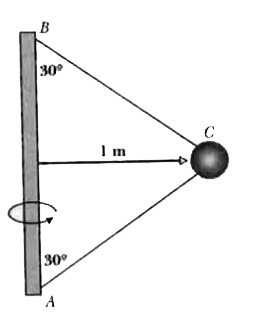
The mass at C is attached to the vertical pole AB by two wires. The assembly is rotating about AB at the constant angular speed ω. If the force in wire BC is twice the force in AC, determine the value of ω, see fig.
View SolutionIn △ABC right angled at B, if the two sides AB and BC are in the ratio 1 : 3, evaluate the value of sin C.
View Solution
Knowledge Check
A particle of mass m rotates with a uniform angular speed ω. It is viewed from a frame rotating about the Z-axis with a uniform angular speed ω0. The centrifugal force on the particler is
Amω2aBmω20aCm(ω+ω02)2aDmωω0aThe rigid conducting thin wire frame carries an electric current I and this frame is inside a uniform magnetic field →B as shown in Fig. 1.146. Then,
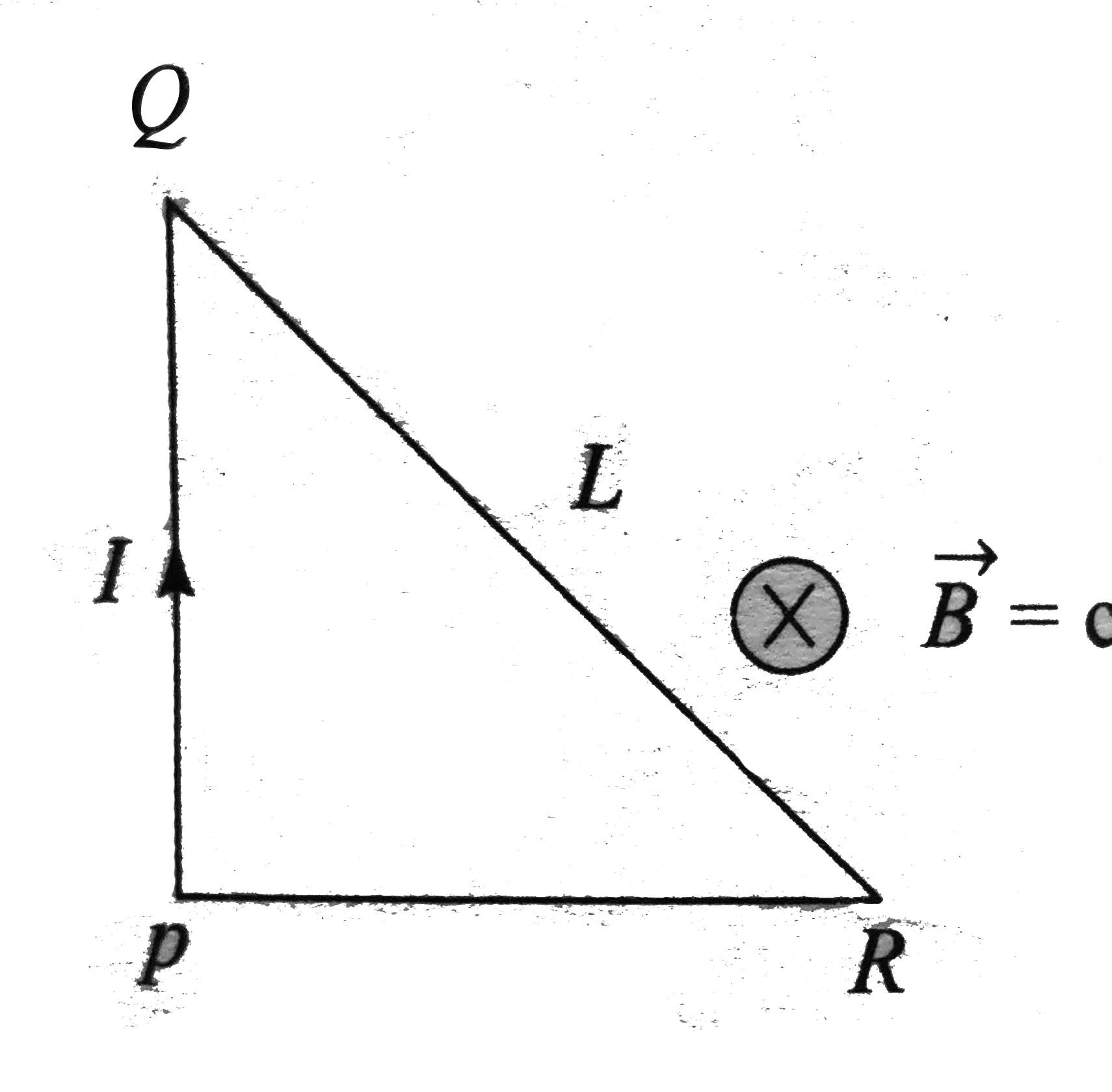 AThe net magnetic force on the frame is zero but the torque is not zeroBThe net magnetic force on the frame and the torque due to magnetic field are both zero.Cthe net magnetic force on the frame is not zero and the torque is also not zero.DNone of these
AThe net magnetic force on the frame is zero but the torque is not zeroBThe net magnetic force on the frame and the torque due to magnetic field are both zero.Cthe net magnetic force on the frame is not zero and the torque is also not zero.DNone of theseA conducting wire frame is placed in a magnetic field which is directed into the paper. The magnetic field is increasing at a constant rate. The direction of induced current in wire AB and CD are
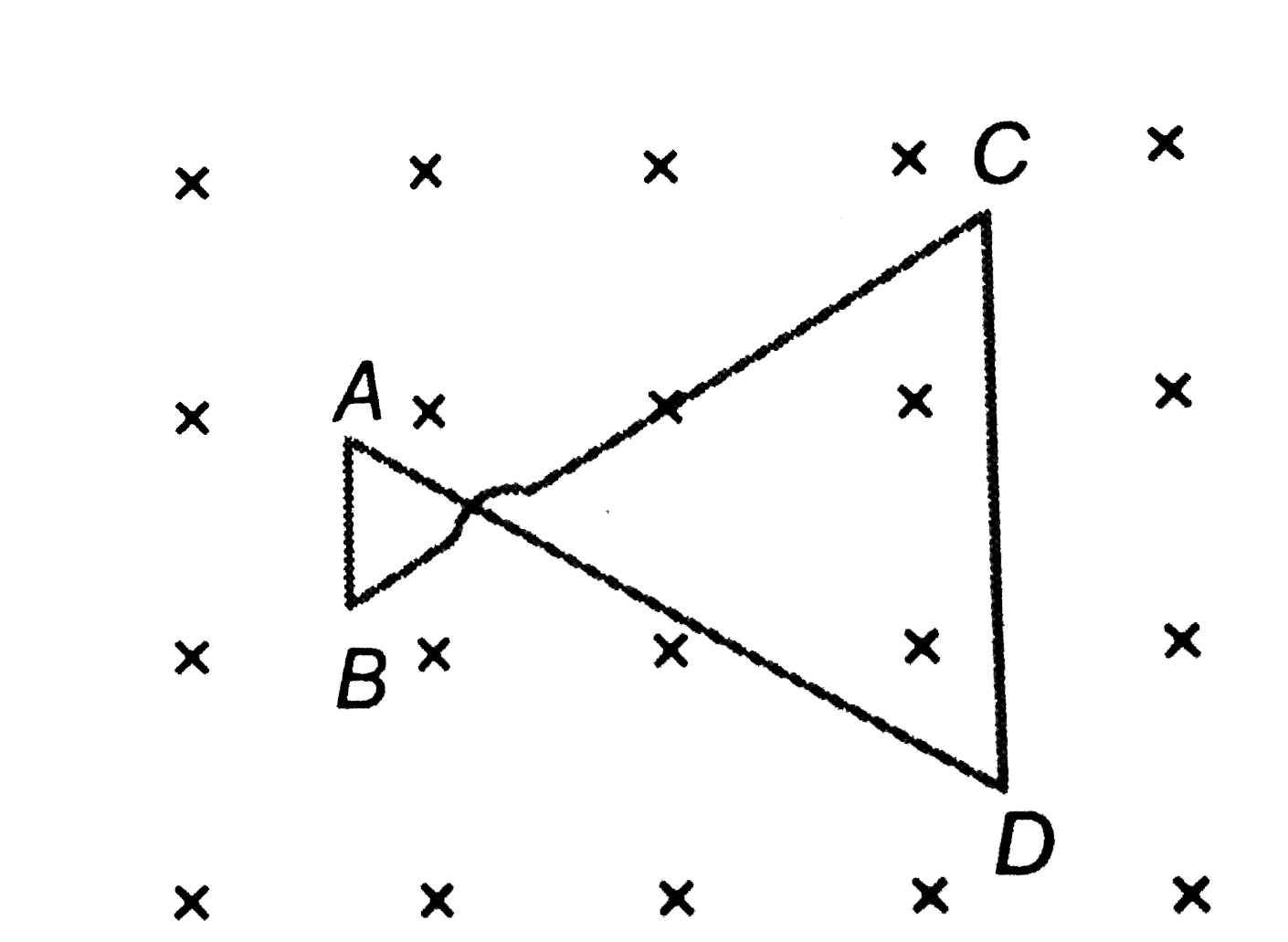 AB to A and D to CBA to B and C to DCA to B and D to CDB to A and C to D
AB to A and D to CBA to B and C to DCA to B and D to CDB to A and C to D
A horizontal wire is free to slide on the vertical rails of a conducting frame as shown in figure. The wire has a mass m and length l and the resistance of the circuit is R. If a uniform magnetic field B is directed perpendicular to the frame, the terminal speed of the wire as it falls under the force of gravity is

View SolutionABC is an equilateral triangular frame of mass m and side r. It is at rest under the action of horizontal magnetic field B (as shown) and the gravitational field.
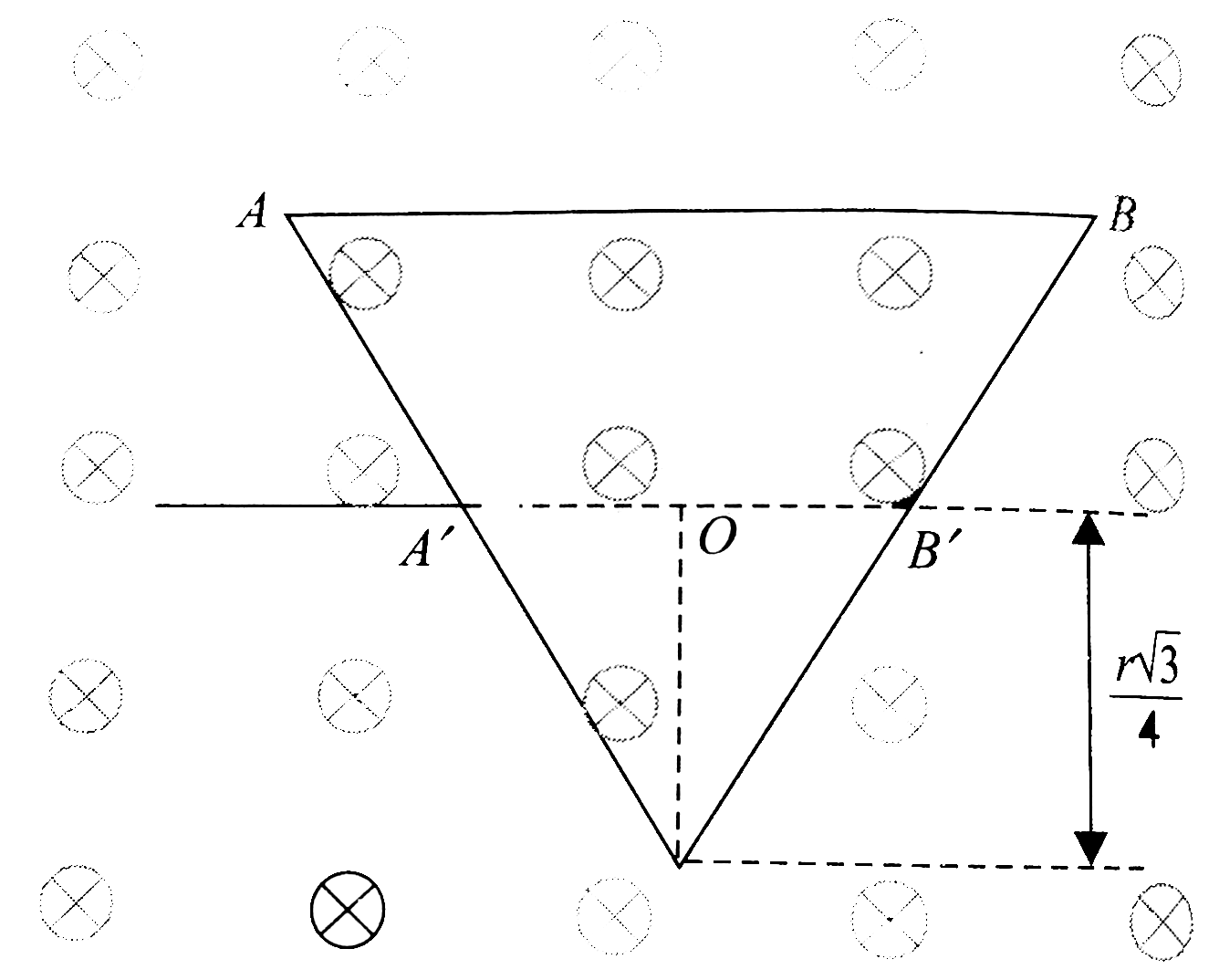
View SolutionA triangular field ABC, right angled at A has length AC=33m and AB=180m. The length of the side BC is
View SolutionSame current i=2 A is flowing in a wire frame as shown in the figure. The frame is a combination of two equilateral triangles ACD and CDE of side 1 m. It is placed in uniform magnetic field B=4 Tacting perpendicular to the plane of frame. The magnitude of magnetic force acting on the frame is

View SolutionA rectangular, horizontal conducting frame carries current, flowing clockwise as seen from above,. P is a point vertically above the centre of the frame. The direction of the magnetic field at P due to the current is
View Solution
