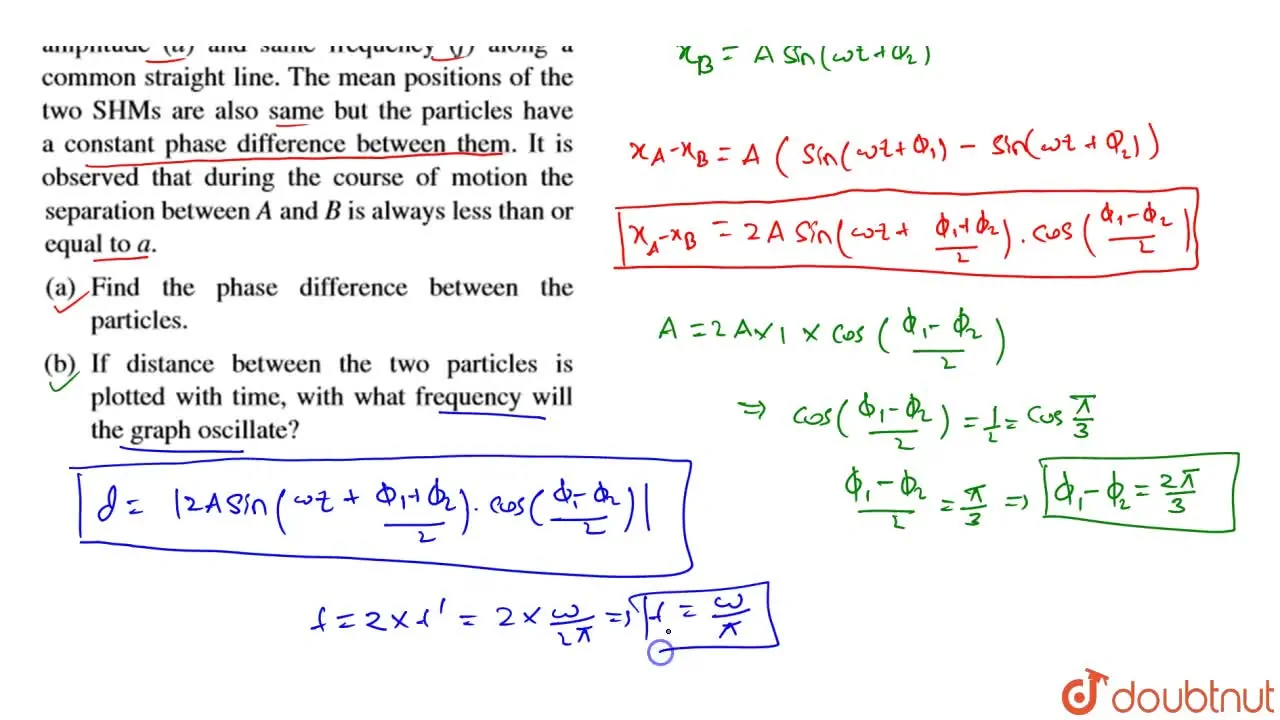
Two particles A and B are describing SHM of same amplitude (a) and same frequency (f) along a common straight line. The mean positions of the two SHMs are also same but the particles have a constant phase difference between them. It is observed that during the course of motion the separation between A and B is always less than or equal to a.
(a) Find the phase difference between the particles.
(b) If distance between the two particles is plotted with time, with what frequency will the graph oscillate?

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(a) ϕ_{1} - ϕ_{2} = \frac{2 π}{3} (b) 2 f$

Updated on:21/07/2023

Answer

Step by step video, text & image solution for Two particles A and B are describing SHM of same amplitude (a) and same frequency (f) along a common straight line. The mean positions of the two SHMs are also same but the particles have a constant phase difference between them. It is observed that during the course of motion the separation between A and B is always less than or equal to a. (a) Find the phase difference between the particles. (b) If distance between the two particles is plotted with time, with what frequency will the graph oscillate? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Knowledge Check

Two particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line. The maximum distance between the two particles is $a \sqrt{2}$ . The initial phase difference between the particle is –
Azero
B $π / 2$
C $π / 6$
D $π / 3$
Two particles P and Q describe S.H.M. of same amplitue 'A' and frequency n along the same straight line. The resultant displacement amplitude of the two S.H.M.s is $(A / \sqrt{2})$ . The initial phase difference between the two particles is nearly
A $π$
B $\frac{π}{2}$
C $\frac{π}{4}$
D $\frac{3 π}{4}$
Two particles $P$ and $Q$ describe $S . H . M .$ of same amplitude a, same frequency $f$ along the same straight line from the same mean position. The maximum distance between the two particles is a $\sqrt{2}$ , If the initial phase difference between the particles is $\frac{π}{N}$ then find $N$ :
Azero
B2
C6
D3

ARIHANT-SIMPLE HARMONIC MOTION-Level 2

Home
Ask Doubt
Profile

The correct Answer is:(a)ϕ1−ϕ2=2π3(b)2f

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line. The maximum distance between the two particles is a√2 . The initial phase difference between the particle is –

Two particles P and Q describe S.H.M. of same amplitue 'A' and frequency n along the same straight line. The resultant displacement amplitude of the two S.H.M.s is (A/√2). The initial phase difference between the two particles is nearly

Two particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line from the same mean position. The maximum distance between the two particles is a √2, If the initial phase difference between the particles is πN then find N :

ARIHANT-SIMPLE HARMONIC MOTION-Level 2

The correct Answer is: $(a) ϕ_{1} - ϕ_{2} = \frac{2 π}{3} (b) 2 f$

Two particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line. The maximum distance between the two particles is $a \sqrt{2}$ . The initial phase difference between the particle is –

Two particles P and Q describe S.H.M. of same amplitue 'A' and frequency n along the same straight line. The resultant displacement amplitude of the two S.H.M.s is $(A / \sqrt{2})$ . The initial phase difference between the two particles is nearly

Two particles $P$ and $Q$ describe $S . H . M .$ of same amplitude a, same frequency $f$ along the same straight line from the same mean position. The maximum distance between the two particles is a $\sqrt{2}$ , If the initial phase difference between the particles is $\frac{π}{N}$ then find $N$ :