A transverse harmonic wave of amplitude 4 mm and wavelength m is travelling in positive direction on a stretched string. At an instant, the particle at m is at mm and is travelling in positive direction. Find the co- ordinate of the nearest particle which is at its positive extreme at this instant.
The correct Answer is:2.25 m
To solve the problem step by step, we will follow these instructions:
Step 1: Understand the wave equation
The general equation for a transverse harmonic wave traveling in the positive x-direction can be expressed as:
y(x,t)=Asin(kx−ωt+ϕ)
where:
- A is the amplitude,
- k is the wave number,
- ω is the angular frequency,
- ϕ is the phase constant.
Step 2: Identify given values
From the problem, we have:
- Amplitude A=4mm=4×10−3m
- Wavelength λ=1.5m
- Position x=1.0m
- Displacement at x=1.0m is y=2mm=2×10−3m
Step 3: Calculate the wave number k
The wave number k is given by:
k=2πλ=2π1.5=4π3rad/m
Step 4: Set up the wave equation at t=0
At t=0, the wave equation simplifies to:
y(x)=Asin(kx+ϕ)
Substituting the known values:
2×10−3=4×10−3sin(4π3⋅1+ϕ)
Step 5: Solve for ϕ
Rearranging gives:
sin(4π3+ϕ)=12
The sine function equals 12 at angles π6+2nπ and 5π6+2nπ. Thus:
1. 4π3+ϕ=π6+2nπ
2. 4π3+ϕ=5π6+2nπ
Step 6: Solve for ϕ in both cases
For the first case:
ϕ=π6−4π3+2nπ
ϕ=π6−8π6+2nπ=−7π6+2nπ
For the second case:
ϕ=5π6−4π3+2nπ
ϕ=5π6−8π6+2nπ=−3π6+2nπ=−π2+2nπ
Step 7: Find the nearest particle at its positive extreme
The positive extreme occurs when y=A=4mm. We need to find the nearest x such that:
Asin(kx+ϕ)=4×10−3
Using the ϕ values calculated, we can find the corresponding x values.
Step 8: Calculate the position
We know that the maximum occurs at:
kx+ϕ=π2+2nπ
This gives:
x=π2−ϕk
Substituting k=4π3 and solving for x will yield the nearest particle's position.
Final Answer
After calculating, we find the nearest particle at its positive extreme is at:
x≈1.5m
The general equation for a transverse harmonic wave traveling in the positive x-direction can be expressed as:
y(x,t)=Asin(kx−ωt+ϕ)
where:
- A is the amplitude,
- k is the wave number,
- ω is the angular frequency,
- ϕ is the phase constant.
From the problem, we have:
- Amplitude A=4mm=4×10−3m
- Wavelength λ=1.5m
- Position x=1.0m
- Displacement at x=1.0m is y=2mm=2×10−3m
The wave number k is given by:
k=2πλ=2π1.5=4π3rad/m
At t=0, the wave equation simplifies to:
y(x)=Asin(kx+ϕ)
Substituting the known values:
2×10−3=4×10−3sin(4π3⋅1+ϕ)
Rearranging gives:
sin(4π3+ϕ)=12
The sine function equals 12 at angles π6+2nπ and 5π6+2nπ. Thus:
1. 4π3+ϕ=π6+2nπ
2. 4π3+ϕ=5π6+2nπ
For the first case:
ϕ=π6−4π3+2nπ
ϕ=π6−8π6+2nπ=−7π6+2nπ
ϕ=5π6−4π3+2nπ
ϕ=5π6−8π6+2nπ=−3π6+2nπ=−π2+2nπ
The positive extreme occurs when y=A=4mm. We need to find the nearest x such that:
Asin(kx+ϕ)=4×10−3
We know that the maximum occurs at:
kx+ϕ=π2+2nπ
This gives:
x=π2−ϕk
After calculating, we find the nearest particle at its positive extreme is at:
x≈1.5m
Topper's Solved these Questions
Similar Questions
A transverse wave of amplitude 2 m, wavelength 4 m and frequency 6 Hz is propagating in a string toward positive y-direction. The equation of the wave may be (y is in m and t is in s)
View SolutionA simple harmonic wave train of amplitude 1 cm and frequency 100 vibration is travelling along positive x-direction with a velocity ov 15ms−1. Calculate displacement y , the particle velocity and particle acceleration at x=180cm from the origin at t=5 s.
View Solution
Knowledge Check
A transverse wave, whose amplitude is 0.5 m wavelength is 1 m and frequency is 2Hz. is travelling along positive x direction. The equation of this wave will be-
Ay=0.5cos(2πx−4πt)By=0.5cos(2πx−4πt)Cy=0.5sin(πx−2πt)Dy=0.5cos(2πx−2πt)A transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is
Ay(x,t)=0.5sin(2πx−4πt)By(x,t)=0.5cos(2πx+4πt)Cy(x,t)=0.5sin(πx−2πt)Dy(x,t)=0.5cos(2πx+2πt)A transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is [
Ay(x,t)=0.5sin(2πx−4πt)By(x,t)=0.5cos(2πx+4πt)Cy(x,t)=0.5sin(πx+2πt)Dy(x,t)=0.5cos(2πx+2πt)
A simple harmonic wave of amplitude "2mm" is travelling along positive x-axis.At a given instant, displacement for two different particles on the wave is 1mm and √2mm along positive Y direction.If wave length of wave is 48mm then find minimum separation between positions of the particles (in mm)
View SolutionA sinusidal wave with amplitude ym=0.5mm and wavelength λ=0.4m. is travelling on a string with speed v=12 m/s. At t=0 particle at x=0.1 m is located at y=0.25 mm and going down.
(a) Find equation of wave.
(b) Plot the sinusoidal wave at t=0.
View SolutionA simple harmoinc progressive wave of amplitude 0.05 m and frequency 5Hz is travelling along the positive direction of x-axis with a speed of 40 m/s, then the displacement of a particle at 30 m from origin in the time 1 second will be
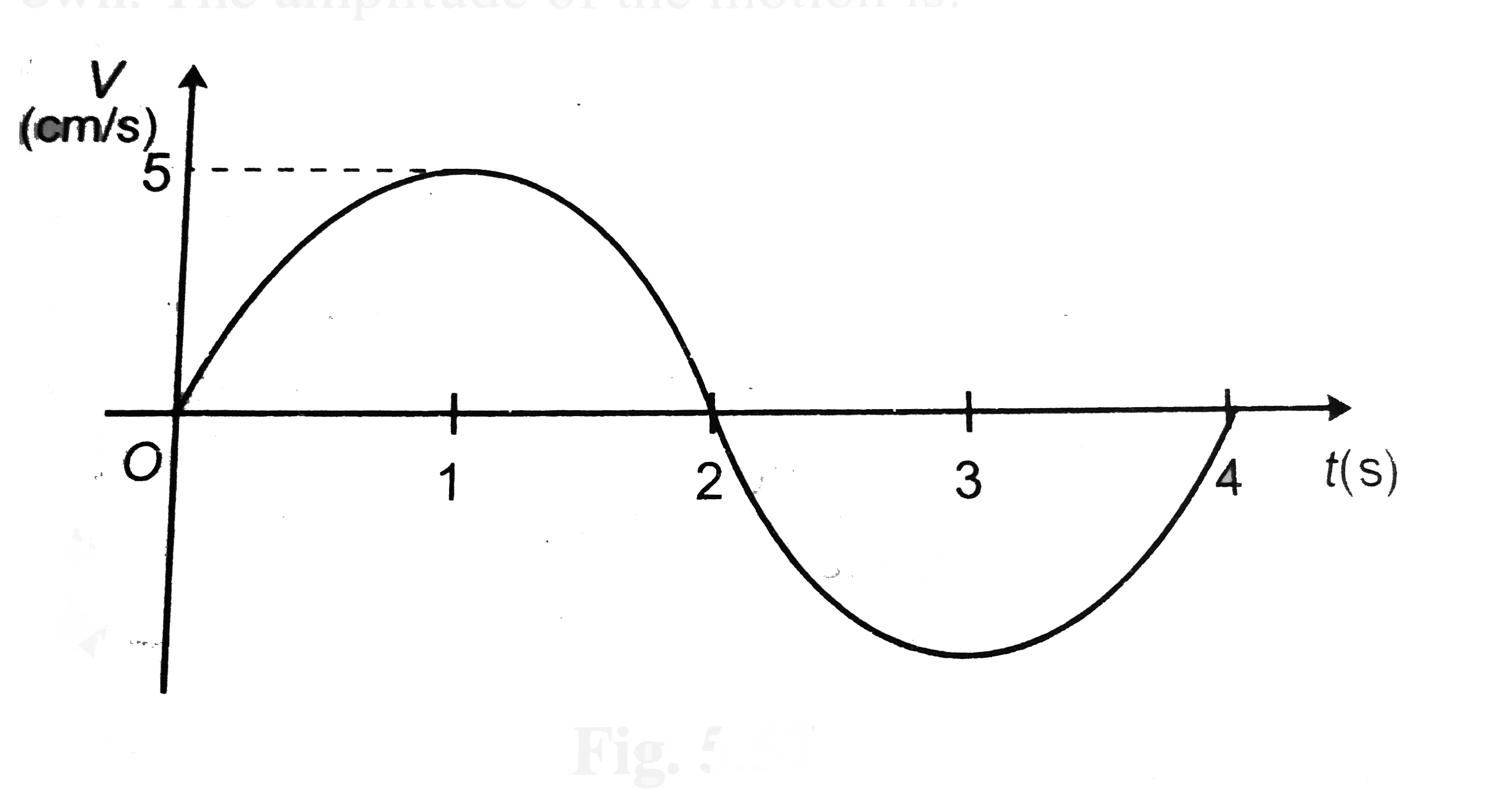
View SolutionA certain transverse sinusoidal wave of wavelength 20 cm is moving in the positive x direction. The transverse velocity of the particle at x=0 as a function of time is shown. The amplitude of the motion is:
View SolutionThe equation of a simple harmonic progressive wave having amplitude 5 mm, period 2 second and wavelength 2m and travelling along the positive direction of the x-axis is given by
View Solution