A long taut string is connected to a harmonic oscillator of frequency f at one end. The oscillator oscillates with an amplitude a0 and delivers power P0 to the string. Due to dissipation of energy the amplitude of wave goes on decreasing with distance x from the oscillator given as a=a0e–kx. In what length of the string (34)th of the energy supplied by the oscillator gets dissipated?
The correct Answer is:ln2k
Step by step video, text & image solution for A long taut string is connected to a harmonic oscillator of frequency f at one end. The oscillator oscillates with an amplitude a_(0) and delivers power P_(0) to the string. Due to dissipation of energy the amplitude of wave goes on decreasing with distance x from the oscillator given as a = a_(0)e^( –kx). In what length of the string (3/4)th of the energy supplied by the oscillator gets dissipated? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long taut string is plucked at its centre. The pulse travelling on it can be described as y(x,t)=e−(x+2t)2+e−(x−2t)2. Draw the shape of the string at time t=0, a short time after t=0 and a long time after t=0.
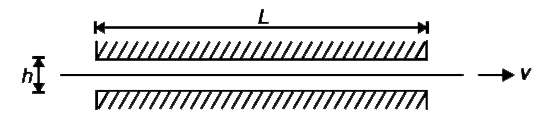
View SolutionDuring a painting process, a thin, flat tape of width b (dimension perpendicular to the plane of the figure) is pulled through a paint filled channel of length L. The density and viscosity of the paint liquid is ρ and η respectively. The tape is pulled at a constant speed v and width of the channel is h. Find the minimum force needed to pull the tape.
View Solution(i) The cause of increases in kinetic energy when a man starts running without his feet slipping on ground is asked to two students. Their answers are– Harshit: Cause of increase in kinetic energy is work done by friction force. Without friction the man cannot run. Akanksha: Cause of increase in kinetic energy is work done by internal (muscle) forces of the body. Who is right? (ii) An inextensible rope is hanging from a tree. A monkey, having mass m, climbs to a height h grabbing the rope tightly. The monkey starts from rest and ends up hanging motionlessly on the rope at height h. (a) How much work is done by gravity on the monkey? (b) How much work is done by the rope on the monkey? (c) Using work – energy theorem, explain the increase in mechanical energy of the monkey.
View SolutionA string is fixed at both end transverse oscillations with amplitude a0 are excited. Which of the following statements are correct ?
View SolutionA string of mass m is fixed at both ends. The fundamental tone oscillations are excited with circular frequency omega and maximum displacement amplitude a_(max) . Find : (a) the maximum kinetic energy of the string, (b) the mean kinetic energy of the string averaged over one oscillation period.
View SolutionA clamped string is oscillating in nth harmonic, then :-
View SolutionA string of mass per unit length μ is clamped at both ends such that one end of the string is at x = 0 and the other is at x=l. When string vibrates in fundamental mode, amplitude of the midpoint O of the string is a, tension in a, tension in the string is T and amplitude of vibration is A. Find the total oscillation energy stored in the string.
View SolutionA transverse harmonic wave is propagating along a taut string. Tension in the string is 50 N and its linear mass density is 0.02 kg m^( –1) The string is driven by a 80 Hz oscillator tied to one end oscillating with an amplitude of 1mm. The other end of the string is terminated so that all the wave energy is absorbed and there is no reflection (a) Calculate the power of the oscillator. (b) The tension in the string is quadrupled. What is new amplitude of the wave if the power of the oscillator remains same? (c) Calculate the average energy of the wave on a 1.0 m long segment of the string.
View SolutionIn damped oscillations, the amplitude is reduced to one-third of its initial value a_(0) at the end of 100 oscillations. When the oscillator completes 200 oscillations ,its amplitude must be
View SolutionThe total energy of simple harmonic oscillator is E and amplitude is 'A'. It the kinetic energy is 3E/4 without altering the amplitude. The displacement of the oscillator is
View SolutionIn damped oscillations, the amplitude of oscillation is reduced to one - third of its initial value a_(0) at the end of 100 oscillations. When the oscillator completes 200 oscillations, the amplitude must be
View SolutionIn damped oscillations, the amplitude of oscillation is reduced to one - third of its initial value a_(0) at the end of 100 oscillations. When the oscillator completes 200 oscillations, the amplitude must be
View SolutionIn damped oscillations, the amplitude of oscillations is reduced to one - third of its initial value a0 at the end of 100 oscillations. When the oscillator completes 200 oscillations, the amplitude must be
View SolutionIn damped oscillations, the amplitude is reduced to one-third of its initial value a_(0) at the end of 100 oscillations. When the oscillator completes 200 oscillations ,its amplitude must be
View SolutionA string is fixed at both end transverse oscillations with amplitude a0 are excited. Which of the following statements are correct ?
View Solution