An electromagnetic wave can be represented by E=Asin(kx−ωt+ϕ), where E is electric field associated with wave, According this equation, for any value of x,E remains sinusoidal for −∞<t<∞. Obviously this corresponds to an idealised situation because radiation from ordinary sources consists of finite size wavetrains. In general, electric field remains sinusoidal only for times of order τc' which is called coherence time. In simpler language it means that for times of order τc' a wave will have a definite phase. The finite value of coherence time could be due to many factors, for example if radiating atom undergoes collision with another atom then wave train undergoes an abrupt phase change or due to the fact that an atom responsible for emitting radiation has a finite life time in the energy level from which it drops to lower energy level, while radiating. Concept of coherence time can be easily understood using young's double slit experiment. Let interference patten is observed around point P at time t, due to superposition of waves emanting from S1 and S2 at times t=r1c and r2c respectively, where r1 and r2 are the distancesS1P&S2P. Obviously if r2−r1c<<τe,{where c=3×108m/s} then, wavetrain arriving at point P from S1 & S2 will have a definite phase relationship and an interference pattern of good contranst will be obtained.
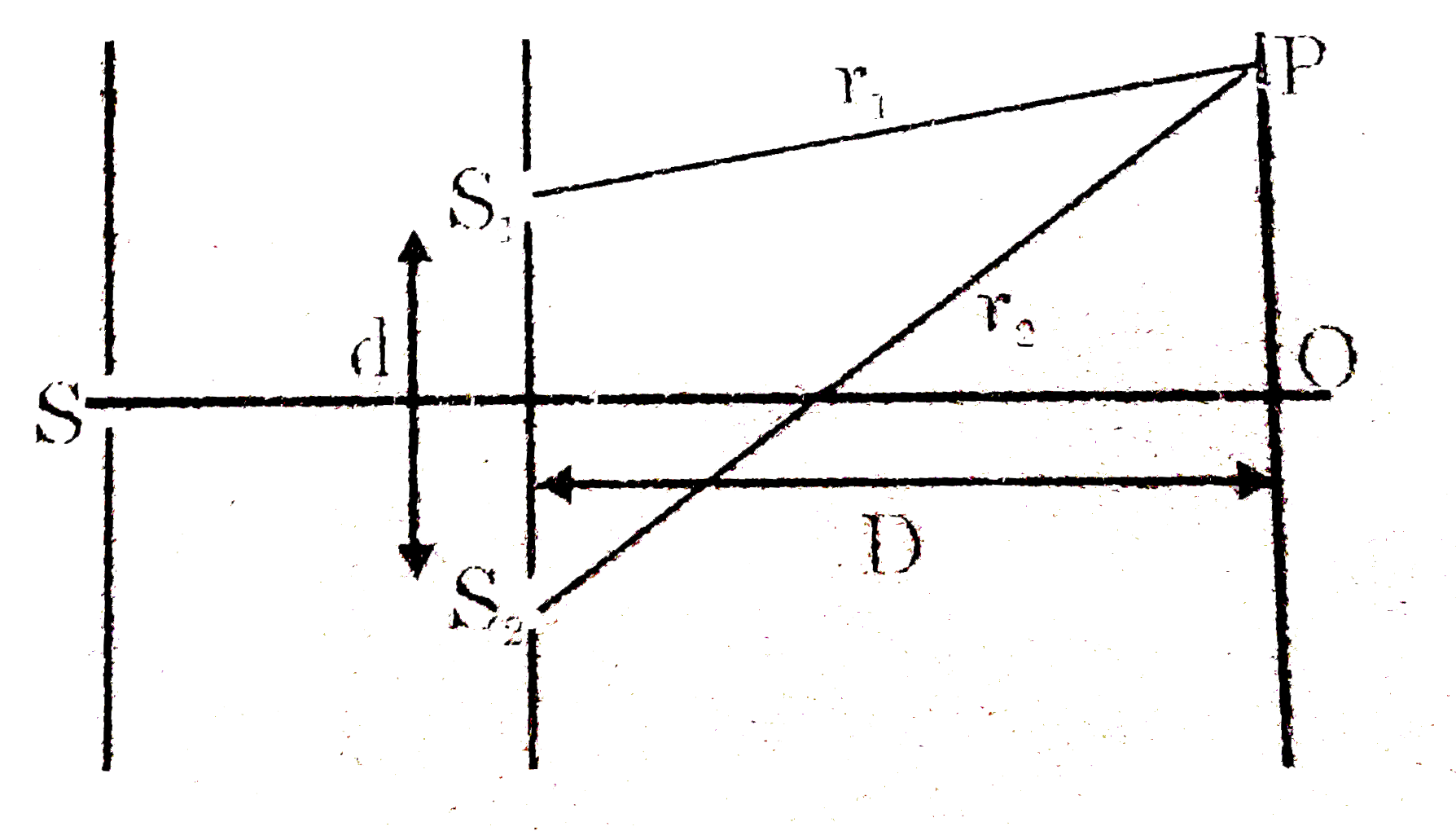
If coherence time is of order 10−10 second and screen is placed at a very large distance from slits in the given figure, then:-
For d=0.3cm, we will not get any fringers on screen (except central maxima)
For d=0.03cm, we will get fringes on a large part of screen
For d=3cm we will get fringes on entire screen.
We will get fringes on entire screen, irrespective of value of d.
The correct Answer is:B
dsinθ=(10−10)(3×108)=3×10−2m=3cm
(A) for d=0.3cm, we will get fringes on entire screen
(B) For d=0.03cm, we will get fringes on entire screen
(C) For d=3cm,θ=45∘
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 physics
63 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 physic
48 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - PAPER 3
100 Videos
- TEST PAPER 4BOOK - ALLENCHAPTER - TEST PAPER 4EXERCISE - PHYSICS
45 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
Knowledge Check
An electromagnetic wave going through vacuum is described by
E= E_0 sin(kx- omega t). Which of the following is/are independent of the wavelength?AkBωCkωDkωAn electromagnetic wave going through vacuum is described by
E=E0sin(kx−ωt),B=B0sin(kx−ωt).
ThenA(E0)k=(B0)ωB(E0)(B0)=ωkC(E0)ω=B0kDnone of these .In an electromagnetic wave, electric field E and magnetic field B are
AMutually perpendicular to each otherBbisect to each otherCboth (A) and (B)Dnone of these
Two sinusoidal waves are superposed. Their equations are
y1=Asin(kx−ωt+π6)andy2=Asin(kx−ωt−π6)
the equation of their resultant is
View SolutionIn a wave motion y=sin(kx−ωt),y can represent :-
View SolutionTwo waves at a point are represented by E1=E0sinωtandE2=E0sin(ω+ϕ). There will eb destructive interference at this point is
View SolutionIn an electromagnetic wave in free space the root mean square value of the electric field is Erms=6V/m. The peak value of the magnetic field is:
View SolutionIn an electromagnetic wave in free space the root mean square value of the electric field is Erms=6V/m. The peak value of the magnetic field is
View Solution
