An uniform electric field E exists along positive x-axis. The work done in moving a charge 0.5 C through a distance 2 m along a direction making an angle 60∘ with x-axis is 10 J. Then the magnitude of electric field is
5Vm−1
2Vm–1
√5Vm−1
20Vm–1
To find the magnitude of the electric field E, we can use the work done in moving a charge in an electric field. The work done W when a charge Q is moved through a distance d in an electric field is given by the formula:
W=Q⋅E⋅d⋅cos(θ)
Where:
- W is the work done (in joules),
- Q is the charge (in coulombs),
- E is the electric field (in volts per meter or newtons per coulomb),
- d is the distance moved (in meters),
- θ is the angle between the direction of the electric field and the direction of the displacement.
1. Identify the given values:
- Charge Q=0.5C
- Distance d=2m
- Angle θ=60∘
- Work done W=10J
2. Substitute the known values into the work formula:
W=Q⋅E⋅d⋅cos(θ)
Plugging in the values:
10=0.5⋅E⋅2⋅cos(60∘)
3. Calculate cos(60∘):
cos(60∘)=12
4. Substitute cos(60∘) into the equation:
10=0.5⋅E⋅2⋅12
5. Simplify the equation:
10=0.5⋅E⋅1
10=0.5E
6. Solve for E:
E=100.5=20V/m
Final Answer
The magnitude of the electric field E is 20V/m or 20N/C.
- W is the work done (in joules),
- Q is the charge (in coulombs),
- E is the electric field (in volts per meter or newtons per coulomb),
- d is the distance moved (in meters),
- θ is the angle between the direction of the electric field and the direction of the displacement.
- Charge Q=0.5C
- Distance d=2m
- Angle θ=60∘
- Work done W=10J
W=Q⋅E⋅d⋅cos(θ)
Plugging in the values:
10=0.5⋅E⋅2⋅cos(60∘)
cos(60∘)=12
10=0.5⋅E⋅2⋅12
10=0.5⋅E⋅1
10=0.5E
E=100.5=20V/m
The magnitude of the electric field E is 20V/m or 20N/C.
Topper's Solved these Questions
Similar Questions
There is and electric field E in the +x direction. If the work done by the electric field in moving a charges 0.2C through a distance of 2m along a line making an angle of 60∘ with the x-axis is 1.0J, what is the value of E in NC−1?
View SolutionThere is an electric field E in x-direction. If the work done on moving a charge of 0.2C through a distance of 2w m along a line making a angle 60∘ with x-axis is 4 J, then what is the value of E?
View Solution
Knowledge Check
There is an electric field E in x-direction. If the work done on moving a charge of 0.2C through a distance of 2w m along a line making a angle 60∘ with x-axis is 4 J, then what is the value of E?
A√3N/CB4N/CC5N/CD20N/CThere is an electric field E in x-direction. If the work done on moving a charge of 0.2C through a distance of 2w m along a line making a angle 60∘ with x-axis is 4 J, then what is the value of E?
A√3N/CB4N/CC5N/CD20N/CThree is an electric field E in the x - direction. If the work done by the electric field in moving a charge of 0.2C through a distance of 2m along a line making an angle 60∘ with the x- axis is 4J, then what is the value of E ?
A√3NC−1BNC−1CNC−1D20NC−1
What is the work done in moving a test charge q through a distance of 1 cm along the equatorial axis of an electric dipole ?
View SolutionWhat is the work done in moving a test charge q through a distance of 1 cm along the equatorial axis of an electric dipole ?
View SolutionA uniform electric field E exists between two oppositely charged plates (Fig. 3.38). What will be the work done in moving a charge q along a closed rectangular path ?
 .
.
View SolutionA uniform electric field E axis between two charged plates as shown in Fig. What would be work done in moving a charge q along the closed recetangualr path ABCDA ?
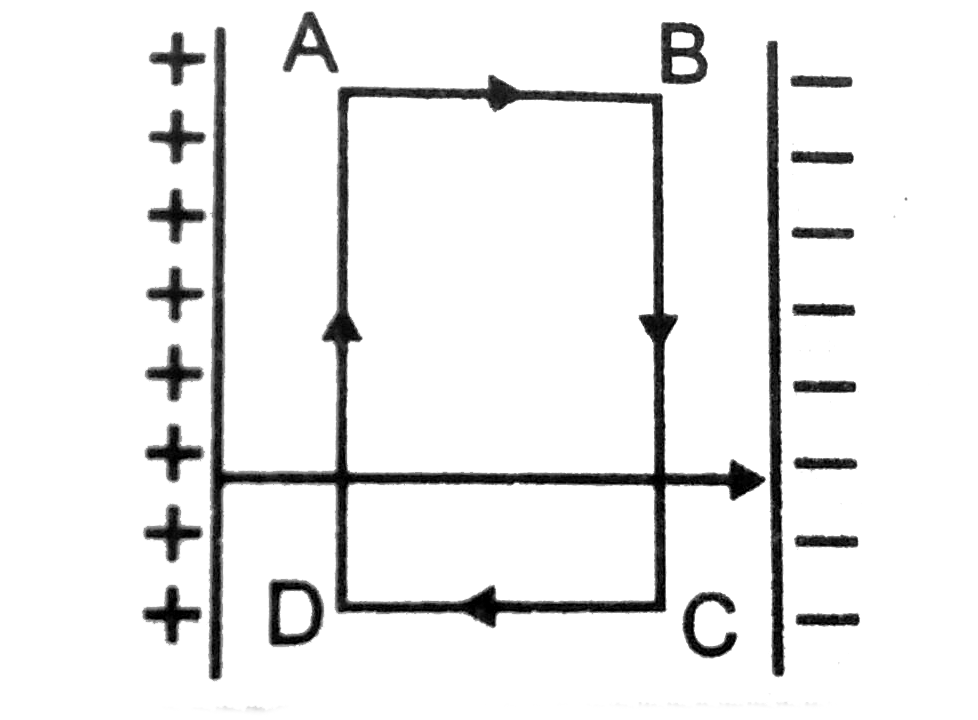
View SolutionA uniform electric field E=2×103NC−1 is acting along the positive x-axis. The flux of this field through a square of 10 cm on a side whose plane is parallel to the yz plane is
View Solution