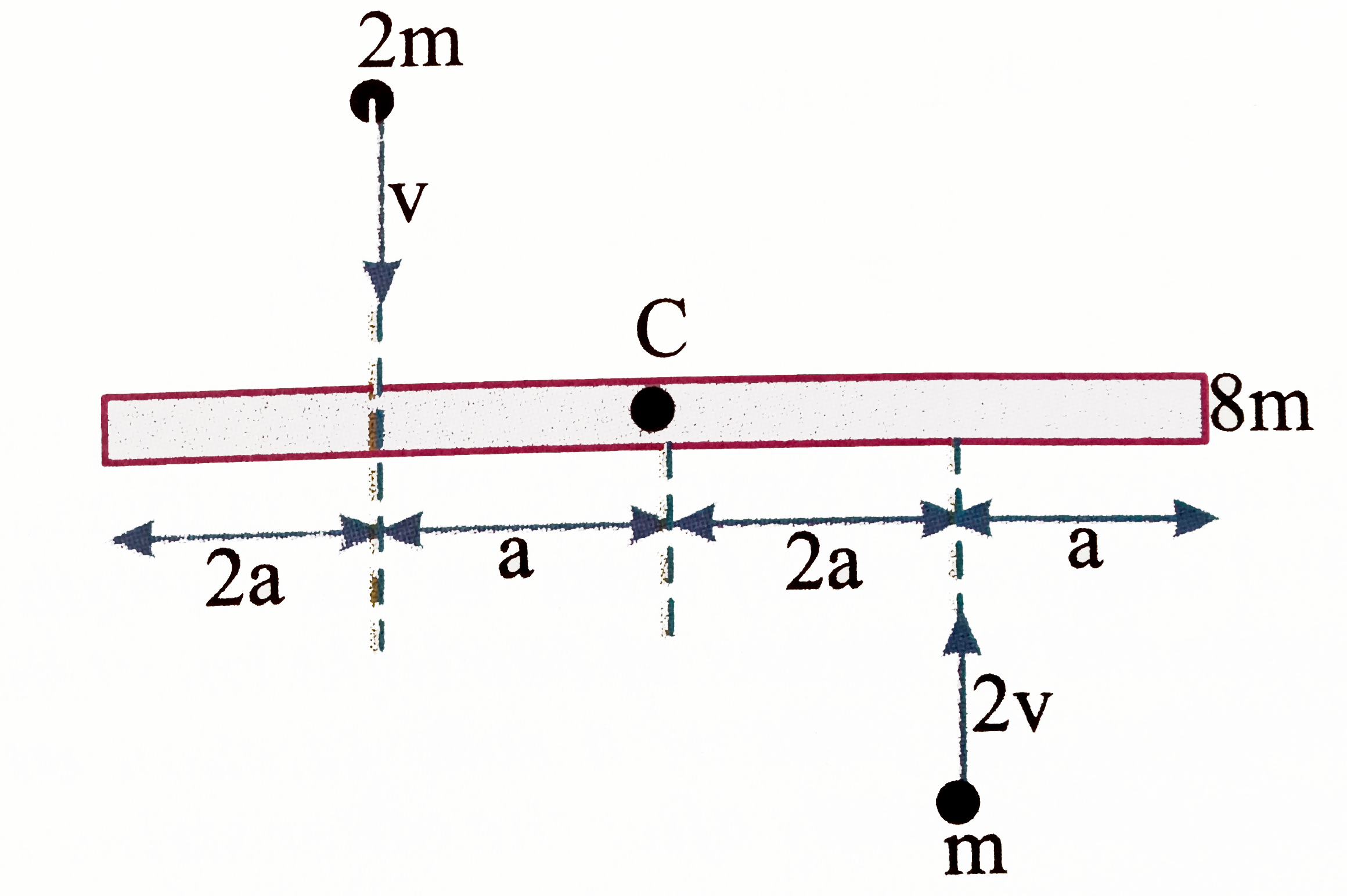
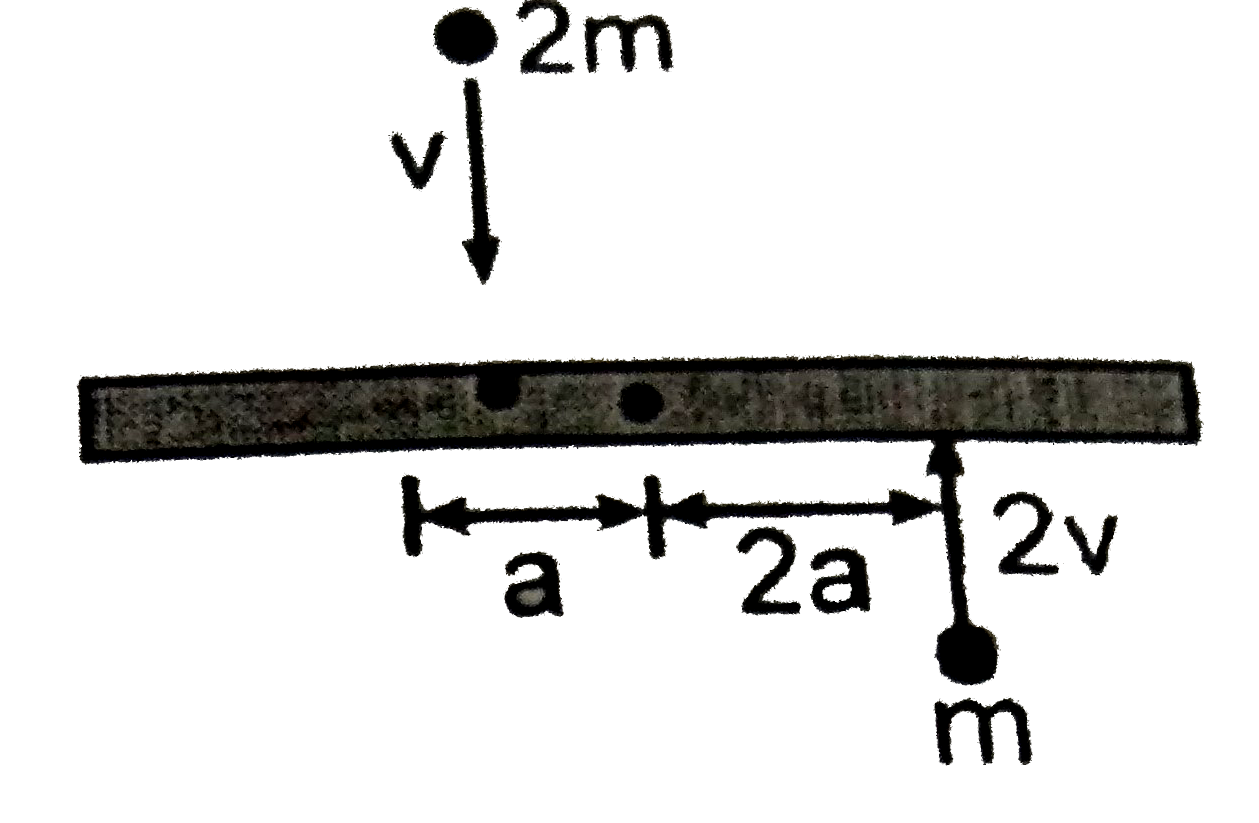
A uniform bar of length 6a and mass 8m lies on a smooth horizontal table. Two point masses m and 2m moving in the same horizontal plane with speeds 2v and v respectively, strike the bar (as shown in figure) and stick to the bar after collision. Calculate (a) velocity of the centre of mass (b) angular velocity about centre of mass and (c) total kinetic energy, just after collision.
35mv2
325mv2
315mv2
3mv2
The correct Answer is:A
(i) Cons. Linear momentum
−2m.v+2v.m=0=MVcm
Vcm=0
(ii) As ball sticks to Red
Conserving angular momentum about C
2v.m2a+2ma=Iω
=(8m.36a212+2m.a2+m4a2)
6mv.a=30ma2.ω
⇒ω=v5a
(iii)KE=12Iω2=12.30ma2×v225a2
=3mv25
Similar Questions
A uniform rod of length lambda lies on a smooth horizontal table A particle moving on the table has a mass m and a speed v before the collision and it sticks to the rod after the collision. The rod has a mass M then find out. The velocity of the centre of mass C and the angular velocity of the system about the centre of mass after the collision.
View SolutionA uniform rod of length 6a and mass 8m is placed on a smooth horizontal table. Two point masses of mass m and 2m moving with velocities 2v and v respectively collide with the bar as shown in the figure and after rotation get stuck to the rod. If the angular velocity about the center of mass, the velocity of the center of mass and the total energy E are respectively omega, v_(C) and E, then after the collision
View SolutionTwo particles A and B of mass m each hit the ends of a rigid bar of mass M and length l inelastically from opposite sides the speeds v and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar+particle).

View SolutionA uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
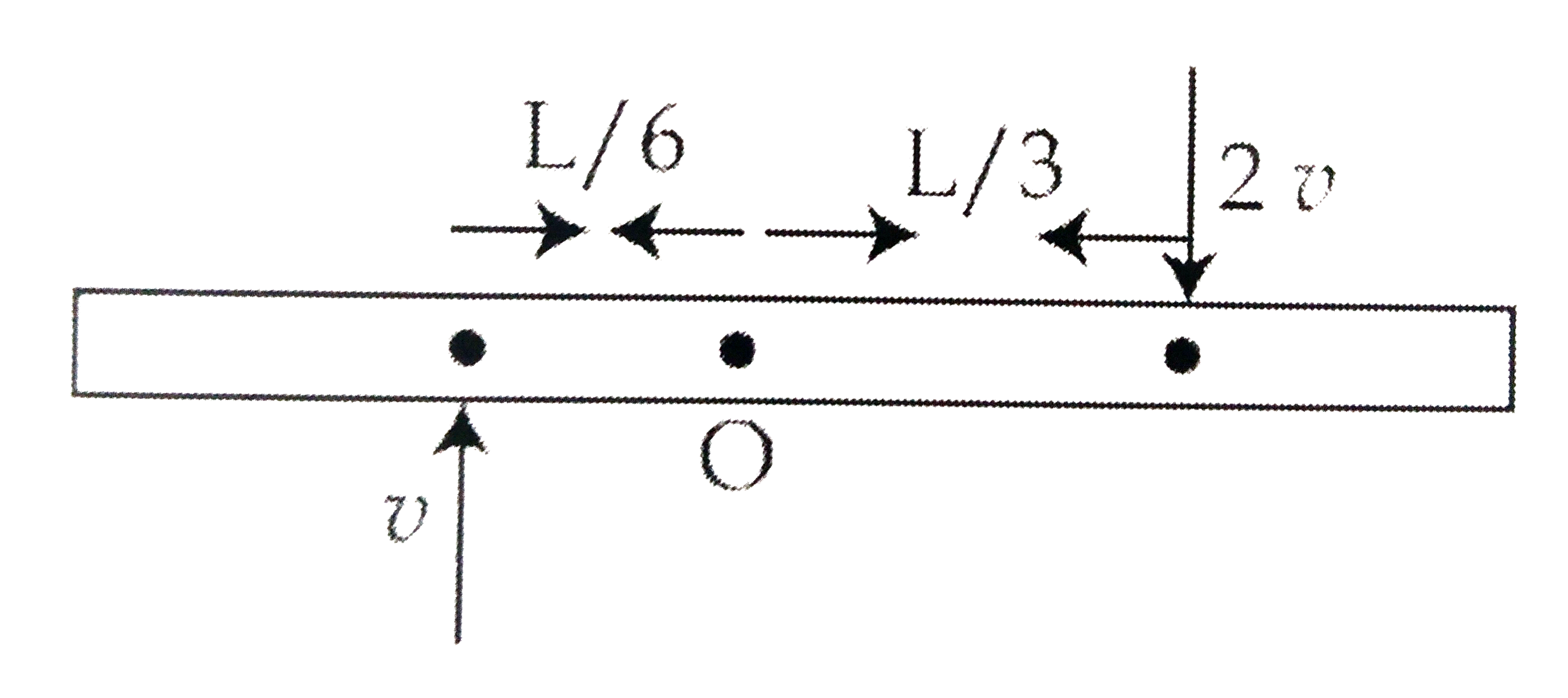
View SolutionA thin uniform bar of length L and mass 8m lies on a smooth horizontal table .Two point masses m and 2 m are moving in the same horizontal plane from opposite sides of the bar with speeds 2v and v respectively .The masses stick to the bar after collision at a distances L3andL6 respectively from the center of the bar if the bar starts rotating about its center of mass as result of collision the angular speed of the bar will be
View SolutionA uniform bar of length 6a and mass 8m lies on a smooth horizontal table. Two point masses m and 2m moving with the same horizontal speed 2v and v respectively, strike the bar as shown in the figure and stick to the bar after collision. Denoting angular velocity (about the centre of mass), total energy, and centre of mass velocity after collision by omega , E and v_(C) respectively, we have after collision
View SolutionA uniform bar of length 6a & mass 8m lies on a smooth horizontal table. Two point masses 'm & 2m' moving in the same horizontal plane with speeds 2v & 'v' respectively strike the bar as shown & stick to the bar after collision then

View SolutionA uniform bar of length 6a and mass 8m lies on a smooth horizontal table. Two point masses m and 2m moving in the same horizontal plane with speeds 2v and v , respectively, strike the bar (as shown in the figure) and stick to the bar after collision. Denoting angular velocity (about the centre of mass), total energy and centre of mass velocity by omega , E and V_(C) , respectively, we have after collision
View Solution