On a disc of radius R a concentric circle of radius R/2 is drawn. The disc is free to rotate about a frictionless fixed axis through its center and perpendicular to plane of the disc. All three forces ( in plane of the disc ) shown in figure are exerted tangent to their respective circular periphery. The magnitude of the net torque ( about centre of disc ) acting on the disc is :
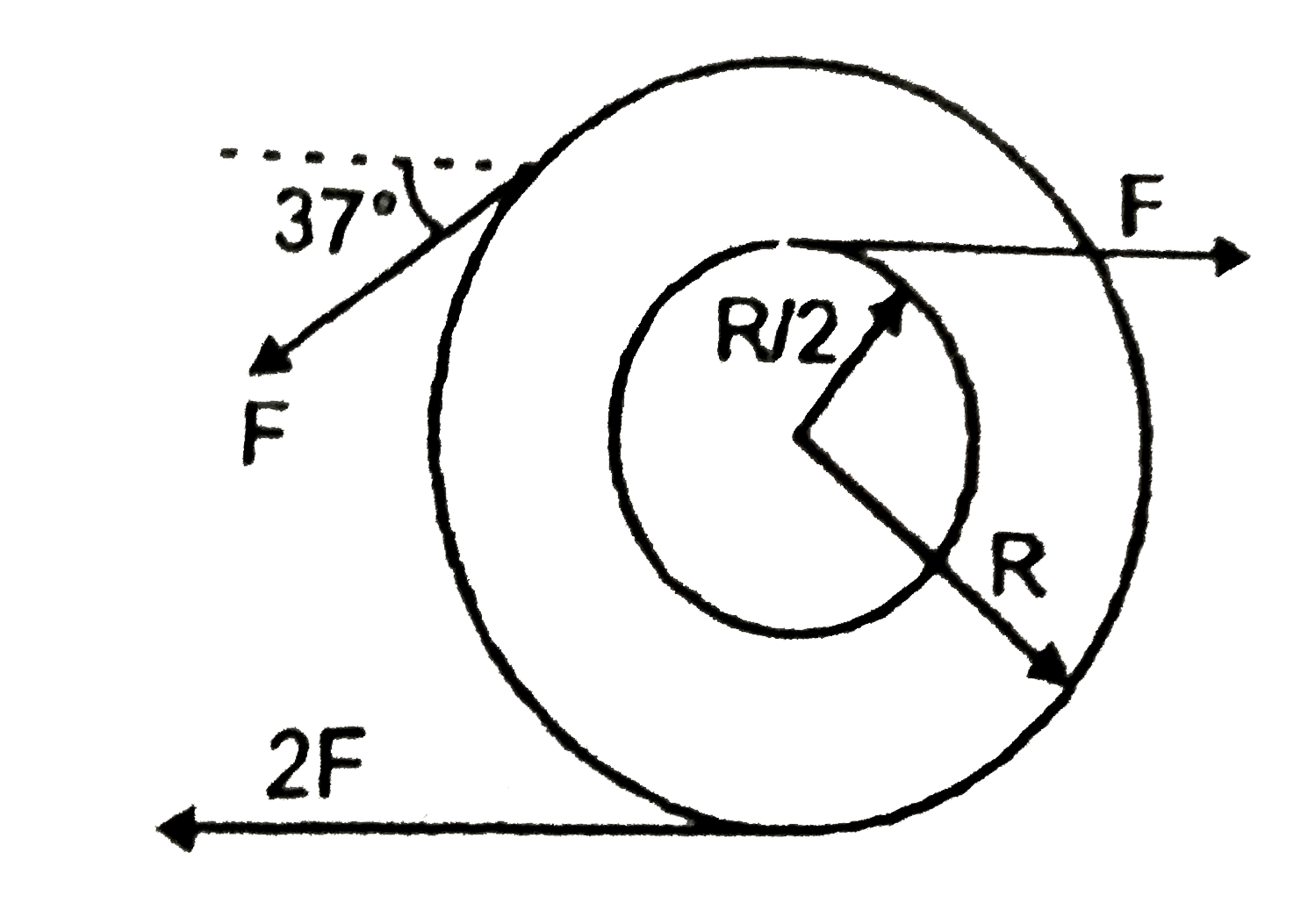
1.5FR
1.9FR
2.3FR
2.5FR
The correct Answer is:A
Torque τ=(2F)R+F(R2)+FR(−1)
=3FR2
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp61
6 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - DPP 62
4 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 59
5 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
54 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
A circular hole of radius r/2 is cut from a circular disc of radius 'r'. The disc lies in the xy plane. Determine the moment of inertia about an axis passing through the centre and perpendicular to the plane of the disc.
View SolutionA disc of mass 2 kg and diameter 40 cm is free to rotate about an axis passing through its centre and perpendicular to its plane. If a force of 50 N is applied to the disc tangentially Its angular acceleration will be
View Solution
Knowledge Check
Radius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
AR/√2BR/2C√2 RD2RA metallic circular disc having a circular hole at its centre rotates about an axis passing through its centre and perpendicular to its plane. When the disc is heated:
Aits angular speed will decreaseBits diameter will decreaseCits moment of inertia will increaseDits angular speed will increaseA metallic circular disc having a circular hole at its centre rotates about an axis passing through its centre and perpendicular to its plane. When the disc is heated:
Aits speed will decreaseBits diameter will decreaseCits moment of inertial will increaseDits speed will increase
A metallic circular disc having a circular hole at its centre rotates about an axis passing through its centre and perpendicular to its plane. When the disc is heated, choose the wrong statement
View SolutionThe moment of Inertia of an incomplete disc of mas M and radius R as shown in figure about the axis passing through the centre and perpendicular to the plane of the disc is:

View SolutionRadius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
View SolutionA disc of mass 4 kg and radius 0.2 m, makes 20 rev/s, about an axis passing through its centre and perpendicular to the plane of the disc. The angular momentum of the disc is approximately equal to
View SolutionMass of bigger disc having radius 2R is M. A disc of radius R is cut from bigger disc. Moment of intertia of disc about an axis passing through periphery and perpendicular to plane is
View Solution
