A ring of radius $R$ is made of a thin wire of material of density $ρ$ having cross section area $a .$ The ring rotates with angular velocity $ω$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $d l$ of angular width $d θ$ is shown in the figure.

The centripetal force acting on the element is

$(a . ρ d l ω^{2} R)$

$R^{2} d θ . ω^{2}$

$\frac{1}{2} a ρ d l ω^{2} R$

zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

A ring of radius $R$ is made of a thin wire of material of density $ρ$ having cross section area $a .$ The ring rotates with angular velocity $ω$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $d l$ of angular width $d θ$ is shown in the figure.

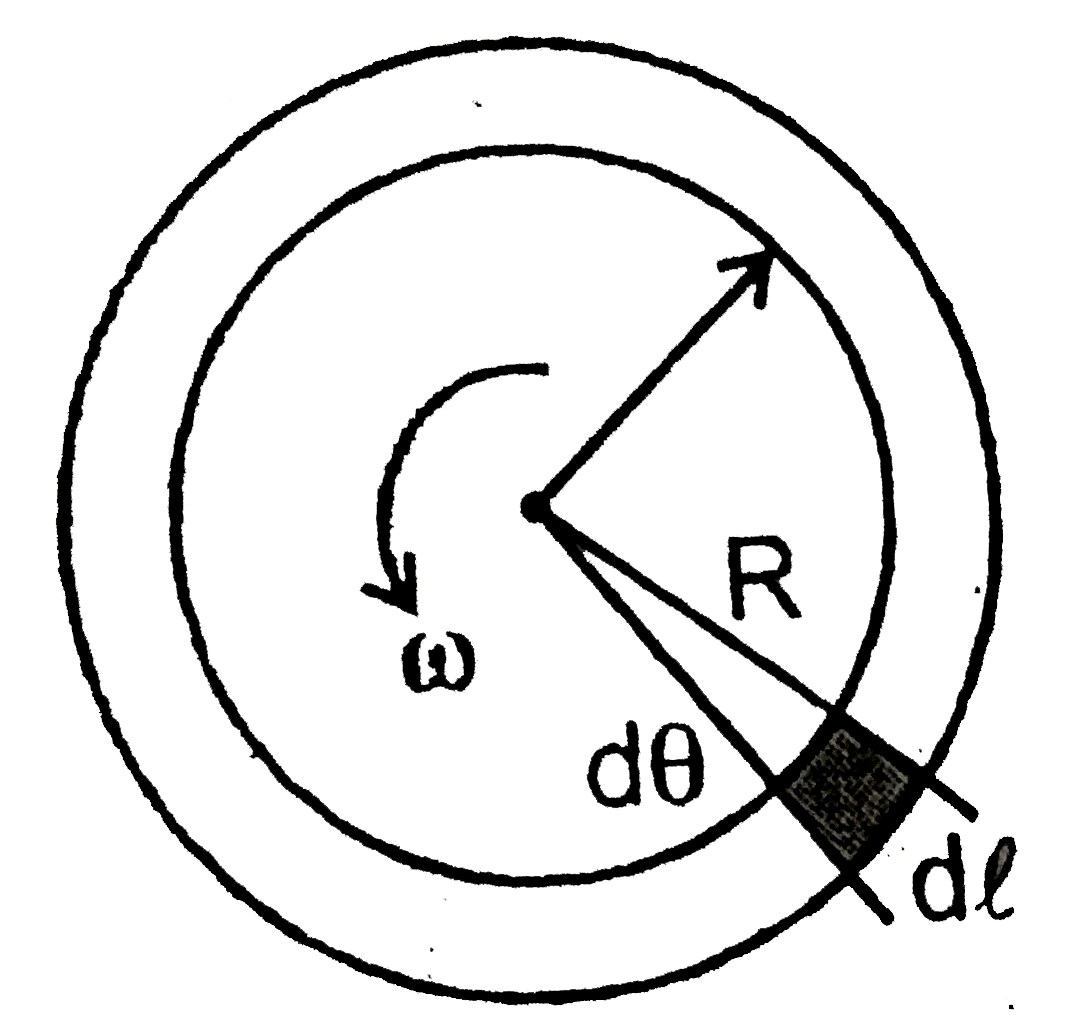
If $T$ is the tension in the ring, then
A $T = \frac{a ρ R^{2} ω^{2}}{2}$
B $T = a ρ R^{2} ω^{2}$
C $a^{2} ρ ω^{2}$
D $T = 2 a ρ R^{2} ω^{2}$
A ring of radius $R$ is made of a thin wire of material of density $ρ$ having cross section area $a .$ The ring rotates with angular velocity $ω$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $d l$ of angular width $d θ$ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius $R$ of the ring is increased to $2 R$ , the new tension will be
A $T / 2$
B $T$
C $2 T$
D $4 T$
Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
A $1 / 2 M R^{2}$
B $M R^{2}$
C $1 / 4 M R^{2}$
D $3 / 4 M R^{2}$