A particle is projected from a point (0,1) of Y-axis (assume + Y direction vertically upwards) aiming towards a point (4,9). It fell on ground along x axis in 1 sec. Taking g=10ms2 and all coordinate in metres. Find the X-coordinate where it fell.
93,0)
(4,0)
(2,0)
(2√5,0)
The correct Answer is:C
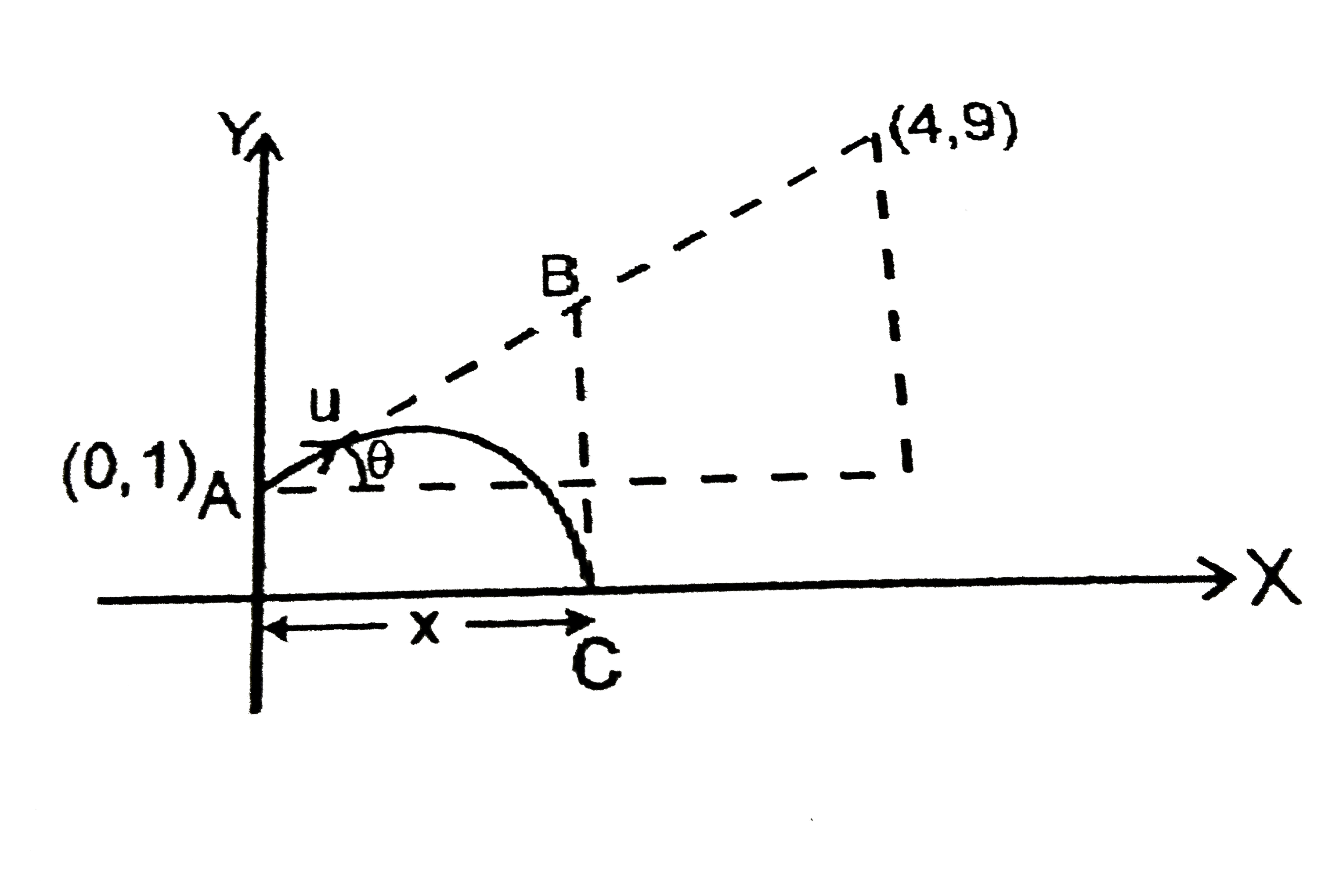
tanθ=9−14−0=2
y=uyt+12ayt2
now, −1=usinθ(1)−12g(1)2
usinθ=4 and sinθ=2√5
⇒u=2√5
now, x=ucosθ(1)=(2√5)×1√5=2m
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 27
3 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 28
7 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 25
5 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
54 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
Two lines cut on the axis of x intercepts 4and−4 and on the axis of y intercepts 2 and 6 respectively. Find the coordinates of their point of intersection.
View SolutionTaking horizontal ground as xz- plane and y-axis vertically upwards. A particle is projected from the horizontal ground from a point whose position vector is ˆiˆk meter with initial velocity from the origin when it hits ground is given by 15[11ˆi+nˆk], find n. (Take g=10m/s2 in negative y - direction)
View Solution
Knowledge Check

A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:A√3msB√4034msC2√5msD√28msA particle is projected upwards with a velocity of 110m/sec at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g =10m/sec2
A10 secondsB22 secondsC5 secomdsD10√3 secondsA particle is projected from the ground . Point of projection is taken as origin, horizontal direction as X-axis and verticle upward direction ass Y-axis. Equation of trajectory of the partcle is y=αx−βx2. Horizontal range of the projectile is
Aα+βBα+βCα/βDβ/α
A particle of mass m is released in vertical plane from a point P at x=x0 on the x-axis. It falls vertically parallel to the y-axis. Find the torque τ acting on the particle at a time about origin.

View SolutionA particle is projected upwards with a velocity of 100m/sec at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g=10m/sec2.
View SolutionTwo particles are moving along x-axis. Particle-1 starts from x=−10m with velocity 4m/s along negative x-direction and acceleration 2m/s2 along positive x-direction. Particle-2 starts from x=+2m with velocity 6m/s along positive x-direction and acceleration 2m/s2 along negative x-direction.
(a) Find the time when they collide.
(b) Find the x-coordinates where they collide. Both start simultaneously.
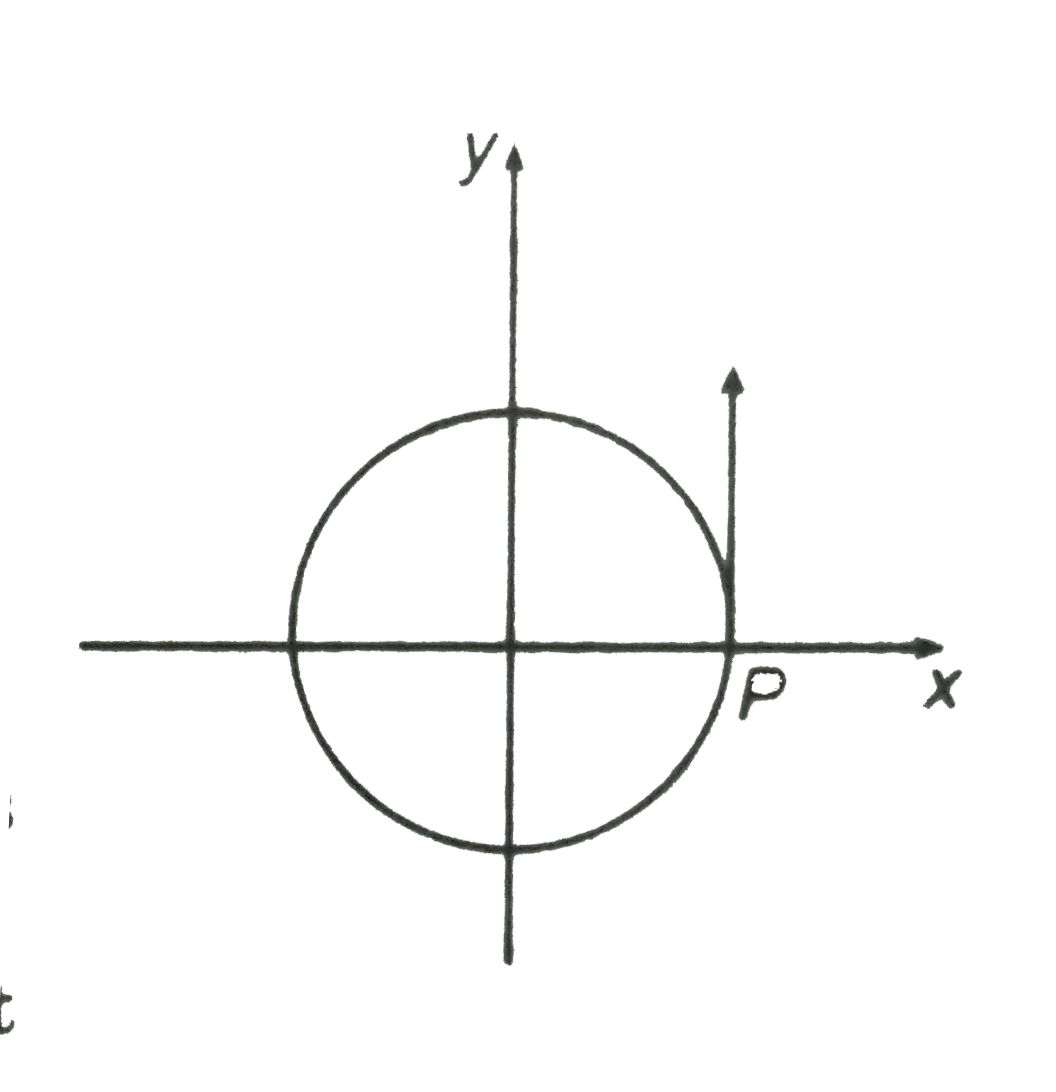
View SolutionA train is moving with a constant speed of 10m/s in a circle of radius 16πm. The plane of the circle lies in horizontal x-y plane. At time t = 0, train is at point P and moving in counter- clockwise direction. At this instant, a stone is thrown from the train with speed 10m/s relative to train towards negative x-axis at an angle of 37∘ with vertical z-axis . Find
(a) the velocity of particle relative to train at the highest point of its
trajectory.
(b) the co-ordinates of points on the ground where it finally falls and that of the hightest point of its trajectory. (Take g =10m/s2,sin37∘=35
View SolutionThe coordinates of a point A on y-axis, at a distance of 4 units from x-axis and below it, are
View Solution