A particle is moving in a straight line as $:$
$a = 1 m / s^{2} 0 \leq t \leq 10$
$a = 2 t t > 10$
where $t$ is the time and $a$ is the acceleration. If initial velocity of the particla is zero then $:$

A

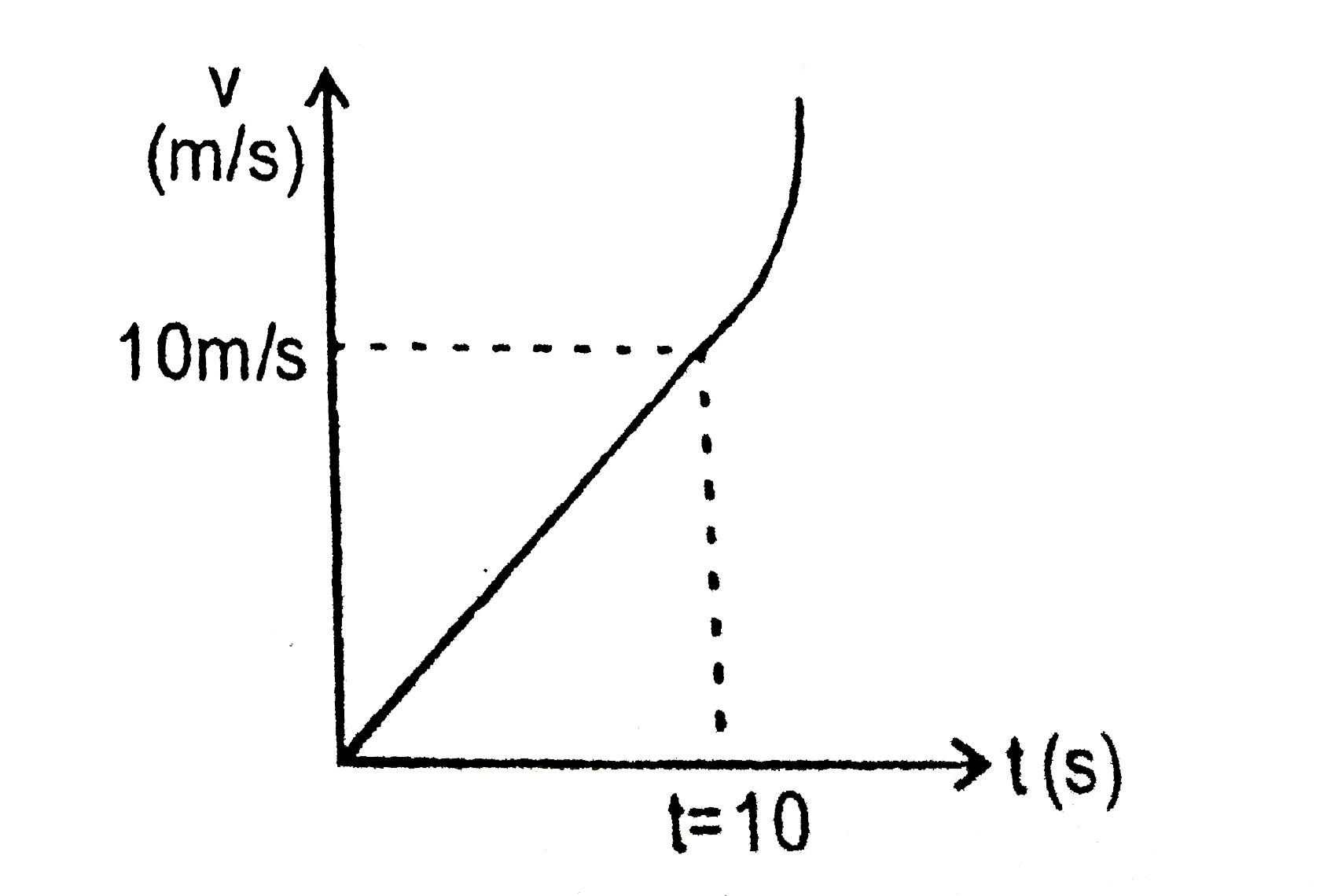
velocity of the particle at $t = 20 sec$ is $310 m / s$

B

displacement of the particle in $t = 20 s$ is $1483.33 m$ .

C

acceleration the particle at $t = 15 s$ is $32 m / s^{2}$ .

D

$v - t$ graph is

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, D

For $t = 0$ to $t = 10$
$V = u + a t$
$V = 0 + (1) (10) = 10 m / s$ /
$a = 2 t$
$v \int 10 d v = 2 20 \int 10 t d t ... . (1)$
$v - 10 = 2 {∣ ∣ ∣ \frac{t^{2}}{2} ∣ ∣ ∣}_{10}^{20}$
$v - 10 = {(20)}^{2} - {(10)}^{2} = 300$
$v = 310 m / s$ .
$(b)$ For $t = 0$ to $t = 10 s$ .
$S = u t + \frac{1}{2} a t^{2}$
$\Rightarrow S = (0) (10) + \frac{1}{2} \times 1 \times {(10)}^{2} = 50 m$
From $(1)$
$v - 10 = t^{2} - {(10)}^{2}$
$v = t^{2} - 90$
$S \int 50 d s = 20 \int 10 (t^{2} - 90) d t$
$S - 50 = [\frac{{(20)}^{3}}{3} - 90 \times 20] - [\frac{{(10)}^{3}}{3} - 90 \times 10]$
$S = 50 + \frac{8000}{3} - 1800 - \frac{1000}{3} + 900$
$S = 50 + \frac{7000}{3} - 900$
$\Rightarrow S = 1483.33 m$

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The displacement of a particle moving in a straight line, is given by $s = 2 t^{2} + 2 t + 4$ where $s$ is in metres and $t$ in seconds. The acceleration of the particle is.
A $2 m / s^{2}$
B $4 m / s^{2}$
C $6 m / s^{2}$
D $8 m / s^{2}$
Question 2 - Select One or More
A particle is moving on a straight line with constant retardation of $1 m / s^{2}$ . Its initial velocity is $10 m / s$ .
AThe distance travelled by the particle till it comes to rest is 50 m.
BThe average velocity till it comes to rest is $5 m / s$ .
CIt comes to rest in 5 sec.
DIts velocity after 5 sec is $5 m / s$
Question 3 - Select One
The equation of a particle moving along a straight line is $s = 2 t^{3} - 9 t^{2} + 12 t$ , where the units of s and t are cm and s. the acceleration of the particle will be zero after
A $\frac{3}{2}$ s
B $\frac{2}{3} s$
C $\frac{1}{2} s$
D1s

RESONANCE-DAILY PRACTICE PROBLEMS-dpp 26

Home
Ask Doubt
Profile