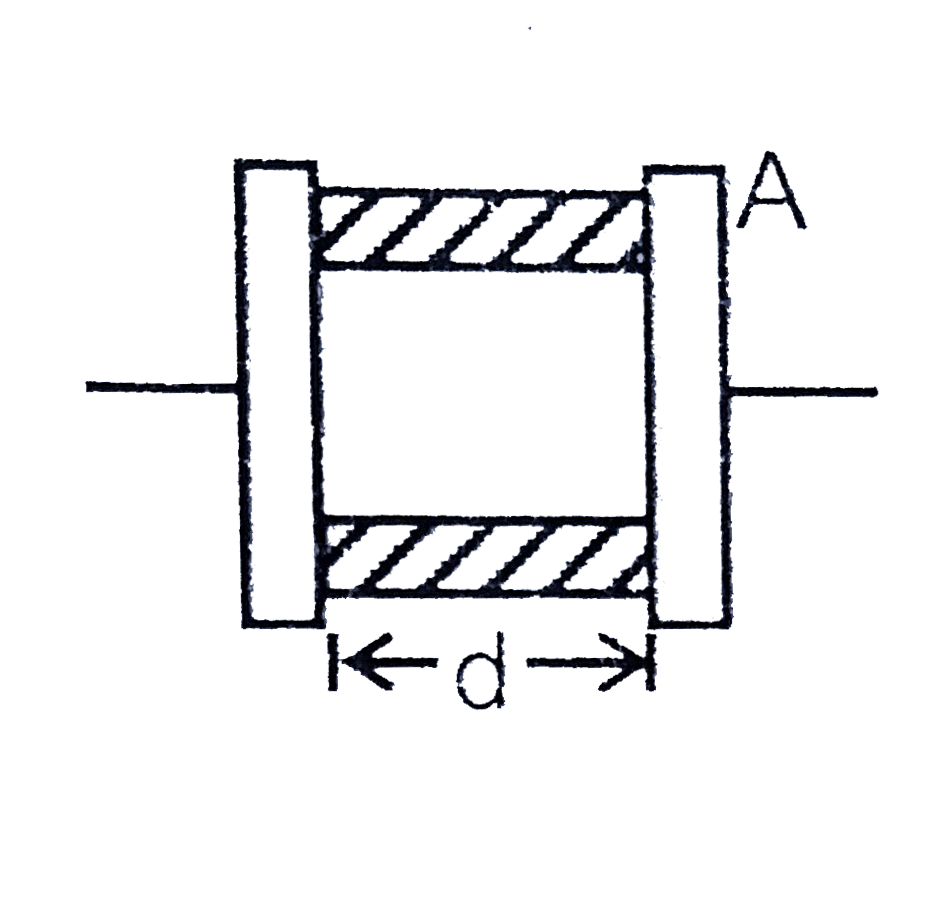
A parallel plate capacitor of area A and separation d is provided with thin insulating spacers to keep its plates aligned in an environment of fluctuating temperature. If the coefficient of thermal expansion of material of the plate is α, find the coefficient of thernal expansion (αs) of the spacers in order that the capacitance does not vary with temperature. (Ignore effect of the spacers on capacitance.)
αs=α2
αs=3α
αs=2α
αs=α
The correct Answer is:C
C=∈0Ax, where x is separation between plates.
1CdCdT=1AdAdT−1xdxdT
For dCdT=0,1xdxdT=1AdAdT
⇒αs=2α
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
A capacitor is to be designed to operate, with constant capacitance, in an environment of fluctuating temperature. As shown in the figure the capacitor is a paralel plate capacitor with 'spacer' to change the distance for compensation of temperature effect. If α1 be the coefficient of linear expansion of plates and α2 that of spacer, find the rate of change of capacitance with temperature and hence find the condition for no change in capacitance with change of temperature. The capacitance of the capacitor is equal to C.
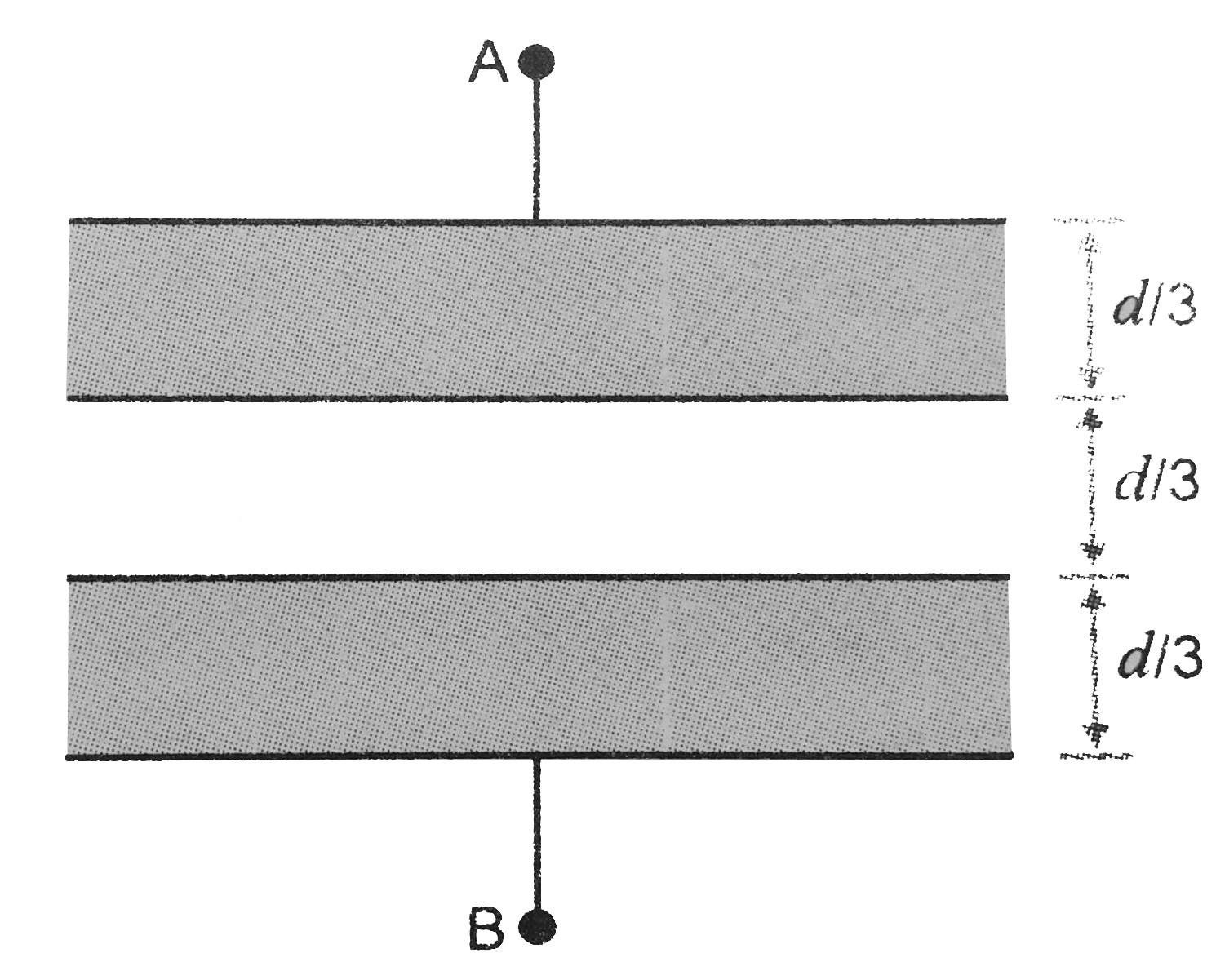
View SolutionFig shows a parallel plate capacitor of plate area A and plate separation d. Its entire space is filled with three different dielectric slabs of same thickness. Find the equivalent capacitance of the arrangment.
View Solution
Knowledge Check
A parallel plate capacitor of plate area A and separation d is filled with two materials each of thickness d2 and dielectric constants ∈1 and ∈2 respectively. The equivalent capacitance will be
 A∈0Ad(∈1+∈2)B∈0Ad(∈1+∈2)∈1∈2C2∈0Ad∈1∈2(∈1+∈2)D2∈0Ad(∈1+∈2)∈1∈2
A∈0Ad(∈1+∈2)B∈0Ad(∈1+∈2)∈1∈2C2∈0Ad∈1∈2(∈1+∈2)D2∈0Ad(∈1+∈2)∈1∈2A metal plate has an area of 10m2 . On raising its temperature by 20∘C . The change in area if the coefficient of linear expansion is a, will be
A100αB200αC400αD600αA fluid having a thermal coefficient of volume expansion γ is filled in a cylindrical vessel up to a height h0. The coefficient of linear thermal expansion of the material of the vessel is α . If the fluid is heated with the vessel , then find the level of liquid when temperature increases by Δθ.
Ah0(1+γΔθ)(1+2αΔθ)Bh0(1+γΔθ)(1+3αΔθ)Ch0(1+γΔθ)(1+αΔθ)Dh0(1+γΔθ)
It was found that resistance of a cylindrical specimen of a wire does not change with small change in temperature. If its temperature coefficient of resistivity is αR then find its thermal expansion coefficient (α).
View SolutionA parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

View SolutionA rod AB of length l is pivoted at an end A and freely rotated in a horizontal plane at an angular speed ω about a vertical axis passing through A. If coefficient of linear expansion of material of rod is α, find the percentage change in its angular velocity if temperature of system is incresed by ΔT
View SolutionFind out the capacitance of parallel plate capacitor of plate area A and plate separation d.
View SolutionImagine a hypothetical material whose average temperature coefficient of linear expansion is 0.1/.∘C .Find the fractional increase in area of thin square plate of above material when temperature is increase by 10∘C
View Solution