A square is inscribed in circle of radius R, a circle is inscribed in the square, a new square in the circle and so on for n times.
Sum of the areas of all circles is
4πR2(1−(12)n)
2πR2(1−(12)n)
3πR2(1−(13)n)
πR2(1−(12)n)
The correct Answer is:B
Step by step video, text & image solution for A square is inscribed in circle of radius R, a circle is inscribed in the square, a new square in the circle and so on for n times. Sum of the areas of all circles is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CONTINUITY AND DIFFERENTIABILITYBook:AAKASH INSTITUTEChapter:CONTINUITY AND DIFFERENTIABILITYExercise:Section - E
Explore 4 Videos
- CONTINUITY AND DIFFERENTIABILITYBook:AAKASH INSTITUTEChapter:CONTINUITY AND DIFFERENTIABILITYExercise:Section - F
Explore 3 Videos
- CONTINUITY AND DIFFERENTIABILITYBook:AAKASH INSTITUTEChapter:CONTINUITY AND DIFFERENTIABILITYExercise:Section - C ( More than one options are correct )
Explore 5 Videos
- CONIC SECTIONSBook:AAKASH INSTITUTEChapter:CONIC SECTIONSExercise:SECTION - J ( Aakash Challengers Questions )
Explore 14 Videos
- DETERMINANTS Book:AAKASH INSTITUTEChapter:DETERMINANTS Exercise:SECTION - J
Explore 12 Videos
Similar Questions
Knowledge Check
If a square is inscribed in a circle of radius 7 cm, then find the area of the square (in cm2).
A49B98C196D392A square is inscribed in a circle then another circle is inscribed in the square . Another square is then inscribed in the circle. Finally a circle is inscirbed in the innermost square . Thus there are 3 circles and 2 squares as shown in the figure . The radius of the outer - most circle is R .
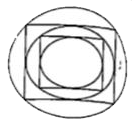
What is the ratio of sum of circumference of all the circles to the sum of perimeters of all the squares ?A(2+√3)πRB(3+√2)πRC3√3πRDnone of theseA square is inscribed in a circle then another circle is inscribed in the square . Another square is then inscribed in the circle. Finally a circle is inscirbed in the innermost square . Thus there are 3 circles and 2 squares as shown in the figure . The radius of the outer - most circle is R .
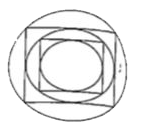
What is the sum of areas of all the squares shown in the figure ?A3R2B3√2R2C3√2R2Dnone of these
In a circle of radius R a square is inscribed, then a circle is inscribed in the square, a new square in the circle and so on for n times. If the ratio of the limit of the sum of areas of all the circles to the limit of the sum of areas of all the squares as n→∞ is k, then find the value of 4kπ.
View SolutionA square ABCD is inscribed in a circle of radius r. Find the area of the square.
View Solution
A square of side x is inscribed in a circle of radius 4 cm. Then x is
View Solutionone circle is inscribbed in a square with area "9pi" then area of square?
View SolutionA square is inscribed in a circle then another circle is inscribed in the square . Another square is then inscribed in the circle. Finally a circle is inscirbed in the innermost square . Thus there are 3 circles and 2 squares as shown in the figure . The radius of the outer - most circle is R .
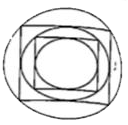
What is the radius of the inner - most circle ?
View Solution