The figure shows the cross-section of the outer wall of a house buit in a hill-resort to keep the house insulated from the freezing temperature of outside. The wall consists of teak wood of thickness L1 and brick of thickness (L2=5L1), sandwitching two layers of an unknown material with identical thermal conductivites and thickness. The thermal conductivity of teak wood is K1 and that of brick is (K2=5K). Heat conducion through the wall has reached a steady state with the temperature of three surfaces being known. (T1=25∘C,T2=20∘C and T5=−20∘C). Find the interface temperature T4 and T3.
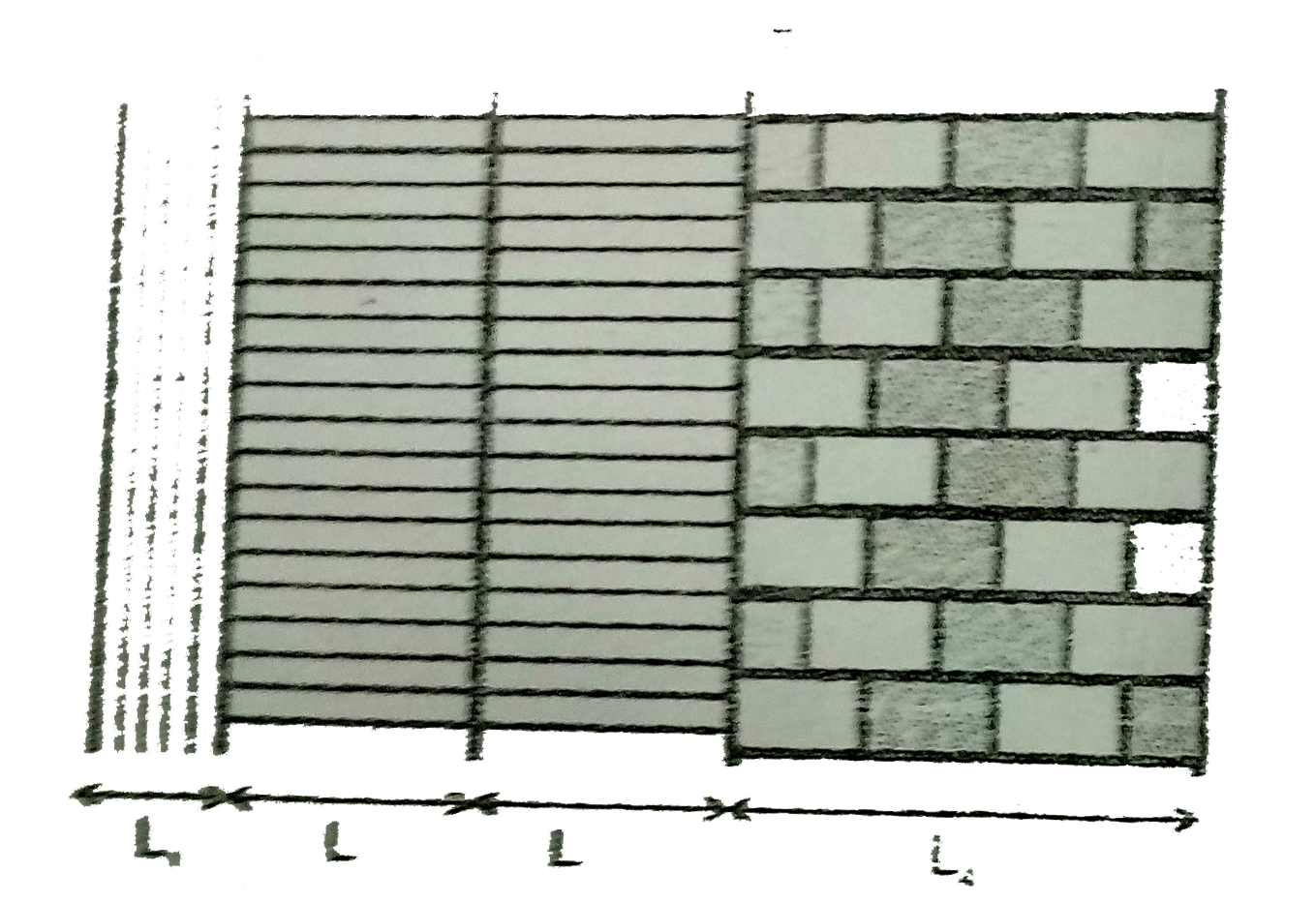
Let interface area be A. then thermal resistance of wood.
R1=L1K1A and that of brick wall
R2=L2K2A=5L15K1A=R1
Let thermal resistance of the each sand witch layer =R. Then the above wall can be visualised as a circuit
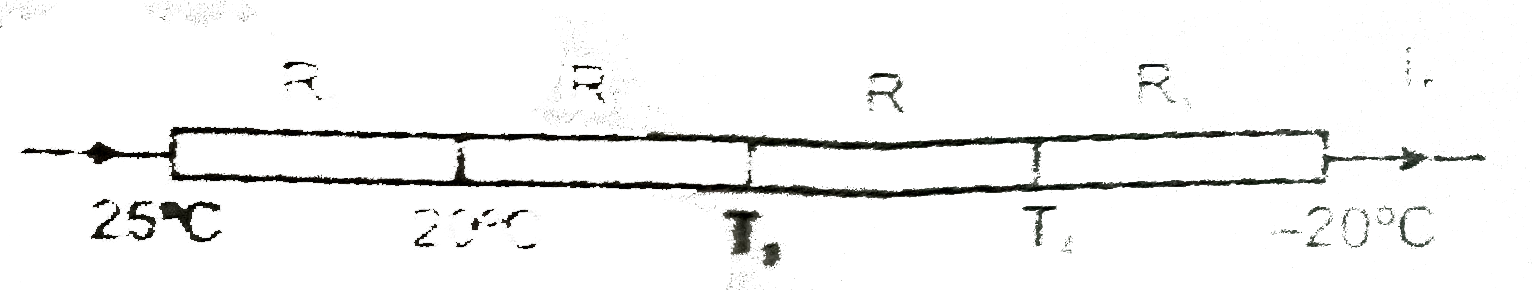
thermal current through each walls is same.
Hence 25−20R1=20−T3R=T3−T4R=T4+20R1
⇒25−20=T4+20⇒T4=−15∘C
also, 20−T3=T3−T4⇒T3=20+T42=2.5∘C
Topper's Solved these Questions
- HEAT TRANSFERBook:RESONANCEChapter:HEAT TRANSFERExercise:Board Level Exercise
Explore 14 Videos
- HEAT TRANSFERBook:RESONANCEChapter:HEAT TRANSFERExercise:Exercise-1
Explore 1 Video
- HEAT TRANSFERBook:RESONANCEChapter:HEAT TRANSFERExercise:Advancel Level Problems
Explore 17 Videos
- GRAVITATIONBook:RESONANCEChapter:GRAVITATIONExercise:Exercise
Explore 21 Videos
- KINEMATICSBook:RESONANCEChapter:KINEMATICSExercise:Exercise
Explore 65 Videos
Similar Questions
What is the S.I. Unit of thermal conductivity?
View SolutionA uniform slab of dimension 10cm×10cm×1cm is kept between two heat reservoir at temperatures 10∘C and 90∘C The larger surface areas touch the reservoirs. The thermal conductivity of the material is 0.80Wm−1.∘C−1 . Find the amount of heat flowing through the slab per minute.
View SolutionSeven rods A,B,C,D,E,FandG are joined as shown in figure. All the rods have equal cross-sectional area A and length l. The thermal conductivities of the rods are KA=KC=K0, KB=KD=2K0, KE=3K0, KF=4K0 and KG=5K0 . The rod E is kept at a constant temperature T1 and the rod G is kept at a constant temperature T2(T2>T1) . (a) Show that the rod F has a uniform temperature T=(T1+2T2)/3 . (b) Find the rate of heat flowing from the source which maintains the temperature T2 .

View SolutionA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
View SolutionA particle moves along a path ABCD as shown in the figure. Then the magnitude of net displacement of the particle from position A to D is:

View SolutionA composite slab is prepared by pasting two plates of thickness L1 and L2 and thermal conductivites K1 and K2 . The slab have equal cross-sectional area. Find the equivalent conductivity of the composite slab.
View SolutionFigure shows in cross section a wall consisting of four layers with thermal conductivities K_(1) = 0.06 W // mK, K_(3) = 0.04 W// mK and K_(4) = 0.10 W// mK . The layer thickness are L_(1) = 1.5 cm, L_(3) = 2.8 cm and L_(4) = 3.5 cm . The temperature of interfaces is as shown in figure. energy transfer through the wall is in steady state. the temperature of the interface between layer 3 and 4 is:
View SolutionTemperature shows in cross section a wall consisting of four layers with thermal conductivities K_(1)=0.06W//mK,K_(3)=0.04W//mK and K_(4)=0.10W//mK the layer thickness are L_(1)=1.5cm,L_(3)=2.8cm and L_(4)=3.5 cm. The temperature of interfaces is as shown in figure energy transfer through the wall is in steady state. Q. Temperature of outer surface of sphere is
View SolutionTemperature shows in cross section a wall consisting of four layers with thermal conductivities K_(1)=0.06W//mK,K_(3)=0.04W//mK and K_(4)=0.10W//mK the layer thickness are L_(1)=1.5cm,L_(3)=2.8cm and L_(4)=3.5 cm. The temperature of interfaces is as shown in figure energy transfer through the wall is in steady state. Q. Thickness of hollow sphere is
View SolutionTwo plates each of area A thickness L1 and L2 thermal conductivities K1 and K2 respectively are joined to from a single plate of thickness (L1+L2) If the temperatures of the free surfaces are θ1 and θ2 Calculate
(a) Rate of flow of heat
(b) Temperature of interface
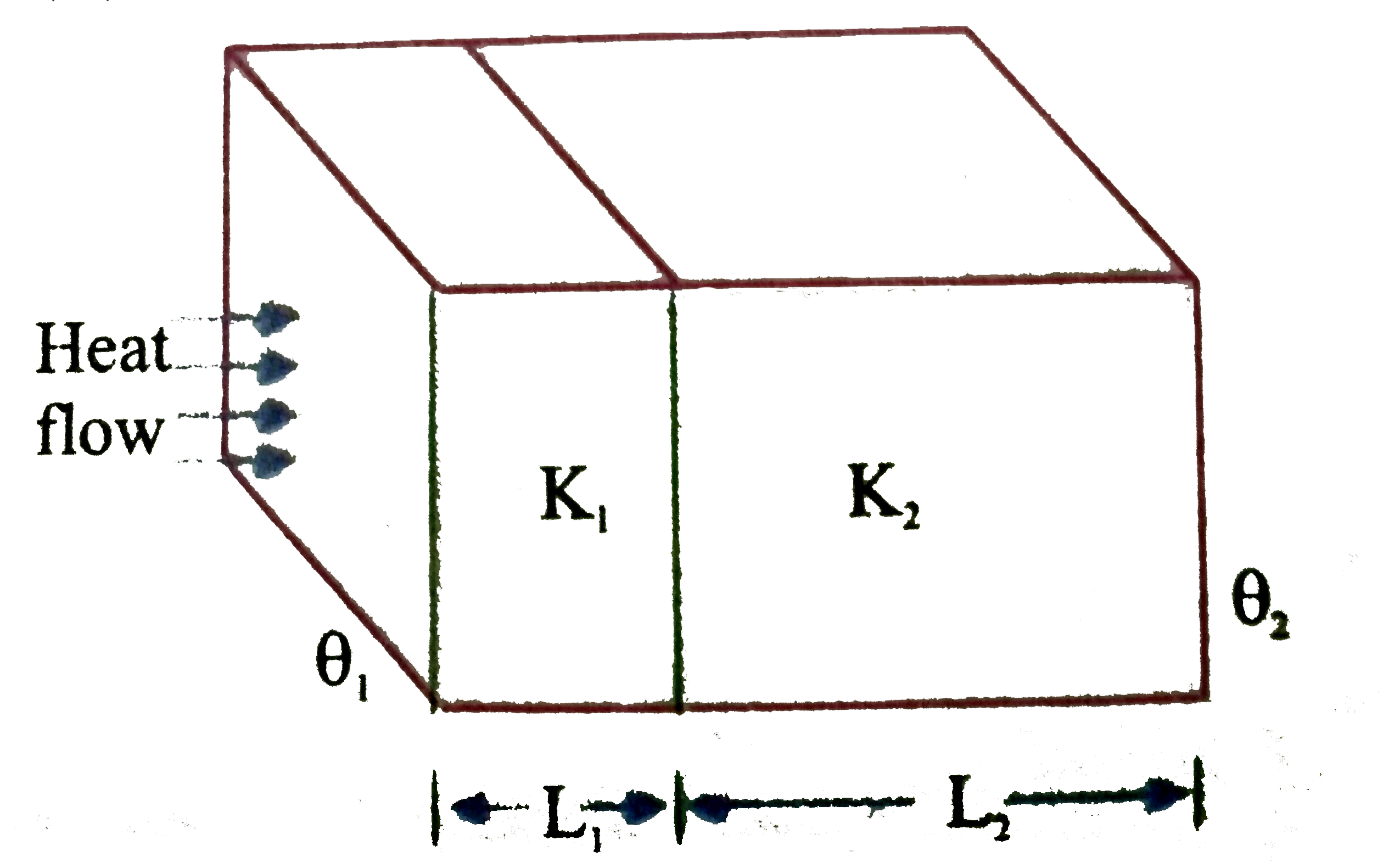 .
.
View SolutionTwo metal plates of same area and thickness l_(1) and l_(2) are arranged in series If the thermal conductivities of the materials of the two plates are K_(1) and K_(2) The thermal conductivity of the combination is .
View SolutionA slab consists of two layers of different materials of the same thickness and having thermal conductivities K_(1) and K_(2) . The equivalent thermal conductivity of the slab is
View SolutionA slab consists of two parallel layers of two different materials of same thickness having thermal conductivities K_(1) and K_(2) . The equivalent conductivity of the combination is
View SolutionA slab consists of two parallel layers of two different materials of same thickness having thermal conductivities K_(1) and K_(2) , the equivalent conductivity of the combination is :
View SolutionA slab consists of two parallel layers of two different materials of same thickness having thermal conductivities k_(1) & k_(2) . The equivalent conductivity of the combination is :
View Solution