A block of mass m is pushed towards the movable wedge of mass M and height h, with a velocity v0. All surfaces are smooth . The minimum value of v0 for which the block will reach the top of the wedge is
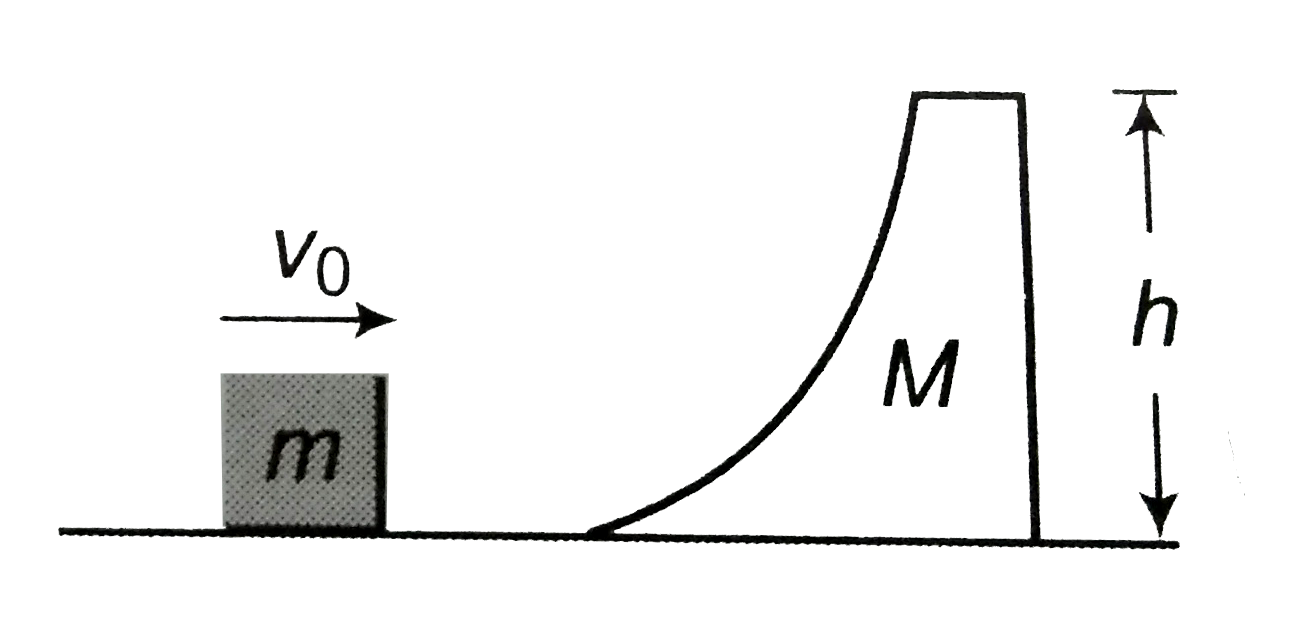
√2gh
η√2gh
√2gh(1+1η)
√2gh(1−1η)
The correct Answer is:C
A small block…………..

Applying conservation of momentum.
Let velocity of the system be v, when the mass reaches the tap.
mv=(ηm+m)v , ⇒v=vη+1
Now, applying conservation energy.
12mv∘=12(η+1)m(vη+1)+mgh
⇒12mv2=12m2(η+1)+mgh , ⇒v=√(2gh)(η+1)η
Hence option : (C)
Similar Questions
A block of mass 1kg 'is pushed towards a movable wedge of mass 2kg and height h=30cm, with a velocity u=6ms. Before striking the wedge it travels 2m on a rough horizontal portion. The velocity is just sufficient for the block to reach the top of the wedge. Assuming all the surfaces are smooth except the given horizontal part and collision of block and wedge is jerkless, the friction coefficient of the rough horizontal part is (Round off the answer to two decimal places)
'(##CEN_KSR_PHY_JEE_C08_E01_015_Q09##)'
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View Solution
Knowledge Check
A block of mass m is pushed towards a movable wedge of mass nm and height h, with a velocity u. All surfaces are smooth. The minimum value of u for which the block will reach the top of the wedge is
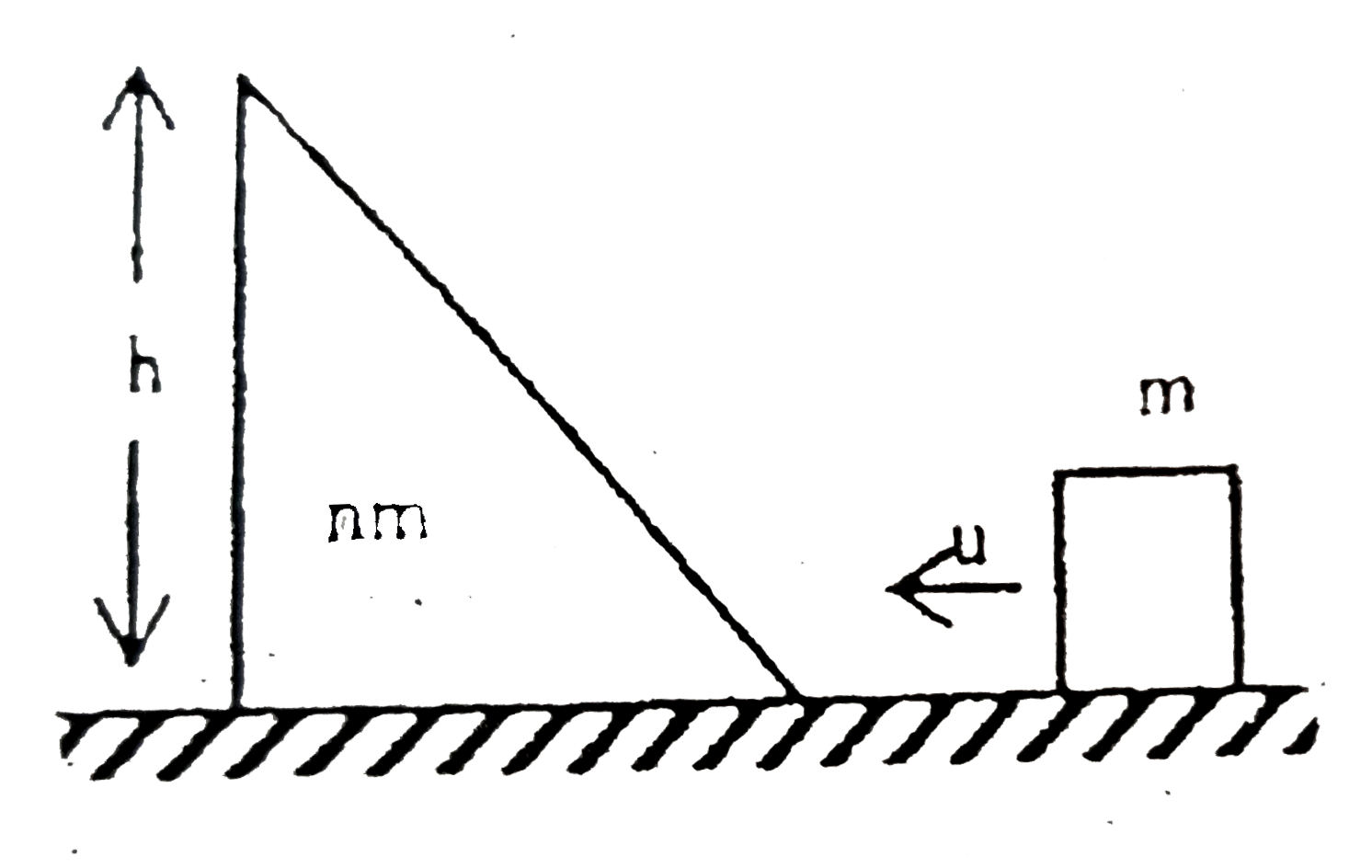 A√2ghBn2ghC√2gh(1+1n)D√2gh(1−1n)
A√2ghBn2ghC√2gh(1+1n)D√2gh(1−1n)A small block of mass m is pushed towards a movable wedge of mass ηm and height h with initial velocity u.All surface are smooth. The minimum value of u for which the block will reach the top of the wedge:
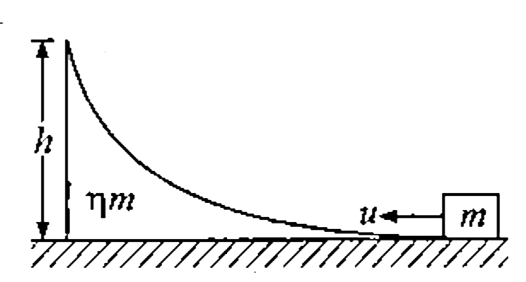 A√2ghBη√2ghC√2gh(1+1η)D√2gh(1−1η)
A√2ghBη√2ghC√2gh(1+1η)D√2gh(1−1η)A small block of mass m is pushed towards a movable wedge of mass ηm and height h with initial velocity u.All surface are smooth. The minimum value of u for which the block will reach the top of the wedge:
 A√2gh(1+1h)B√(1+12gh)nC√n2ghD√1+h2gh
A√2gh(1+1h)B√(1+12gh)nC√n2ghD√1+h2gh
A block of mass m is at rest over the rough surface of the fixed wedge.

View SolutionA block of mass m is pushed towards a movable wedge of mass nm and height h, with a velocity u. All surfaces are smooth. The minimum value of u for which the block will reach the top of the wedge is

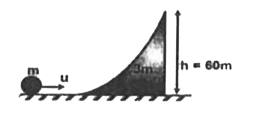
View SolutionA ball of mass 'm' is pushed with a velocity u towards a movable wedge of mass 3m which is at rest. Height of the wedge is 60m. Assume all surfaces to be smooth. The minimum value of 'u' for which the block will reach the top of the wedge is ( There is no loss of energy when the ball tries to rise on the wedge ) ( take g =10m/s2 )
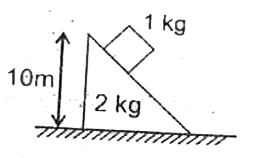
View SolutionA block of mass 1 kg is released from the top of a wedge of mass 2 kg. Height of wedge is 10 meter and all the surfaces are frictionless. Then
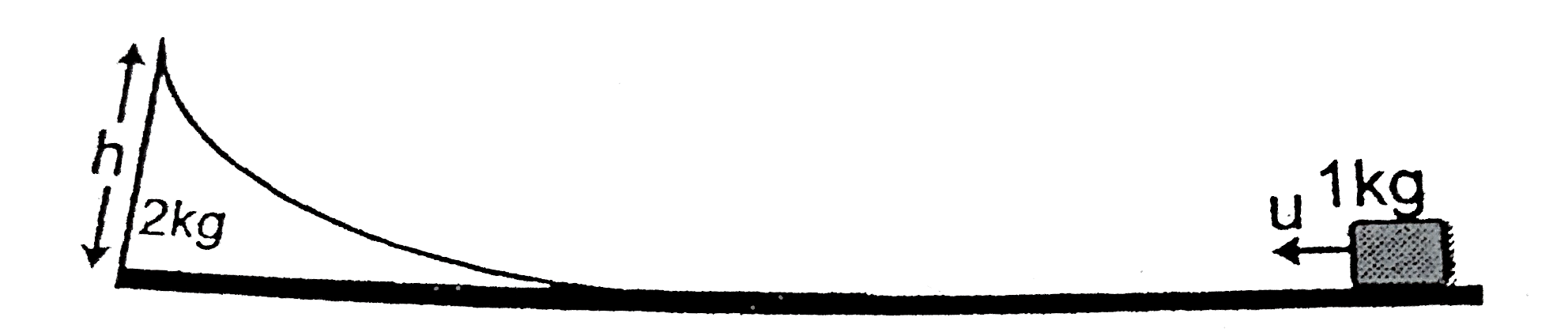
View SolutionA block of mass 1kg is pushed on a movable wedge of mass 2kg and height h=30cm with a velocity u=6m/sec. Before striking the wedge it travels 2m on a rough horizontal portion. Velocity is just sufficient for the block to reach the top of the wedge. Assuming all surfaces are smooth except the given horizontal part and collision of block and wedge is jerkless, the friction coefficition of the rough horizontal part is :
View Solution
