Find the distance at which probability of finding electron is maximum

One of the major requirement in atomic structure is determination of location of electron inside an atom. The wave mechanical model establishes this in accordance with Heisenberg's uncertainity principle through the concept of orbitals. The orbitals are defined as that '3D' space in which probability of finding electron is maximum and are represented by wave functions $Ψ_{n, l, m}$ where n,l and m are quantum number. The variation of $Ψ$ is analysed in terms of polar coordinates and hence $Ψ = f (r, 0, ϕ)$ where 'r' represents radius vector and 0 and $ϕ$ represents angle $(∠)$ Which the radius vector with x-axis respectively. The expressions of $Ψ_{r, 0, ϕ}$ are often given in terms of $σ$ instead of r where $σ = \frac{2 Z r}{n α_{0}}$ and Z = atomic number and n= shell number.
If an orbital is represented as:
$Ψ_{r, 0, ϕ} = \frac{2}{3} {(\frac{1}{3 α_{0}})}^{3 / 2} . (σ - 1) (σ^{2} - 8 σ + 12)$
$. σ^{- σ / 2} . cos 0$
belong to which orbital?

View Solution

The radial distribution functions $[P (r)]$ is used to determine the most probable radius, which is used to find the electron in a given orbital $\frac{d P (r)}{d r}$ for $1 s$ -orbital of hydrogen like atom having atomic number $Z$ , is $\frac{d P}{d r} = \frac{4 Z^{3}}{a_{0}^{3}} (2 r - \frac{2 Z r^{2}}{a_{0}}) e_{0}^{- 2 Z r / a_{0}}$ :

View Solution

The Schrodinger wave equation for H-atom is
$\nabla^{2} Ψ = \frac{8 π^{2} m}{h^{2}} (E - V) Ψ = 0$
Where $\nabla^{2} = \frac{\partial^{2}}{\partial x^{2}} + \frac{\partial^{2}}{\partial y^{2}} + \frac{\partial^{2}}{\partial z^{2}}$
E = Total energy and V=potential energy wave function $Ψ_{(\begin{matrix} r & θ & ϕ \end{matrix})} R_{(r)} Θ_{(θ)} Φ_{(ϕ)}$
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. $Θ$ and $Φ$ are angular wave function. $R^{2}$ is known as radial probability density and $4 π r^{2} R^{2} d r$ is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
$Ψ_{1 s} = \sqrt{\frac{1}{π a_{0}^{a}}} e_{r}^{- z_{r} / a_{0}}$
$Ψ_{2 s} = {(\frac{Z}{2 a_{0}})}^{½} (1 - \frac{Z r}{a_{0}}) e^{- \frac{Z r}{a_{0}}}$
The plot of radial probability function $4 π r^{2} R^{2}$ aganist r will be:
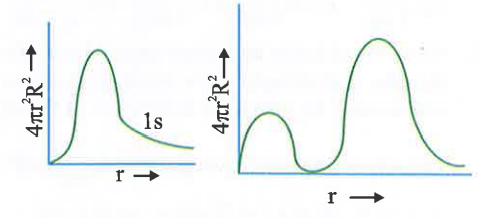
ocr_image
Answer the following questions:
The following graph is plotted for ns-orbitals

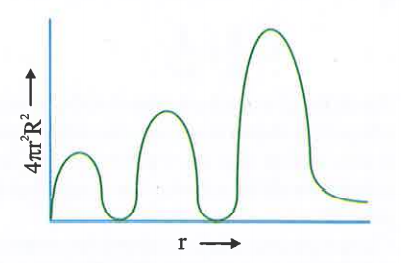
The value of 'n' will be:

View Solution

The Schrodinger wave equation for H-atom is
$\nabla^{2} Ψ = \frac{8 π^{2} m}{h^{2}} (E - V) Ψ = 0$
Where $\nabla^{2} = \frac{\partial^{2}}{\partial x^{2}} + \frac{\partial^{2}}{\partial y^{2}} + \frac{\partial^{2}}{\partial z^{2}}$
E = Total energy and V=potential energy wave function $Ψ_{(\begin{matrix} r & θ & ϕ \end{matrix})} R_{(r)} Θ_{(θ)} Φ_{(ϕ)}$
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. $Θ$ and $Φ$ are angular wave function. $R^{2}$ is known as radial probability density and $4 π r^{2} R^{2} d r$ is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
$Ψ_{1 s} = \sqrt{\frac{1}{π a_{0}^{a}}} e_{r}^{- z_{r} / a_{0}}$
$Ψ_{2 s} = {(\frac{Z}{2 a_{0}})}^{½} (1 - \frac{Z r}{a_{0}}) e^{- \frac{Z r}{a_{0}}}$
The plot of radial probability function $4 π r^{2} R^{2}$ aganist r will be:
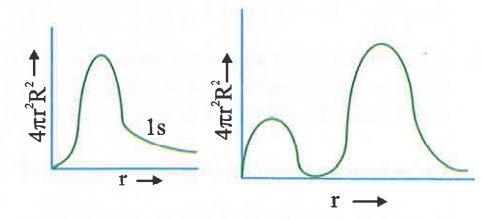
ocr_image
Answer the following questions:
The value of radius 'r' for 2s atomic orbital of H-atom at which the radial node will exist may be given as:

View Solution

The Schrodinger wave equation for H-atom is
$\nabla^{2} Ψ = \frac{8 π^{2} m}{h^{2}} (E - V) Ψ = 0$
Where $\nabla^{2} = \frac{\partial^{2}}{\partial x^{2}} + \frac{\partial^{2}}{\partial y^{2}} + \frac{\partial^{2}}{\partial z^{2}}$
E = Total energy and V=potential energy wave function $Ψ_{(\begin{matrix} r & θ & ϕ \end{matrix})} R_{(r)} Θ_{(θ)} Φ_{(ϕ)}$
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. $Θ$ and $Φ$ are angular wave function. $R^{2}$ is known as radial probability density and $4 π r^{2} R^{2} d r$ is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
$Ψ_{1 s} = \sqrt{\frac{1}{π a_{0}^{a}}} e_{r}^{- z_{r} / a_{0}}$
$Ψ_{2 s} = {(\frac{Z}{2 a_{0}})}^{½} (1 - \frac{Z r}{a_{0}}) e^{- \frac{Z r}{a_{0}}}$
The plot of radial probability function $4 π r^{2} R^{2}$ aganist r will be:
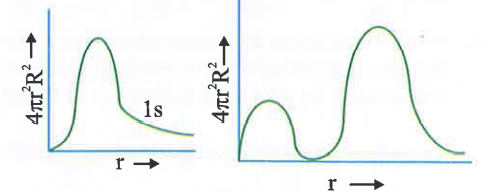
ocr_image
Answer the following questions:
What will be number of angular nodes and spherical nodes for 4f atomic orbitals respectively.

View Solution

Find the distance at which probability of finding electron is maximum for $1 s$ orbital in a He atom. The wave function orbital given as.
$ψ_{1 s} = \frac{4}{a_{0}^{3 / 2}} e^{\frac{2 r}{a_{0}}}$

Topper's Solved these Questions

Similar Questions

Knowledge Check

Probability of finding the electron $ψ^{2}$ of 's' orbital does not depend upon

The radius of maximum probability for $1 s$ orbital of $H$ atom is

ALLEN-ATOMIC STRUCTURE-Exercise - 05[A]

Find the distance at which probability of finding electron is maximum for 1s orbital in a He atom. The wave function orbital given as. ψ1s=4a3/20e2ra0

Probability distribution function is P(r)=ψ2=4πr2=(16a30)e(4ra0)4πr2 ⇒P(r)=k.r2.e.4ra0 differentating dP(r)dr=2r.e.4ra0−(4a0)r2.e4ra0=0 ⇒1=2ra0⇒r=a02 implies probability of finding electron is maximum at distance a02 from nucleus.

Topper's Solved these Questions

Similar Questions

Knowledge Check

Probability of finding the electron ψ2 of 's' orbital does not depend upon

The radius of maximum probability for 1s orbital of H atom is

ALLEN-ATOMIC STRUCTURE-Exercise - 05[A]

Find the distance at which probability of finding electron is maximum for $1 s$ orbital in a He atom. The wave function orbital given as.
$ψ_{1 s} = \frac{4}{a_{0}^{3 / 2}} e^{\frac{2 r}{a_{0}}}$

Probability of finding the electron $ψ^{2}$ of 's' orbital does not depend upon

The radius of maximum probability for $1 s$ orbital of $H$ atom is