The radial distribution functions is used to determine the most probable radius, which is used to find the electron in a given orbital for -orbital of hydrogen like atom having atomic number , is :
At the point maximum value of radial distribution function , one antinode is present
Most probable radius of is pm
Most probable radius of is pm
Most probable radius of hydrogen atom is pm
The correct Answer is:A, B, C, D
At the point of maximum value of RDF
where for and for the for hydrogen.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - NUCLEAR CHEMISTRYEXERCISE - PART-III5videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCECHAPTER - NUCLEAR CHEMISTRYEXERCISE - STAGE-II1videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCECHAPTER - NUCLEAR CHEMISTRYEXERCISE - ADVANCED LEVEL PROBLEMS30videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCECHAPTER - NITROGEN CONTAINING COMPOUNDSEXERCISE - ORGANIC CHEMISTRY(Nitrogen containing Compounds)30videos
NITROGEN CONTAINING COMPOUNDS
- BOOK - RESONANCECHAPTER - P BLOCK ELEMENTSEXERCISE - PART -II24videos
P BLOCK ELEMENTS
Similar Questions
Find the distance at which probability of finding electron is maximum for 1s orbital in a He atom. The wave function orbital given as.
ψ1s=4a3/20e2ra0View SolutionHydrogen Atom and Hydrogen Molecule
The observed wavelengths in the line spectrum of hydrogen atom were first expressed in terms of a series by johann Jakob Balmer, a Swiss teacher. Balmer's empirical empirical formula is
1λ=RH(122−1n2), N=3,4,5
RH=Mee48ε20h3c=109.678cm−1
Here,
RH is the Rydberg Constant, me is the mass of electron. Niels Bohr derived this expression theoretically in 1913. The formula is easily generalized to any one electron atom//ion.
Determine the loest energy and the radius of the Bohr orbit of the muonic hydrogen atom. Ignore the motion of the nucleus in your calculation. The radius of the Bohr orbit of a hydrogen atom
(called the Bohr radius,a0=ε0h2mee2∏is0.53Å)
The classical picture of an ''orbit'' in Bohr's theory has now been replaced by the quantum mechanical nation of an 'orbital'. The orbital ψ1σ1s(r) for the ground state of a hydrogen atom is given by
ψ1s(r)=1√∏a30era0
where r is the distance of the electron from the nucleus and a0 is the Bohr radius.View Solution
Knowledge Check
- Question 1 - Select One
The plots of radial distribution function for various orbitals of hydrogen atom against 'r' are given below
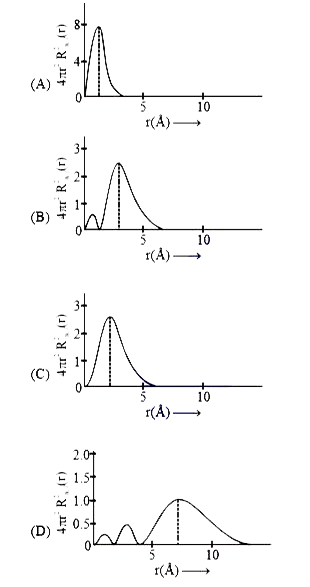
The correct plot for 3s orbital is :A(B)B(A)C(D)D(C) - Question 2 - Select One
The Schrodinger wave equation for H-atom is
∇2Ψ=8π2mh2(E−V)Ψ=0
Where ∇2=∂2∂x2+∂2∂y2+∂2∂z2
E = Total energy and V=potential energy wave function Ψ(rθϕ)R(r)Θ(θ)Φ(ϕ)
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Θ and Φ are angular wave function. R2 is known as radial probability density and 4πr2R2dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
Ψ1s=√1πaa0e−zr/a0
Psi_(2s) = ((Z)/(2a_(0)))^(½) (1-(Zr)/(a_(0)))e^(-(Zr)/(a_(0)))
The plot of radial probability function 4πr2R2 aganist r will be:
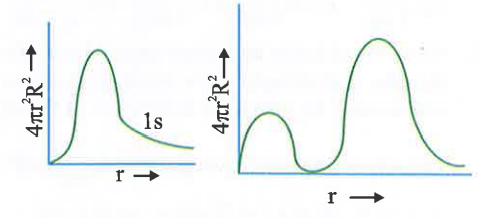
Answer the following questions:
The following graph is plotted for ns-orbitals
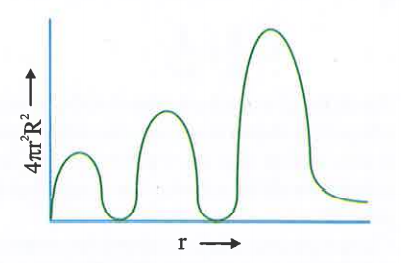
The value of 'n' will be:A1B2C3D4 - Question 3 - Select One
The Schrodinger wave equation for H-atom is
∇2Ψ=8π2mh2(E−V)Ψ=0
Where ∇2=∂2∂x2+∂2∂y2+∂2∂z2
E = Total energy and V=potential energy wave function Ψ(rθϕ)R(r)Θ(θ)Φ(ϕ)
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Θ and Φ are angular wave function. R2 is known as radial probability density and 4πr2R2dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
Ψ1s=√1πaa0e−zr/a0
Ψ2s=(Z2a0)½(1−Zra0)e−Zra0
The plot of radial probability function 4πr2R2 aganist r will be:
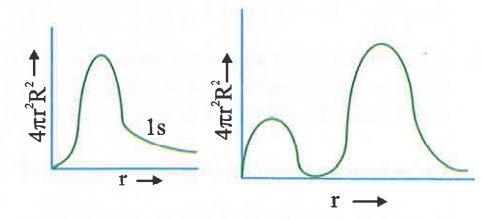
Answer the following questions:
The value of radius 'r' for 2s atomic orbital of H-atom at which the radial node will exist may be given as:Ar=2a0Br=a04Cr=a0Dr=a05
The variation of radial probability density R2 (r) as a function of distance r of the electron from the nucleus for 3p orbital:
View SolutionThe Schrodinger wave equation for H-atom is
∇2Ψ=8π2mh2(E−V)Ψ=0
Where ∇2=∂2∂x2+∂2∂y2+∂2∂z2
E = Total energy and V=potential energy wave function Ψ(rθϕ)R(r)Θ(θ)Φ(ϕ)
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Θ and Φ are angular wave function. R2 is known as radial probability density and 4πr2R2dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
Ψ1s=√1πaa0e−zr/a0
Ψ2s=(Z2a0)½(1−Zra0)e−Zra0
The plot of radial probability function 4πr2R2 aganist r will be:
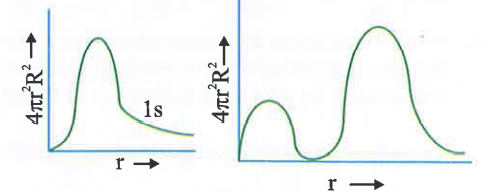
Answer the following questions:
What will be number of angular nodes and spherical nodes for 4f atomic orbitals respectively.View SolutionWhich one of the following about an electron occupying the 1s orbital in a hydrogen atom is incorrect ? (The Bohr radius is represented by a0).
View SolutionThe wave function for an orbital is H-atom is given as
ψ=(√281√π)(1a0)32(6−ra0)(ra0)er3a0.sinθsinϕ
The orbital is :View SolutionThe radius of the first orbit of the hydrogen atom is given as a0. Calculate the value of the de-Broglie wavelength of an electron revolving in the fourth orbit of a hydrogen atom ?
View Solution

