The Schrodinger wave equation for H-atom is
∇2Ψ=8π2mh2(E−V)Ψ=0
Where ∇2=∂2∂x2+∂2∂y2+∂2∂z2
E = Total energy and V=potential energy wave function Ψ(rθϕ)R(r)Θ(θ)Φ(ϕ)
R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Θ and Φ are angular wave function. R2 is known as radial probability density and 4πr2R2dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr.
Number of radial node =n -l - 1
Number of angular node = l
For hydrogen atom, wave function for 1s and 2s-orbitals are:
Ψ1s=√1πaa0e−zr/a0
Ψ2s=(Z2a0)½(1−Zra0)e−Zra0
The plot of radial probability function 4πr2R2 aganist r will be:
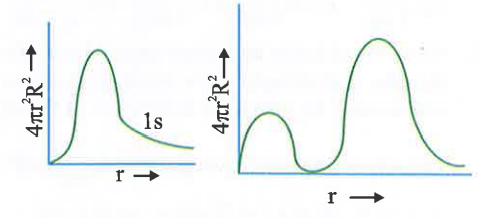
Answer the following questions:
The following graph is plotted for ns-orbitals
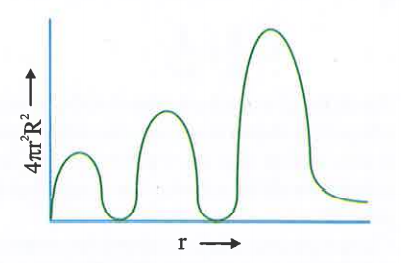
The value of 'n' will be:
1
2
3
4
The correct Answer is:C
no of peaks for given orbital in a plot is n−l
Topper's Solved these Questions
Similar Questions
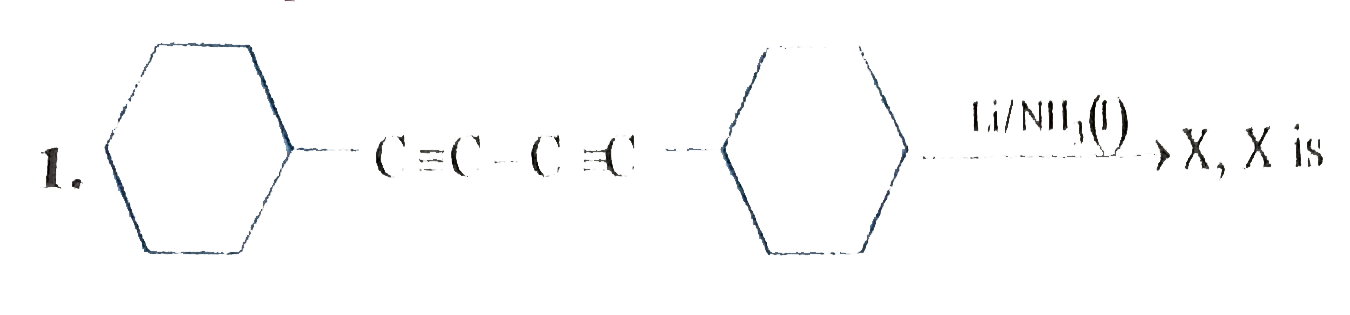
Complete the following reaction
View Solution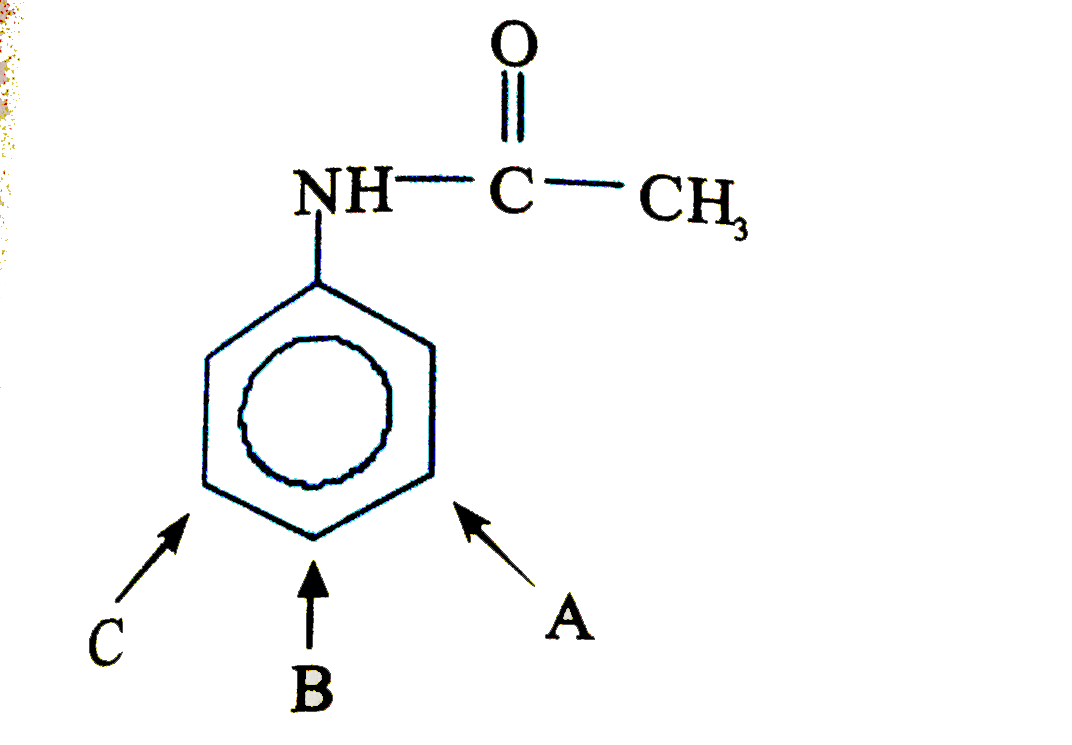
Identify the position where electrophilic aromatic substitution EAS) is most favourable
View SolutionIf x = r cos theta and y = r sin theta, prove that (del ^ (2) r) / (del x ^ (2)) + (del ^ (2) r) / (del y ^ (2)) = (1) / (r) [((del r) / (del x)) ^ (2) + ((del r) / (del y)) ^ (2)]
View SolutionOPERATOR FORM SCHRODINGER WAVE EQUATION,PLOT OF RADIAL WAVE FUNCTION 'R',PLOT OF RADIAL PROBABILITY DENSITY 'R^2
View SolutionCompare Schrodinger equation with most important equation of Newtions law.
∂2ψ∂x2+∂2ψ∂y2+∂2ψ∂z2=−(E−V)8π2mhψ Schrodinger equation....(i)
md2xdt2=Fm Newton's law ....(ii)
View SolutionFor the wave function
Ψ=√281√πa3/20[6−ra0]ra0×e−r/3a0sinθ.cosϕ
Identify the orbital .
View SolutionIn Schrodinger wave mechanical model Ψ2(r,θ,ϕ) represents :
View SolutionThe Schrodinger wave equation for H-atom is nabla^(2) Psi = (8pi^(2)m)/(h^(2)) (E-V) Psi = 0 Where nabla^(2) = (del^(2))/(delx^(2)) +(del^(2))/(dely^(2)) +(del^(2))/(delz^(2)) E = Total energy and V=potential energy wave function Psi_(((r, theta,phi)))R_((r))Theta_((theta))Phi_((phi)) R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Theta and Phi are angular wave function. R^(2) is known as radial probability density and 4pir^(2)R^(2)dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr. Number of radial node =n -l - 1 Number of angular node = l For hydrogen atom, wave function for 1s and 2s-orbitals are: Psi_(1s) = sqrt((1)/(pia_(0)^(a)))e^(-z_(r)//a_(0)) Psi_(2s) = ((Z)/(2a_(0)))^(½) (1-(Zr)/(a_(0)))e^(-(Zr)/(a_(0))) The plot of radial probability function 4pir^(2)R^(2) aganist r will be: Answer the following questions: The value of radius 'r' for 2s atomic orbital of H-atom at which the radial node will exist may be given as:
View SolutionThe Schrodinger wave equation for H-atom is nabla^(2) Psi = (8pi^(2)m)/(h^(2)) (E-V) Psi = 0 Where nabla^(2) = (del^(2))/(delx^(2)) +(del^(2))/(dely^(2)) +(del^(2))/(delz^(2)) E = Total energy and V=potential energy wave function Psi_(((r, theta,phi)))R_((r))Theta_((theta))Phi_((phi)) R is radial wave function which is function of ''r'' only, where r is the distance from nucleus. Theta and Phi are angular wave function. R^(2) is known as radial probability density and 4pir^(2)R^(2)dr is known as radial probability function i.e., the probability of finding the electron is spherical shell of thickness dr. Number of radial node =n -l - 1 Number of angular node = l For hydrogen atom, wave function for 1s and 2s-orbitals are: Psi_(1s) = sqrt((1)/(pia_(0)^(a)))e^(-z_(r)//a_(0)) Psi_(2s) = ((Z)/(2a_(0)))^(½) (1-(Zr)/(a_(0)))e^(-(Zr)/(a_(0))) The plot of radial probability function 4pir^(2)R^(2) aganist r will be: Answer the following questions: What will be number of angular nodes and spherical nodes for 4f atomic orbitals respectively.
View SolutionFor any two numbers θandϕ , we define θRϕ if and only if sec2θ−tan2ϕ=1 the relation R is -
View SolutionIn schrodinger wave equation ∇2ψ+?π2mh2(E−V)ψ=0 ? is- "
View Solution[" Which among the following "],[" statements is/are correct? "],[qquad [" For all orbitals,the radial "],[" wave function "(Psi)],[" approaches to zero as "r],[" approaches infinity "],[" The radial probability "],[" density "(psi^(2))_(" for "'1s'" orbital is ")],[" maximum at nucleus "],[" The radial distribution "],[" function "(4 pi r^(2)psi^(2))_(" for "'1s'" is ")],[" minimum at nucleus "],[" In plot of radial wave "],[" function "(psi)_(" changes its ")],[" sign at nodes "]]
View SolutionIn Schrodinger wave mechanical model Ψ2(r,θ,ϕ) represents :
View SolutionWhen Schrodinger wave equation in polar coordinates is solved the solution for Phi is of the form Psi (r, theta , phi)= R(r) , Y(theta , phi) . Here R(r) is radial part of wave function and Y(theta, phi) is angular part of the wave function. The region or space where probability of finding electron is zero is called nodal surface. If the probability of finding electron is zero then Psi^2 (r, theta, phi)=0 implies Psi (r, theta, phi)=0 If the radial wave function is equal to zero we get radial node and if angular part is equal to zero we get angular nodes. Total no. of nodes for any orbital = n - 1. Where ‘n’ is principal quantum number. The d-orbital with two nodal surfaces formed cones
View SolutionWhen Schrodinger wave equation in polar coordinates is solved the solution for Φ is of the form Ψ(r,θ,ϕ)=R(r),Y(θ,ϕ). Here R(r) is radial part of wave function and Y(θ,ϕ) is angular part of the wave function. The region or space where probability of finding electron is zero is called nodal surface. If the probability of finding electron is zero then Ψ2(r,θ,ϕ)=0⇒Ψ(r,θ,ϕ)=0
If the radial wave function is equal to zero we get radial node and if angular part is equal to zero we get angular nodes. Total no. of nodes for any orbital = n - 1. Where ‘n’ is principal quantum number.
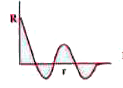 represents
represents
View Solution