In a histogram the area of each rectangle is proportional to
1)the class mark of the corresponding class interval
2)the class size of the corresponding class interval
3)frequency of the corresponding class interval
4)cumulative frequency of the corresponding class interval
To solve the question regarding the area of rectangles in a histogram, we need to analyze the relationship between the area of each rectangle and the corresponding class interval.
1. Understanding Histogram Basics:
- A histogram is a graphical representation of the distribution of numerical data. It consists of bars (rectangles) where the height of each bar represents the frequency of data within a particular range (class interval).
Hint: Remember that the height of each bar in a histogram corresponds to the frequency of the data in that interval.
2. Identifying Axes:
- In a histogram, the x-axis typically represents the class intervals, while the y-axis represents the frequency of the data points that fall within those intervals.
Hint: The x-axis shows the ranges of data, and the y-axis shows how many data points fall into those ranges.
3. Calculating Area of Rectangles:
- The area of each rectangle in a histogram is calculated by multiplying the height (frequency) of the rectangle by its width (class size). Therefore, the area of each rectangle is proportional to the frequency of the corresponding class interval.
Hint: Area = Height (Frequency) × Width (Class Size).
4. Analyzing Options:
- Now, let's analyze the options given in the question:
1) Class mark of the corresponding class interval - This is not correct.
2) Class size of the corresponding class interval - This is not correct.
3) Frequency of the corresponding class interval - This is correct.
4) Cumulative frequency of the corresponding class interval - This is not correct.
Hint: Focus on the definition of area in terms of frequency and class size.
5. Conclusion:
- From the analysis, we conclude that the area of each rectangle in a histogram is proportional to the frequency of the corresponding class interval.
Final Answer: The correct answer is 3) frequency of the corresponding class interval.
- A histogram is a graphical representation of the distribution of numerical data. It consists of bars (rectangles) where the height of each bar represents the frequency of data within a particular range (class interval).
- In a histogram, the x-axis typically represents the class intervals, while the y-axis represents the frequency of the data points that fall within those intervals.
- The area of each rectangle in a histogram is calculated by multiplying the height (frequency) of the rectangle by its width (class size). Therefore, the area of each rectangle is proportional to the frequency of the corresponding class interval.
- Now, let's analyze the options given in the question:
1) Class mark of the corresponding class interval - This is not correct.
2) Class size of the corresponding class interval - This is not correct.
3) Frequency of the corresponding class interval - This is correct.
4) Cumulative frequency of the corresponding class interval - This is not correct.
- From the analysis, we conclude that the area of each rectangle in a histogram is proportional to the frequency of the corresponding class interval.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Write the comulative frequency of class interval 30-40
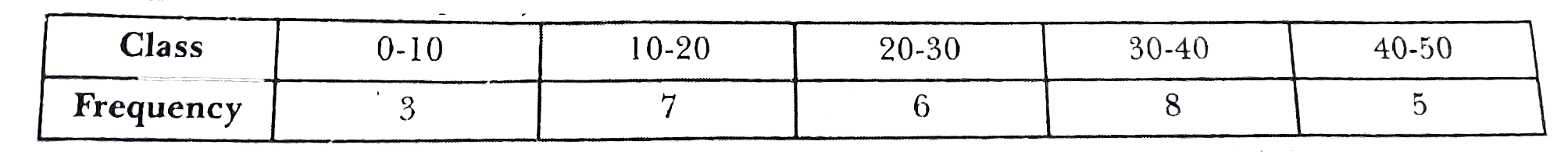 A21B24C28D8
A21B24C28D8If a class mark of a class-interval 8.5. The class size is 5,then the class limits of the corresponding class-interval is
A6.5-11.5B6−11C5.5−10.5D7−12The mid point of class interval is called
AClass IntervalBFrequencyCMid value,DNone of these
Classes or Class Intervals
View SolutionThe class mark of the class interval 4.7-6.3 is _______.
View SolutionUnequal Class Intervals
View SolutionIn whilch graph the breadth of rectangle is equal to the length of the class interval?
View SolutionThe mid-value of a class interval is 25 and the class size is 8. The class interval is
View Solution
