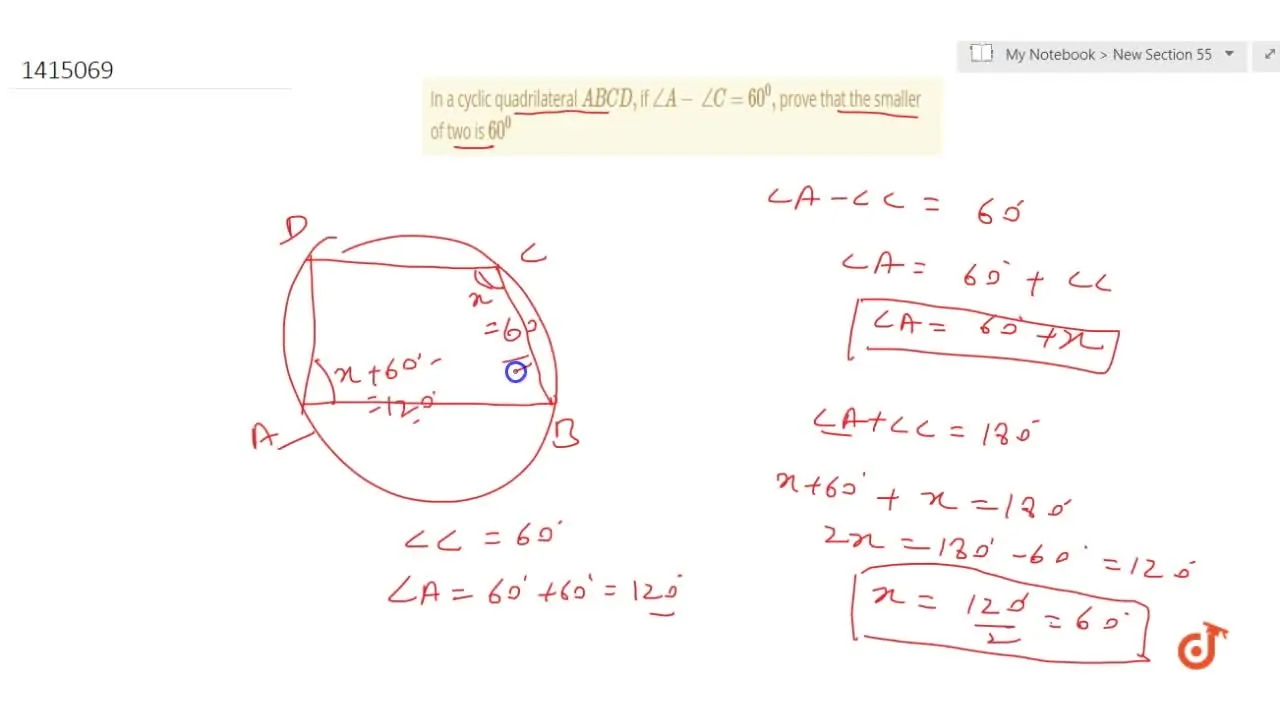
In a cyclic quadrilateral $A B C D,$ if $∠ A - ∠ C = 60^{0},$ prove that the smaller of two is $60^{0}$

Video Solution

Text Solution

Verified by Experts

Here, $A B C D$ is a cyclic quadrilateral.
We know, in a cyclic quadrilateral, the sum of opposite angles are supplementary, i.e. $180^{0}$
Then, $∠ A + ∠ C = 180^{0}$
But given, $∠ A - ∠ C = 60^{0}$
Adding the two equations, $2 ∠ A = 240 \land 0$
$∠ A = 120^{0}$
Therefore, $∠ A + ∠ C = 180^{0}$
$120^{0} + ∠ C = 180^{0}$
$∠ C = 60^{0}$
The smaller one is $60^{0}$ .

Updated on:21/07/2023

In a cyclic quadrilateral $A B C D ∠ A + ∠ B + ∠ C + ∠ D = ?$
AA) $90^{\circ}$
BB) $360^{\circ}$
CC) $180^{\circ}$
DD) $120^{\circ}$
If ABCD is a cyclic quadrilateral with $∠ A = 50^{\circ}, ∠ B = 80^{\circ}$ , then $C and ∠ D$ are
A $100^{\circ}, 130^{\circ}$
B $115^{\circ}, 115^{\circ}$
C $110^{\circ}, 120^{\circ}$
D $130^{\circ}, 100^{\circ}$
If A,B,C,D are the angles of a cyclic quadrilateral and cos A+cos B= K(cos C + cos D), then the value of K is
A1
B $- 1$
C2
D $- 2$