A closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as $\to μ = - \to I A$ where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field $\to B$ , then torque acting on the loop is given by $\to τ = \to μ \times \to B$ . Now answer the following questions:
Consider the situation shown in Fig., ring is having a uniformly distributed positive charge. Magnetic field is perpendicular to the axis of ring. Now ring is rotated in anticlockwise direction as seen from left hand side direction of magnetic torque acting on the ring is

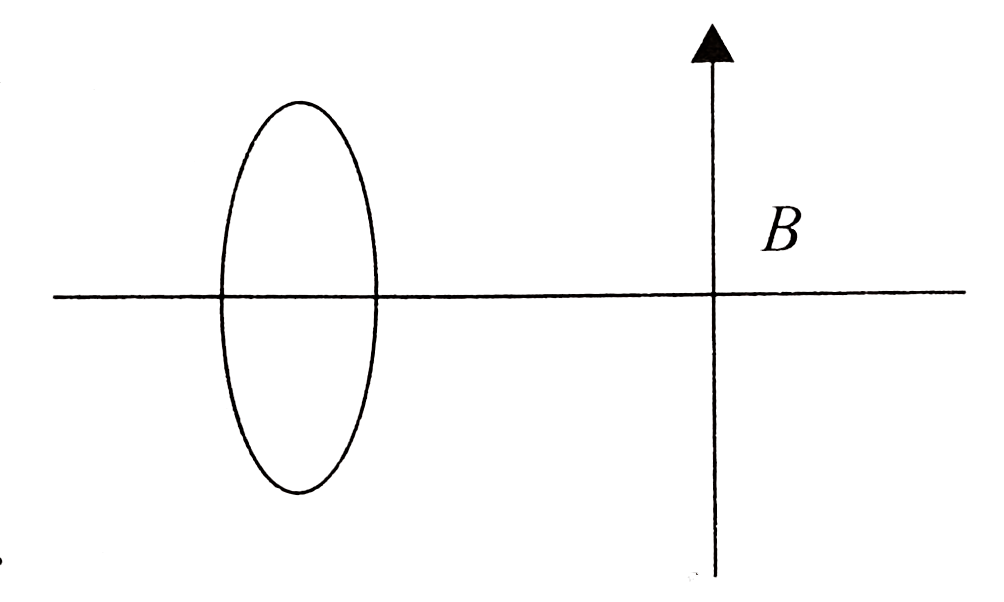
Parallel to

Parallel to axis of ring

perpendicular to axis and magnitic field

Coming out of plane of paper

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$\to τ$ is perpendicular $\to M$ and $\to B$

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Knowledge Check

A closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as $\to μ = - \to I A$ where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field $\to B$ , then torque acting on the loop is given by $\to τ = \to μ \times \to B$ . Now answer the following questions:
A uniformly charged insulating ring is rotated in a uniform magnetic field about its own axis, then
ARing will experience a magnetic force
BRing must experience a magnetic torque
CRing may experience a magnetic torque
DNone of the above
A closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as $\to μ = - \to I A$ where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field $\to B$ , then torque acting on the loop is given by $\to τ = \to μ \times \to B$ . Now answer the following questions:
Let ring in the above question is having a radius R and a charge Q is uniformly distributed over it. Ring is rotated with a constant angular velocity $(ω)$ as mentioned above.
Torque acting on the ring due to magnetic force is
A $\frac{Q R^{2} ω B}{2}$
B $\frac{Q R^{2} ω B}{2 ω}$
C $\frac{ω R^{2} B}{2 π}$
DNone of the above
A dipole of dipole moment $\to P$ is placed in uniform electric field $\to E$ , then torque acting on it is given by
A $\to τ = \to P . \to E$
B $\to τ = \to P \times \to E$
C $\to τ = \to P + \to E$
D $\to τ = \to P - \to E$