A mass 'm' moves with a velocity 'v' and collides inelastieally with another identical mass . After collision the 1st mass moves with velocity v√3 in a direction perpendicular to the initial direction of motion. Find the speed of the 2nd mass after collision.
⋅→mbeforecollision⋅m↑⏐
⏐
⏐
⏐⏐v/√3aftercollision⟩
2v√3
v√3
v
√3v
The correct Answer is:A
In the collision , the momentum is conserved. Before the collision momentum is along the x-axis , after the collision , the resultant momentum should be along the x-axis , for this the second particle should move at some angle with the x-axis. Here , the momentum should be conserved in the x-axis and y-directions separately.
Momentum conservation :
x-axis : mv = mV cos theta rArr V cos theta = v<br>y−axis:0=mv√3−mVsinθ⇒Vsinθ=v√3
V=√(Vsinθ)2+(Vcosθ)2=2v√3
Topper's Solved these Questions
Similar Questions
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA particle of mass m moving with velocity u collides elastically head on with a particle of mass 2m at rest. After collision the heavier particle moves with velocity?
View Solution
Knowledge Check
A mass m moves with a velocity v and collides inelastically with another identical mass. After collision the 1st mass moves with velocity v√3 in a direction perpendicular to the initial direction of motion. Find the speed of the second mass after collision
AvB√3vC2√3vDv√3A mass ‘m’ moves with a velocity ‘v’ and collides inelastically with another identical mass at rest. After collision the 1st mass moves with velocity v√3 in a direction perpendicular to the initial direction of motion. Find the speed of the 2nd mass after collision :
AvB√3vC2√3 vDv√3A mass m moves with a velocity v and collides inelastically with another identical mass at rest. After collision, the first mass moves with velocity v√3 in a direction perpendicular to Before collision the initial direction of motion. The speed of the 2nd mass after collision
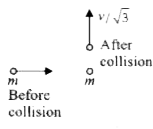 A2v√3Bv√3CvD√3v
A2v√3Bv√3CvD√3v
A sphere of mass m moves with a velocity 2v and collides inelastically with another identical sphere of mass m. After collision the first mass moves with velocity v in a direction perpendicular to the initial direction of motion . Find the speed of the second sphere after collision .
View SolutionA ball of mass M moving with a velocity v collides perfectly inelastically with another ball of same mass but moving with a velocity v in the opposite direction. After collision
View SolutionA ball of mass m moving with velocity v collides elastically with another ball of identical mass coming from the opposite direction with velocity 2v. Their velocities after collisions are-
View SolutionA ball of mass m moving with a velocity v undergoes an oblique elastic collision with another ball of the same mass m but at rest. After the collision if the two balls move with the same speeds , the angle between their directions of motion will be:
View SolutionA smooth sphere of mass M moving with velocity u directly collides elastically with another sphere of mass m at rest. After collision their final velocities are V and v respectively. The value of v is
View Solution