Three identical metal balls each of radius r are placed touching each other on a horizontal surface such that an equilateral triangle is formed, when the center of three balls are joined. The center of mass of system is located at the
horizontal surface
center one of the balls
line joining centers of any two balls
point of intersection of medians
The correct Answer is:D
To solve the problem of finding the center of mass of three identical metal balls arranged in an equilateral triangle, we will follow these steps:
Step 1: Understand the Arrangement
We have three identical metal balls, each with a radius r. They are arranged in such a way that they touch each other, forming the vertices of an equilateral triangle. The centers of the balls will also form the vertices of this triangle.
Step 2: Determine the Coordinates of the Centers
Let’s assign coordinates to the centers of the balls:
- Ball 1 (C1) at (0, 0)
- Ball 2 (C2) at (2r, 0) (since the distance between the centers of two touching balls is 2r)
- Ball 3 (C3) at (r, r√3) (the third vertex of the equilateral triangle)
Step 3: Calculate the Center of Mass
The center of mass (CM) of a system of particles can be calculated using the formula:
\(
CM_x = \frac{x_1 + x_2 + x_3}{3}
\)
\(
CM_y = \frac{y_1 + y_2 + y_3}{3}
\)
Substituting the coordinates:
- For x-coordinates:
\(
CM_x = \frac{0 + 2r + r}{3} = \frac{3r}{3} = r
\)
- For y-coordinates:
\(
CM_y = \frac{0 + 0 + r\sqrt{3}}{3} = \frac{r\sqrt{3}}{3}
\)
Step 4: Conclusion
Thus, the center of mass of the system of three identical metal balls is located at the point:
\(
\left( r, \frac{r\sqrt{3}}{3} \right)
\)
We have three identical metal balls, each with a radius r. They are arranged in such a way that they touch each other, forming the vertices of an equilateral triangle. The centers of the balls will also form the vertices of this triangle.
Let’s assign coordinates to the centers of the balls:
- Ball 1 (C1) at (0, 0)
- Ball 2 (C2) at (2r, 0) (since the distance between the centers of two touching balls is 2r)
- Ball 3 (C3) at (r, r√3) (the third vertex of the equilateral triangle)
The center of mass (CM) of a system of particles can be calculated using the formula:
\(
CM_x = \frac{x_1 + x_2 + x_3}{3}
\)
\(
CM_y = \frac{y_1 + y_2 + y_3}{3}
\)
- For x-coordinates:
\(
CM_x = \frac{0 + 2r + r}{3} = \frac{3r}{3} = r
\)
\(
CM_y = \frac{0 + 0 + r\sqrt{3}}{3} = \frac{r\sqrt{3}}{3}
\)
Thus, the center of mass of the system of three identical metal balls is located at the point:
\(
\left( r, \frac{r\sqrt{3}}{3} \right)
\)
Topper's Solved these Questions
Similar Questions
Three thin unifrom circular discs each of mass M and radius R are placed touching each other on a horizontal surface such that an equilateral triangle is formed, when the center of three discs are joined. Find the M.I. about an axis passing through the center of mass system of discs and perpendicular to the plane.
View SolutionThree identical spheres each of radius 'R' are placed on a horizontal surface touching one another. If one of the spheres is removed, the shift in the centre of mass of the system is.
View Solution
Knowledge Check
Three identical metal balls, each of the radius r are placed touching each other on a horizontal surface such that an equilateral triangle is formed when centres of three balls are joined. The centre ofthe mass of the system is located at
Aline joining centres of any two ballsBcentre of one of the ballsChorizontal surfaceDhorizontal surfaceThree identical balls each of radius 10 cm and mass 1 kg each are placed touching one another on a horizontal surface. Where is their centre of mass located?
AOn the horizontal surfaceBAt the point of contact of any two spheresCAt the centre of one ballDnone of theseThree identical spheres each of radius R are placed touching each other on a horizontal table as shown in figure. The co-ordinates of centre of mass are :
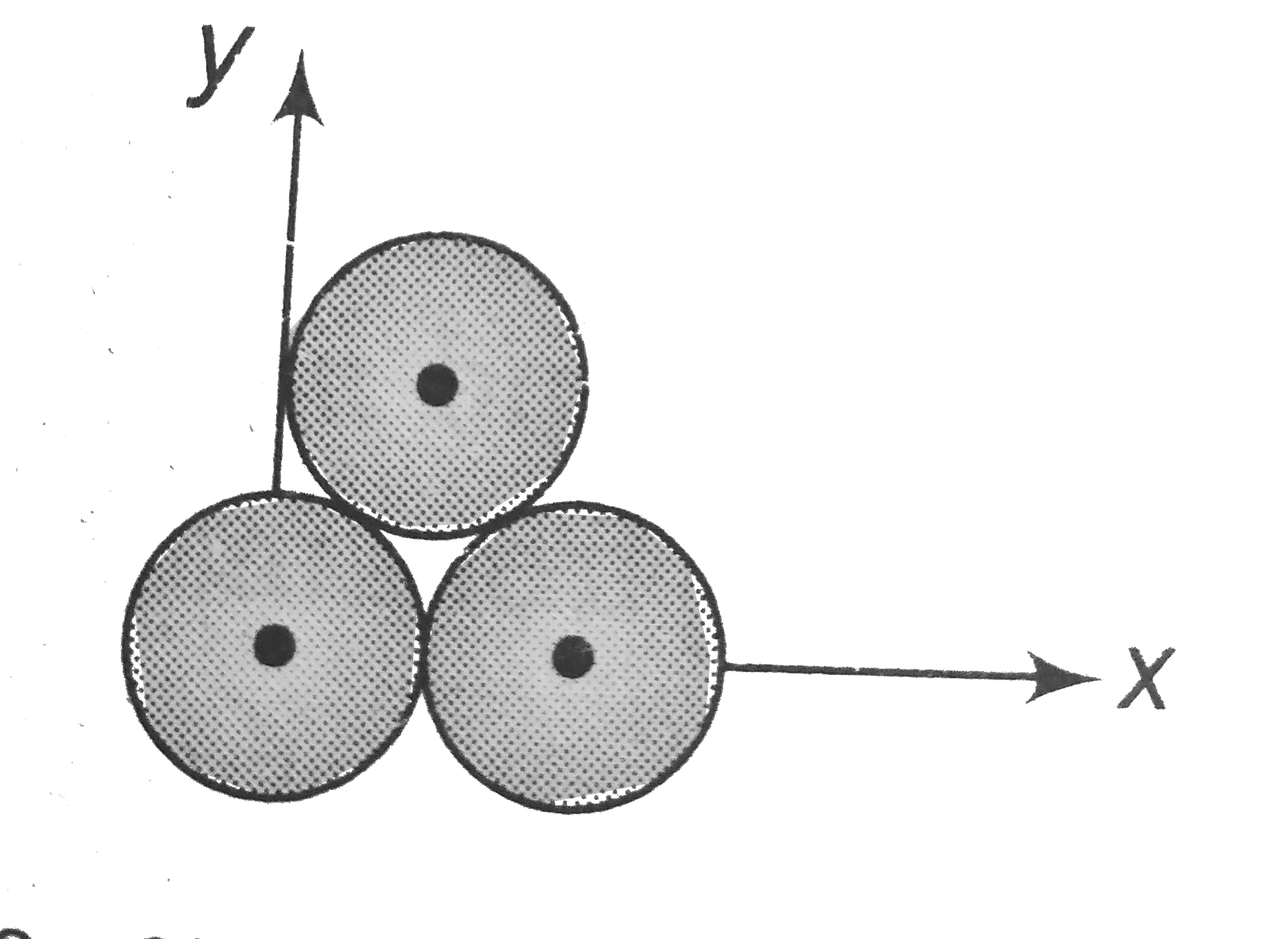 .A(R,R)B(0,0)C(R2,R2)D(R,R√3)
.A(R,R)B(0,0)C(R2,R2)D(R,R√3)
Three identical spheres each of radius 3cm are placed on a horizontal surface touching one another. The distance of centre of mass of system from any centre of the sphere is
View SolutionTwo balls of mass m each are placed at the two vertices of an equilateral triangle. Another ball of mass 2m is placed at the third vertex of the triangle. Locate the centre of mass of the system.
View SolutionThree identical spheres each of mass m and radius R are placed touching each other so that their centres A,B and C lie on a straight line. The position of their centre of mass from centre of A is
View SolutionThree identical masses, each of mass 1kg, are placed at the corners of an equilateral triangle of side l. Then the moment of inertia of this system about an axis along one side of the triangle is
View SolutionThree identical bar magnets each of magnetic moment M are placed in the form of an equilateral triangle as shown.

View Solution
