At time t=0, the position vector of a particle moving in the x−y plane is 5ˆjm.By time t=0.62sec,its position vector has become (5.1ˆi+0.4ˆj)m.with the data answer the following questions.
The angle θ made by the average velocity with the positive x axis
tan−1(2)
tan−1(3)
tan−1(1)
tan−1(4)
The correct Answer is:D
x=at⇒Vx=a⇒ax=0
y=at(1−at)⇒Vy=a−a2αt⇒ay=−2αa
Again y=axa(1−xa.α)⇒y=x−x2αa
If →V and →a makes an angle 45∘ with each other →V must be making an angle 45∘ with X-axis
So Vx=Vy⇒a=a−2aαt0⇒t0=1α
Displacement −−→Δx=(.1ˆi+4ˆj)m
→Vavg= Average velocity
(.1ˆi+.4ˆj.02)m/sec=(5ˆi+20ˆj)m/sec
So ∣∣→Vavg∣∣=√425m/sec=20.6m
θ=tan−1205=tan−14
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-Vi Integer
9 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-I (H.W)
41 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-Vi multiple answer
16 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
Similar Questions
In a particle accelerator, the position vector of a particle is initially estimated as →r=6.0ˆi−7.0ˆj+3.0ˆk and after 10 s, it is estimated to be →r=−3.0ˆi+9.0ˆj−3.0ˆk, all in meters. In unit vector notation, what is the average velocity of the particle?
View SolutionThe position vector of a particle at time t is given by →r=2tˆi+5tˆj+4sinωtˆk where ω is a constant.Answer the following questions
Velocity vector is perpendicular to …….vector
View Solution
Knowledge Check
At time t=0, the position vector of a particle moving in the x−y plane is 5ˆjm.By time t=0.62sec,its position vector has become (5.1ˆi+0.4ˆj)m.with the data answer the following questions.
The magnitude of the average velocity during the above time interval.A.0206m/secB0.206m/secC20.6m/secD2.06m/secPosition vectors of a particle moving in xy plane at time t is →r=a(1−cosomηt)ˆi+asinωtˆj. The path of the particle is
Aa circle of radius a and center at (a,0)Ba circle of radius a and center at (0,0)Can ellipseDneither a circle nor an ellipseThe position vector of a particle is r=asinωtˆi+acosωtˆj
The velocity of the particle isAparallel to position vecorBperpendicular to position vectorCdirected towards originDdirected awa from the origin
The displacement of a particle from a point having position vector 2ˆi+4ˆj to another point having position vector 5ˆj+1ˆj is
View SolutionVx is the velocity of a particle of a particle moving along the x−axis as shown. If x=2.0m at t=1.0s, what is the position of the particle at t=6.0s?

View Solutionvx is the velocity of a particle moving along the x-axis as shown in the figure. If x=2.0m at t=1.0s, what is the position of the particle at t=6.0s ?
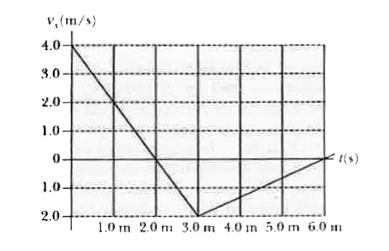
View SolutionA particle moves in x-y plane. The position vector of particle at any time t is →r={(2t)ˆi+(2t2)ˆj} m. The rate of change of θ at time t = 2 s (where θ is the angle which its velocity vector makes with positive x-axis) is
View SolutionThe position vector of a particle at time t is given by →r=2tˆi+5tˆj+4sinωtˆk where ω is a constant.Answer the following questions
Velocity vector of the particle is
View Solution