A man is riding on a flat car travelling with a constant speed of 10m/s.He wishes to throw a ball through a stationary hoop 15m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5m/s w.r.t himself.
At what horizontal distance in front of the hoop must he release the ball?
12.5m
15.5m
17.5m
20m
The correct Answer is:C
To solve the problem, we need to determine the horizontal distance in front of the hoop where the man must release the ball so that it passes through the hoop horizontally.
1. Identify the Given Information:
- Speed of the car (v_car) = 10 m/s
- Speed of the ball with respect to the man (v_ball) = 12.5 m/s
- Height of the hoop above the man's hands (h) = 15 m
- The ball must pass through the hoop horizontally.
2. Determine the Vertical Motion:
- The ball is thrown at an angle θ with respect to the horizontal.
- The vertical component of the ball's velocity (v_vertical) is given by:
vvertical=vball⋅sin(θ)
- The horizontal component of the ball's velocity (v_horizontal) is given by:
vhorizontal=vball⋅cos(θ)+vcar
3. Use the Vertical Motion Equation:
- Since the ball passes through the hoop horizontally, its vertical velocity at that point is zero.
- Using the kinematic equation for vertical motion:
h=vvertical⋅t−12gt2
- Here, g is the acceleration due to gravity (approximately 9.81 m/s²).
4. Calculate Time to Reach the Hoop:
- The ball must fall a vertical distance of 15 m, so we set up the equation:
15=vball⋅sin(θ)⋅t−12gt2
- Rearranging gives us a quadratic equation in terms of t:
12gt2−vball⋅sin(θ)⋅t+15=0
5. Solve for Time (t):
- Using the quadratic formula t=−b±√b2−4ac2a, we can find t.
6. Calculate Horizontal Distance:
- The horizontal distance (d) traveled by the ball when it reaches the hoop is given by:
d=vhorizontal⋅t
- Substitute vhorizontal=vball⋅cos(θ)+vcar into the equation.
7. Determine the Angle θ:
- From the vertical motion, we can find sin(θ) and cos(θ) using trigonometric identities based on the relationship between the vertical and horizontal components.
8. Final Calculation:
- Substitute the values into the equations to find the horizontal distance in front of the hoop.
- Speed of the car (v_car) = 10 m/s
- Speed of the ball with respect to the man (v_ball) = 12.5 m/s
- Height of the hoop above the man's hands (h) = 15 m
- The ball must pass through the hoop horizontally.
- The ball is thrown at an angle θ with respect to the horizontal.
- The vertical component of the ball's velocity (v_vertical) is given by:
vvertical=vball⋅sin(θ)
- The horizontal component of the ball's velocity (v_horizontal) is given by:
vhorizontal=vball⋅cos(θ)+vcar
- Since the ball passes through the hoop horizontally, its vertical velocity at that point is zero.
- Using the kinematic equation for vertical motion:
h=vvertical⋅t−12gt2
- Here, g is the acceleration due to gravity (approximately 9.81 m/s²).
- The ball must fall a vertical distance of 15 m, so we set up the equation:
15=vball⋅sin(θ)⋅t−12gt2
- Rearranging gives us a quadratic equation in terms of t:
12gt2−vball⋅sin(θ)⋅t+15=0
- Using the quadratic formula t=−b±√b2−4ac2a, we can find t.
- The horizontal distance (d) traveled by the ball when it reaches the hoop is given by:
d=vhorizontal⋅t
- Substitute vhorizontal=vball⋅cos(θ)+vcar into the equation.
- From the vertical motion, we can find sin(θ) and cos(θ) using trigonometric identities based on the relationship between the vertical and horizontal components.
- Substitute the values into the equations to find the horizontal distance in front of the hoop.
Topper's Solved these Questions
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-Vi Integer
Explore 9 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-I (H.W)
Explore 41 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-Vi multiple answer
Explore 16 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NARAYNAChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:LEVEL-II (H.W)
Explore 24 Videos
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Level 2 H.W
Explore 29 Videos
Similar Questions
A man is standing on a rail road car travelling with a constant speed of v=10ms−1 (Fig . 5.34). He wishes to throw a ball through a stationary hoop 5m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5ms−1 w.r.t. himself.
(a) What must be the vertical component of the initial velocity of the ball ?
(b) How many seconds after he releases the ball will it pass through the hoop ?
( c) At what horizontal distance in front of the loop must he release the ball ?
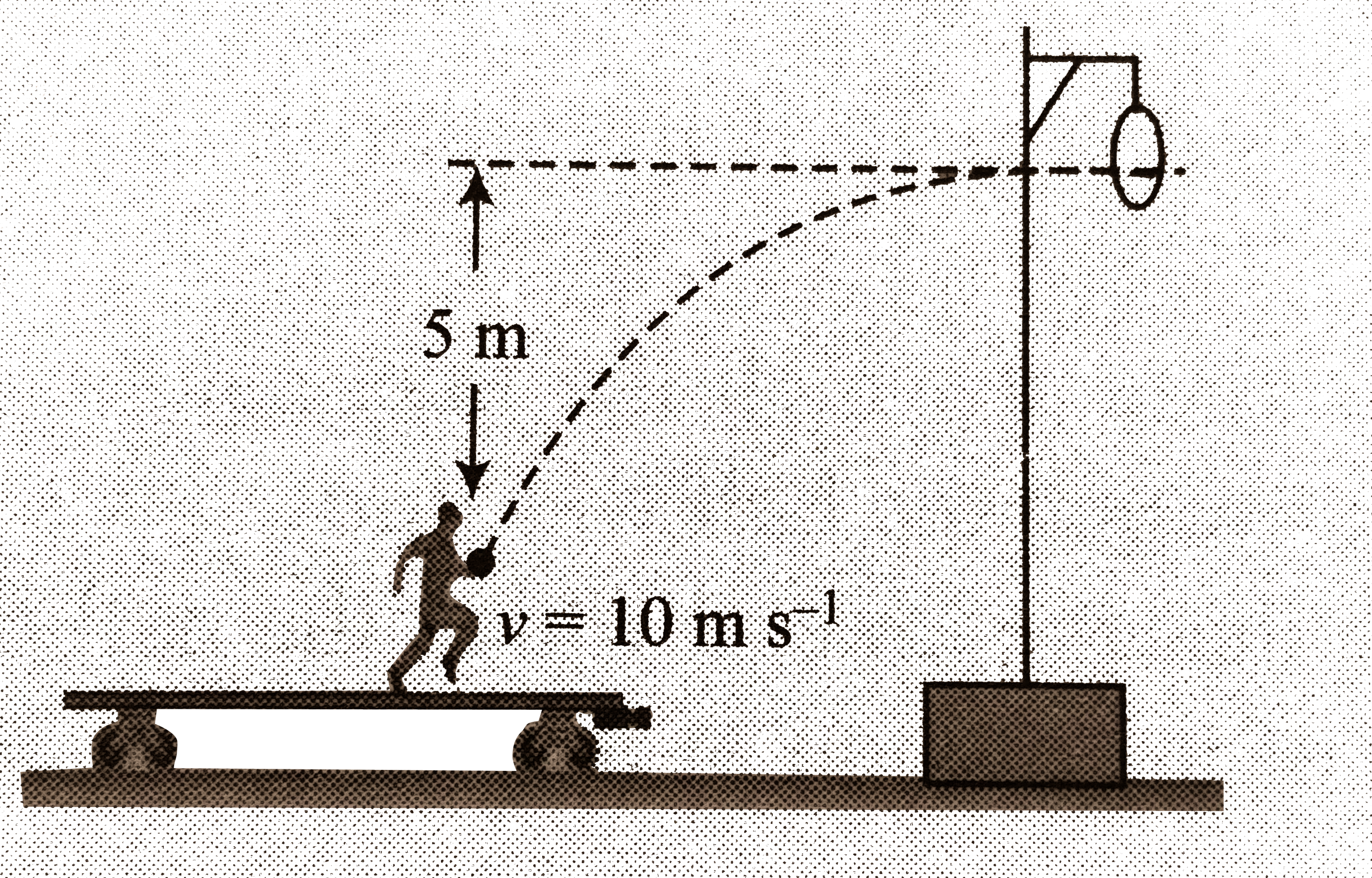 .
.
View SolutionA man thrown a ball making an angle of 60∘ with the horizontal.He runs on a level ground with constant velocity and catches the ball at the same horizontal level as it was thrown.If he had thrown the ball with speed 4m/s, then speed of running must be ?(in m/s)
View Solution
Knowledge Check
A man is riding on a flat car travelling with a constant speed of 10m/s.He wishes to throw a ball through a stationary hoop 15m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5m/s w.r.t himself.
How many seconds after he release the ball will it pass through the hoop?A1secB2secC3secD4secA ball is thrown horizontally with a speed of 20m/s from the top of a tower of height 100m
Horizontal distance travelled by the before it strickes the ground isA10√5mB20√5mC30√5mD40√5mA ball is thrown horizontally with speed 10m/s from the top of tower of height 80m. After how much time and at what horizontal distance from the tower it strikes the ground ?
A2s,40mB2s,20mC4s,40mD4s,80m
There is a tall cylindrical building standing in a field. Radius of the cylinder is R=8m. A boy standing at A (at a distance of 10 m from the centre of the cylindrical base of the building) knows that his friend is standing at B behind the building. The line joining A and B passes through the centre of the base of the building. Distance between A and B is 50 m. A wants to throw a ball to B but he realizes that the building is too tall and he cannot throw the ball over it. He throws the ball at a speed of 20m/s such that his friend at B has to move minimum distance to catch it.
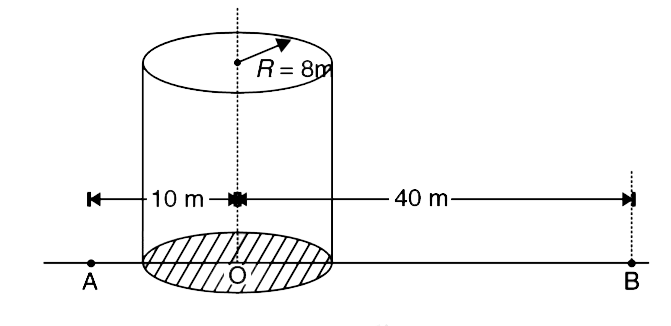
(a) What is the minimum distance that boy at B will have to move to catch the ball?
(b) At what angle to the horizontal does the boy at A throws the ball? Assume that the ball is released and caught at same height above the ground.
[Take g=10m/s2 and sin−1(0.75)≅48.6∘
View SolutionA cricketer can throw a ball to a maximum horizontal distance of 100 m. How high above the ground can the cricketer throw the ball, with the same speed ?
View SolutionA man can throw a stone with initial speed of 10m/s. Find the maximum horizontal distance to which he can throw the stone in a room of height h m for:
(i) h=2m and (ii) h=4m.
View SolutionA boy is standing inside a train moving with a constant velocity of magnitude 10 m/s. He throws a ball vertically up with a speed 5 m/s relative to the train. Find the radius of curvature of the path of the L ball just at the time of projection.
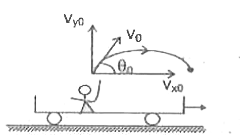
View SolutionA boy throws a ball in air in such a manner that when the ball is at its maximum height he throws another ball. If the balls are thrown after the time difference of 1 s, then what will be the height attained by them?
View Solution