Two forces F1 and F2 are acting at a point, whose resultant is F.If F2 is doubled F is also doubled. If F2 is reversed then also F is doubled. Then F1:F2:F is
√2:√2:√3
√3:√3:√2
√3:√2:√3
√2:√3:√2
The correct Answer is:D
To solve the problem, we need to analyze the conditions given and derive the relationship between the forces F1, F2, and the resultant force F.
1. Understanding the Forces:
- Let F1 and F2 be two forces acting at a point, and their resultant is F.
- We can represent the resultant force using the parallelogram law of vector addition.
2. First Condition: When F2 is doubled:
- The new force F′2=2F2.
- The resultant becomes F′=F1+2F2.
- According to the problem, this resultant is also double the original resultant: F′=2F.
- Therefore, we can write the equation:
F1+2F2=2F(1)
3. Second Condition: When F2 is reversed:
- The new force F′′2=−F2.
- The resultant becomes F′′=F1−F2.
- Again, this resultant is double the original resultant: F′′=2F.
- Thus, we can write the equation:
F1−F2=2F(2)
4. Setting Up the Equations:
- From equation (1):
F1+2F2=2F(1)
- From equation (2):
F1−F2=2F(2)
5. Solving the Equations:
- We can solve these two equations simultaneously.
- From equation (2), we can express F1:
F1=2F+F2(3)
- Substitute equation (3) into equation (1):
(2F+F2)+2F2=2F
2F+3F2=2F
3F2=0⟹F2=0
- Substitute F2=0 back into equation (3):
F1=2F+0=2F
6. Finding the Ratios:
- Now we have F1=2F and F2=0.
- The ratio F1:F2:F becomes:
2F:0:F
- This can be simplified to:
2:0:1
Final Result:
The ratio F1:F2:F is 2:0:1.
- Let F1 and F2 be two forces acting at a point, and their resultant is F.
- We can represent the resultant force using the parallelogram law of vector addition.
- The new force F′2=2F2.
- The resultant becomes F′=F1+2F2.
- According to the problem, this resultant is also double the original resultant: F′=2F.
- Therefore, we can write the equation:
F1+2F2=2F(1)
- The new force F′′2=−F2.
- The resultant becomes F′′=F1−F2.
- Again, this resultant is double the original resultant: F′′=2F.
- Thus, we can write the equation:
F1−F2=2F(2)
- From equation (1):
F1+2F2=2F(1)
- From equation (2):
F1−F2=2F(2)
- We can solve these two equations simultaneously.
- From equation (2), we can express F1:
F1=2F+F2(3)
- Substitute equation (3) into equation (1):
(2F+F2)+2F2=2F
2F+3F2=2F
3F2=0⟹F2=0
- Substitute F2=0 back into equation (3):
F1=2F+0=2F
- Now we have F1=2F and F2=0.
- The ratio F1:F2:F becomes:
2F:0:F
- This can be simplified to:
2:0:1
The ratio F1:F2:F is 2:0:1.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - NCERT BASED QUES. SINGLE ANS.
8 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - NCERT BASED QUES. MORE THAN ONE OPTION
6 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(C.W)
45 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
Similar Questions
Knowledge Check
Two forces F1 and F2 are acting on a rod abc as shown in figure.
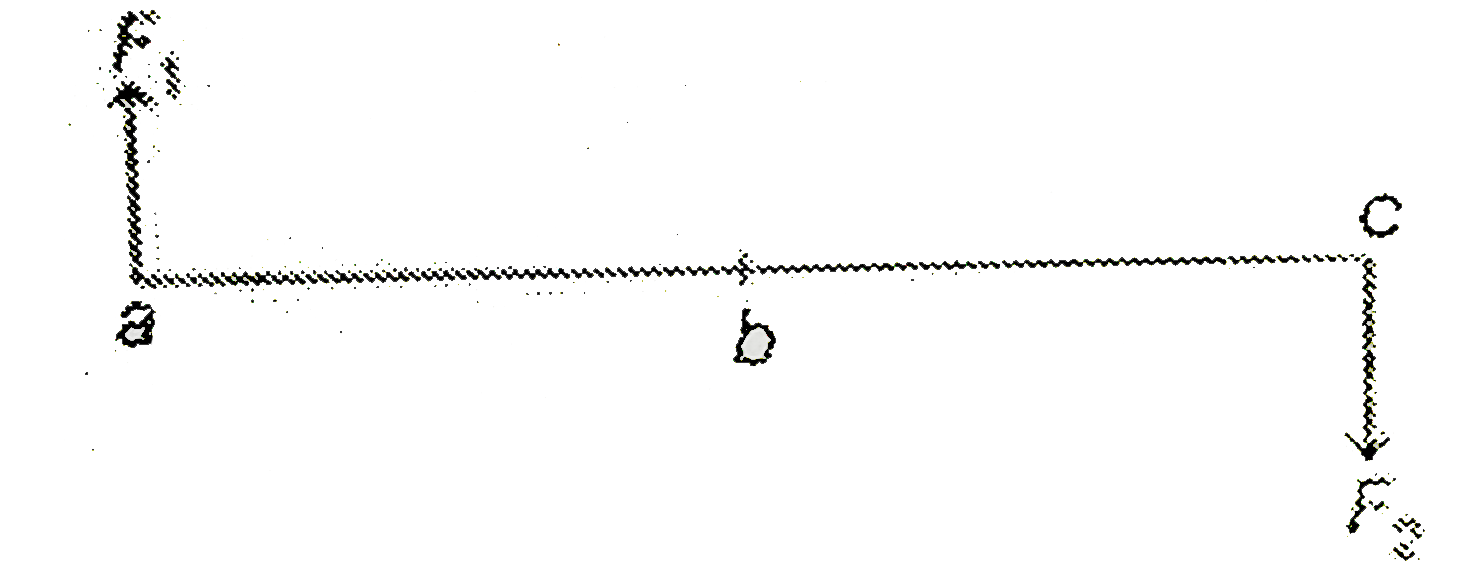 Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)
Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)Two forces −→F1and−→F2 are acting at right angles to each other , find their resultant ?
A√F21−F22B√F21+F22C√(F1+F2)DF1+F2Two forces of mangitude F1 and F2 are acting on an object.The magnitude of the net force Fnet on the object will be in the range.
AF1≤Fnet≤F2B|F1−F2|/2≤Fnet≤(F1+F2)/2C|F1−F2|≤Fnet≤F1+F2D|F1−F2|≥Fnet|F1+F2|
Two forces F1 and F2 are acting on a body. One force is double that of the other force and the resultant is equal to the greater force. Then the angle between the two forces is
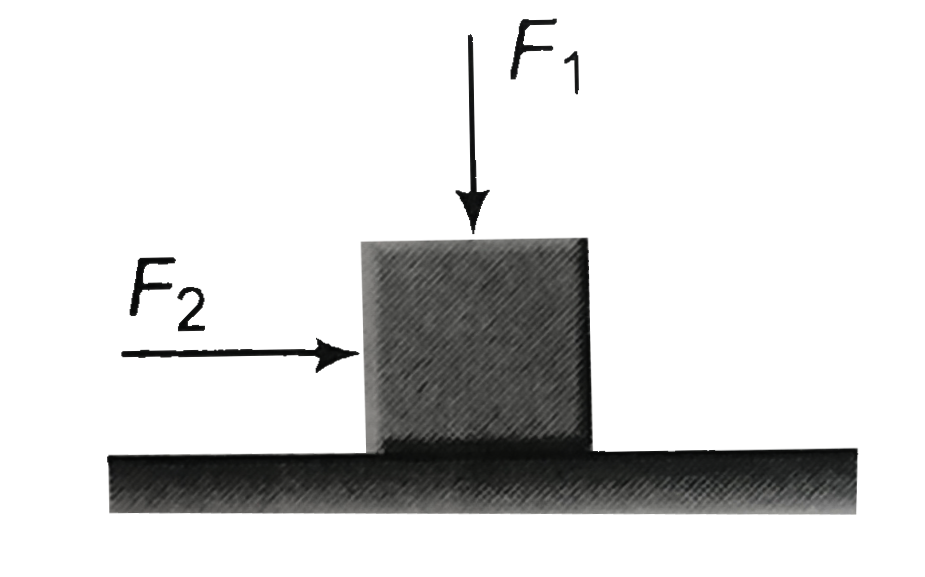
View SolutionA block is kept at rest on a rought ground as shown. Two force F1and F2 are acting on it. If we increased either of the two force F1and F2, force of friction acting on the block will increase.
By increasing F1, normal reaction from ground will increase.
View SolutionTwo force F1 and F2 are acting on a body. One force is double that of the other force and the resultant is equal to the greater force. Then the angle between the two forces is :-
View SolutionForces F1andF2 act on a point mass in two mutually perpendicular directions. The resultant force on the point mass will be
View SolutionWhen forces F1 , F2 , F3 are acting on a particle of mass m such that F2 and F3 are mutually prependicular, then the particle remains stationary. If the force F1 is now rejmoved then the acceleration of the particle is
View Solution
