A particle of mass 1kg is moving X-axis. Its velocity is 6m/s at x=0. Acceleration-displacement curve and potential energy-dispalcement curve of the particle are shown :
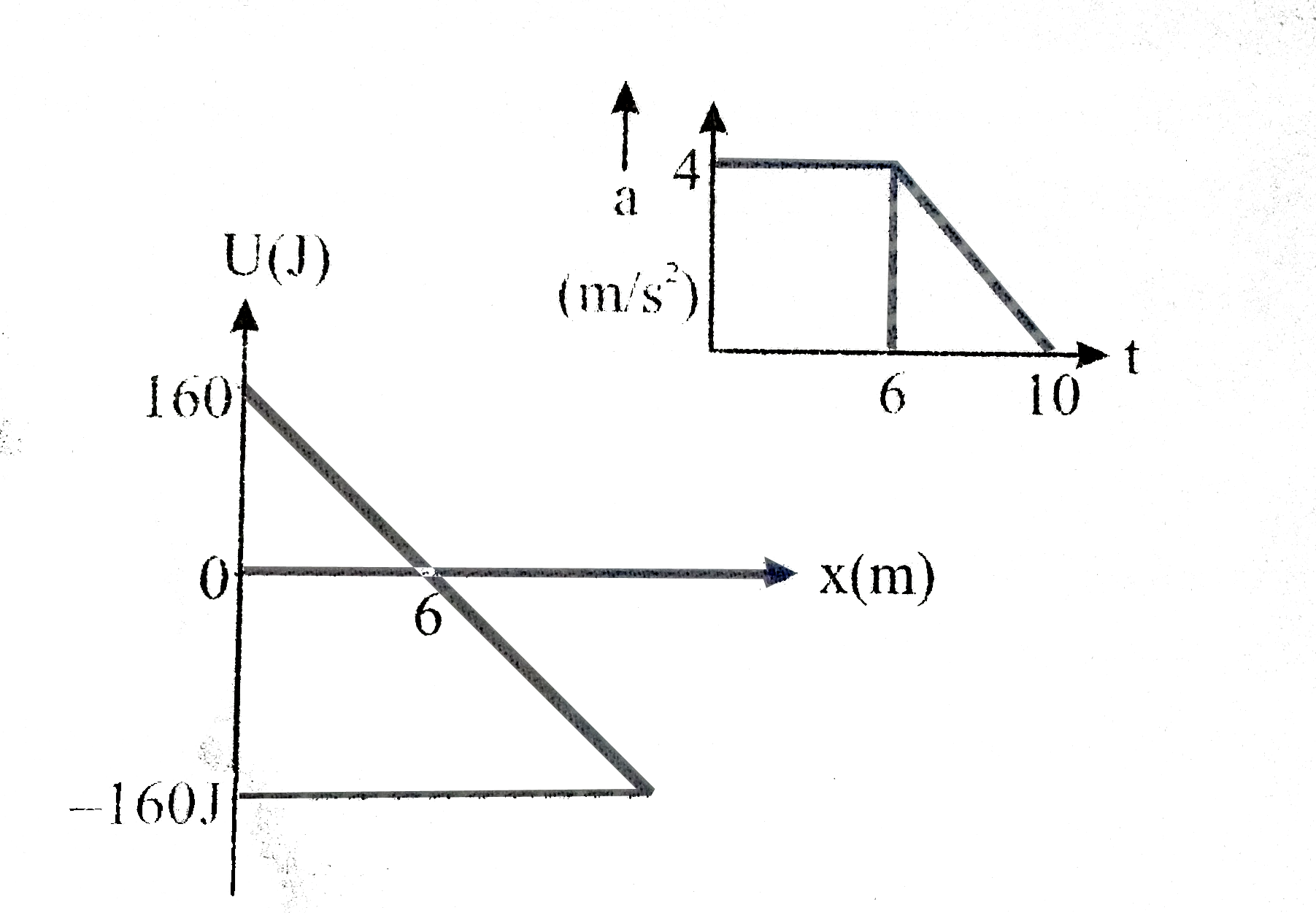 .
.
the work done by all the forces is 704J
the work done by external forces is 350J
the work done by external forces is 384J
the work done by conservation forces is 300J.
The correct Answer is:A, C
Area of (a−t) curve =32ms−1=Vf−Vi
Vf=32+Vi=32+6=38ms−1
work done by all forces =ΔKE
=12m(V2f−V2i)=12(382−62)=704J
work done by external forces =704−320=384J.
Topper's Solved these Questions
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-VI (Comprehension)
20 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-VI (Integer)
12 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-VI (Single Answer)
31 Videos
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE IV
43 Videos
Similar Questions
A time varying force, F=2t is acting on a particle of mass 2kg moving along x-axis. velocity of the particle is 4m/s along negative x-axis at time t=0. Find the velocity of the particle at the end of 4s.
View SolutionA particle is moving in a straight line and its velocity varies with its displacement as v=√4+4s m/s. Assume s=0 at t=0. find the displacement of the particle in metres at t=1s.
View Solution
Knowledge Check
If x,F and U denote the dispalcement, force acting on and potential energy of a particle then
AU=FBF=+dUdxCF=−dUdxDF=1x(dUdx)A particle of mass 1 kg is moving in straight line and its acceleration v/s displacement graph is shown in figure. Work done by the force during x = 0 to x = 10 m is
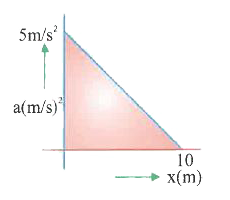 A25 JB26 JC28 JD30 J
A25 JB26 JC28 JD30 JThe mass of particle is 1kg it is moving along x- axis The period of its small osciltion is π2. Find the its potential energy:
A−4sin2xB−16sinxC−16cosxD−4cos2x
The potential energy of a particle of mass 2kg moving along the x-axis is given by U(x)=4x2−2x3( where U is in joules and x is in meters). The kinetic energy of the particle is maximum at
View SolutionA big particle of mass (3+m) kg blasts into 3 pieces , such that a particle of mass 1 kg moves along x - axis , with velocity 2ms−1 and a particle of mass 2 kg moves with velocity 1ms−1 perpendicular to direction of 1 kg particle . If the third particle moves with velocity √2ms−1, then m is
View SolutionThe particle moves with rectilinear motion given the acceleration-displacement (a-S) curve is shown in figure, determine the velocity after the particle has traveled 30 m. If the initial velocity is 10m/s
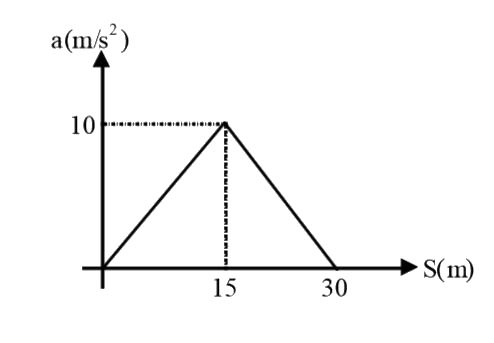
View SolutionComprehension # 5
One particle of mass 1kg is moving along positive x-axis with velocity 3m/s. Another particle of mass 2kg is moving along y-axis with 6m/s. At time t=0,1kg mass is at (3m,0) and 2kg at (0,9m),x−y plane is the horizontal plane. (Surface is smooth for question 1 and rough for question 2 and 3)
The centre of mass of the two particles is moving in a straight line which equation is :-
View SolutionComprehension # 5
One particle of mass 1kg is moving along positive x-axis with velocity 3m/s. Another particle of mass 2kg is moving along y-axis with 6m/s. At time t=0,1kg mass is at (3m,0) and 2kg at (0,9m),x−y plane is the horizontal plane. (Surface is smooth for question 1 and rough for question 2 and 3)
Co-ordinates of centre of mass where it will stop finally are :-
View Solution
