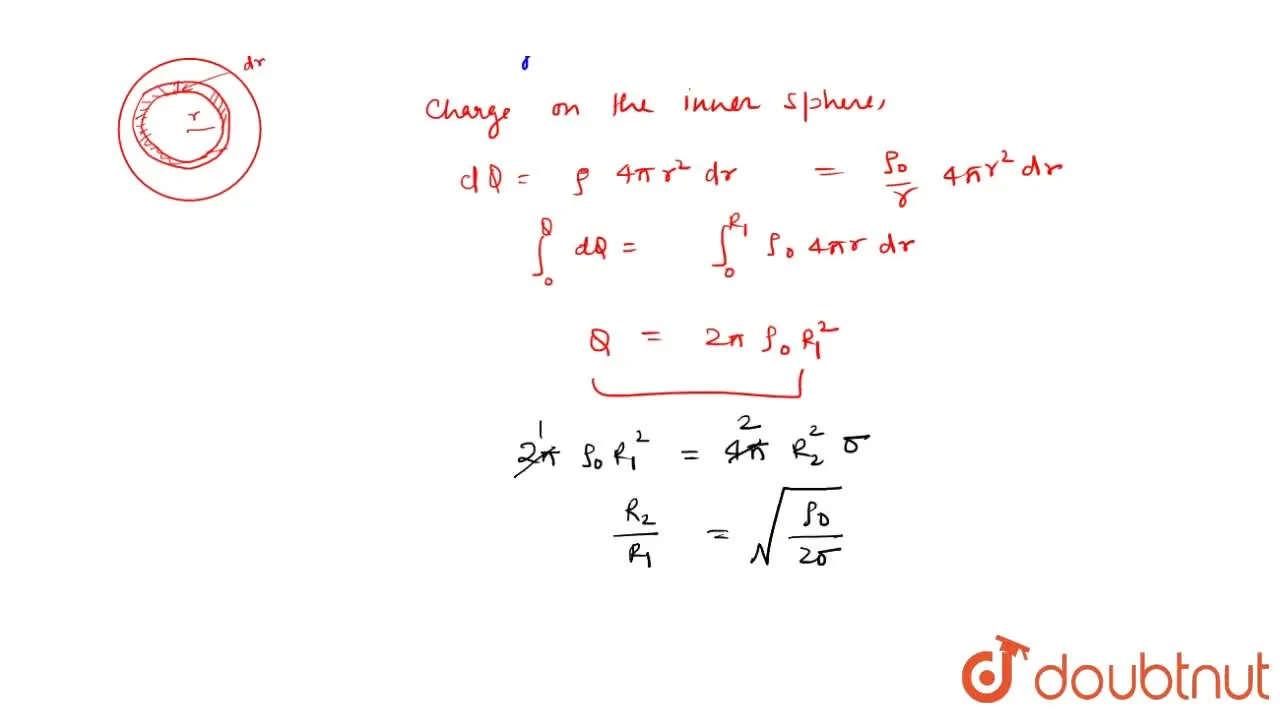
A solid sphere of radius R1 and volume charge density ρ=ρ0r is enclosed by a hollow sphere of radius R2 with negative surface charge density σ, such that the total charge in the system is zero . ρ0 is positive constant and r is the distance from the centre of the sphere . The ratio R2/R1 is
σρ0
√2σρ0
√ρ02σ
ρ0σ
The correct Answer is:3
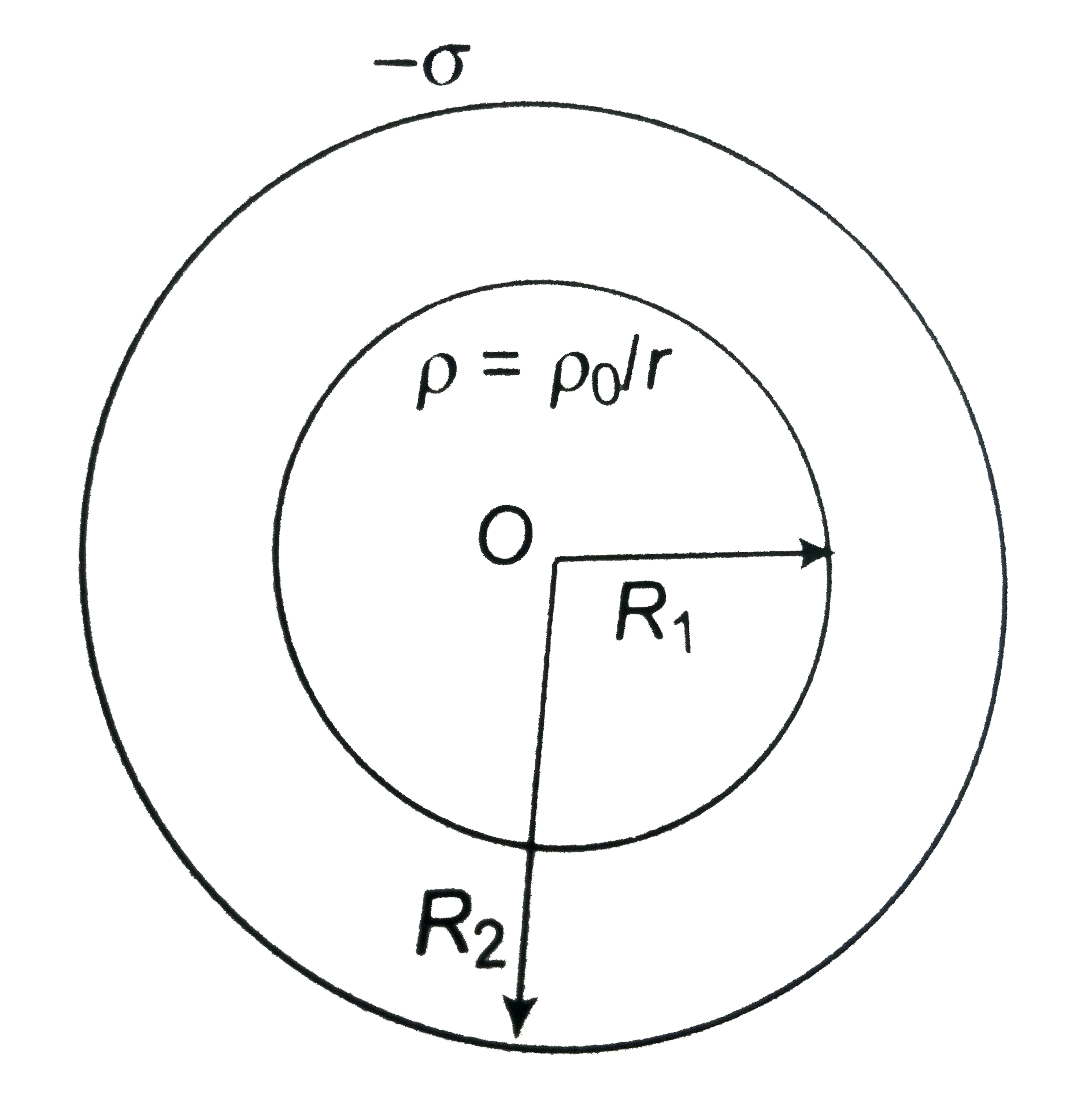
Charge inside sphere of radius R1
Q1=∫ρ.4πr2dr
=∫R10ρ0r4πr2dr=4πρ0∫R10rdr
=4πρ0R212=2πρ0R21
Charge on hollow sphere
Q2=−σ.4πR22
Q1+Q2=0
2πρ0R21−σ.4πR22=0
ρ0R21−2σR22=0
R2R1=√ρ02σ
Related Playlists
Similar Questions
Repeat the question if sphere is a hollow non-conducting sphere of radius R and has uniform surface charge density σ.
View SolutionTwo charged spheres of radii R1andR2 having equal surface charge density. The ratio of their potential is
View SolutionAn insulating solid sphere of the radius R is charged in a non - uniform manner such that the volume charge density ρ=Ar, where A is a positive constant and r is the distance from the centre. The potential difference between the centre and surface of the sphere is
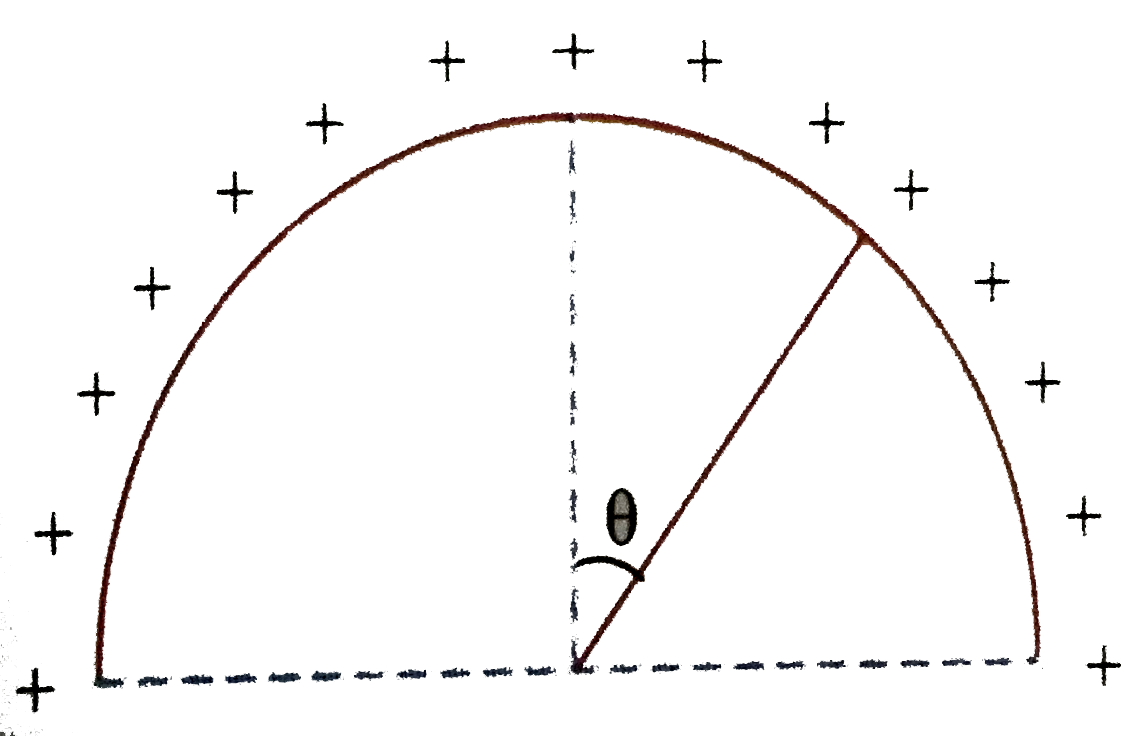
View SolutionFor the hemisphere of radius R shown in the figure the surface charge density σ varies with θ (shown in figure) as σ=σ0cosθ the total charge on the sphere is
 View Solution
View SolutionAn insulating solid sphere of the radius R is charged in a non - uniform manner such that the volume charge density ρ=Ar, where A is a positive constant and r is the distance from the centre. The potential difference between the centre and surface of the sphere is
View SolutionThe electric field intensity in free space at a distance 'r' outside a charged conducting sphere of radius 'R' in terms of surface charge density σ is
View SolutionA sphere of radius R_(0 carries a volume charge density proportional to the distance (x) from the origin, rho=alpha x where alpha is a positive constant.The total charge in the sphere of radius R_(0) is
View SolutionA solid sphere of radius R has a volume charge density ρ=ρ0r2 (where ρ0 is a constant ans r is the distance from centre). At a distance x from its centre (for x<R), the electric field is directly proportional to :
View SolutionA sphere of radius R contains charge density ρ(r)=A(R−r), for 0<r<R. The total electric charge inside the sphere is Q.
The value of A in terms of Q and R isView SolutionA solid sphere of radius R has a charge Q distributed in its volume with a charge density ρ=kra, where k and a are constants and r is the distance from its centre. If the electric field at r=R2 is 18 times that r=R, find the value of a.
View Solution