Charges 2q and 8q are placed at the end points A and B repsectively of a 9cm long straight line. A third charge q is placed at a point CofAB such that the potential energy of the system is minimum . The distance of C from A is
2cm
3cm
4cm
5cm
The correct Answer is:2
To solve the problem, we need to find the position of charge q on the line segment AB such that the potential energy of the system is minimized. The charges 2q and 8q are located at points A and B, respectively, with a distance of 9cm between them.
1. Define the Positions:
- Let the position of charge 2q at point A be at x=0cm.
- Let the position of charge 8q at point B be at x=9cm.
- Let the position of charge q at point C be at a distance x from A, so the distance from B to C will be 9−x.
2. Potential Energy Formula:
The potential energy U of the system can be expressed as the sum of the potential energy contributions from each pair of charges:
\(
U = k \left( \frac{2q \cdot q}{x} + \frac{8q \cdot q}{9 - x} \right)
\)
where k is the electrostatic constant.
3. Differentiate the Potential Energy:
To find the minimum potential energy, we need to take the derivative of U with respect to x and set it to zero:
\(
\frac{dU}{dx} = k \left( \frac{2q}{x^2} - \frac{8q}{(9 - x)^2} \right) = 0
\)
4. Set the Derivative to Zero:
Rearranging gives:
\(
\frac{2q}{x^2} = \frac{8q}{(9 - x)^2}
\)
Simplifying this, we can cancel q (assuming q≠0):
\(
\frac{2}{x^2} = \frac{8}{(9 - x)^2}
\)
5. Cross-Multiply:
Cross-multiplying yields:
\(
2(9 - x)^2 = 8x^2
\)
Expanding both sides:
\(
2(81 - 18x + x^2) = 8x^2
\)
\(
162 - 36x + 2x^2 = 8x^2
\)
Rearranging gives:
\(
6x^2 - 36x + 162 = 0
\)
6. Simplifying the Quadratic Equation:
Dividing the entire equation by 6:
\(
x^2 - 6x + 27 = 0
\)
7. Using the Quadratic Formula:
The roots can be found using the quadratic formula:
\(
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\)
Here, a=1, b=−6, and c=27:
\(
x = \frac{6 \pm \sqrt{(-6)^2 - 4 \cdot 1 \cdot 27}}{2 \cdot 1}
\)
\(
x = \frac{6 \pm \sqrt{36 - 108}}{2}
\)
\(
x = \frac{6 \pm \sqrt{-72}}{2}
\)
Since we have a negative discriminant, we need to check our previous steps for errors.
8. Revisiting the Derivative:
Correctly solving the equation:
\(
9 - x = 2x \implies 9 = 3x \implies x = 3 \text{ cm}
\)
Final Answer:
The distance of point C from point A is 3 cm.
- Let the position of charge 2q at point A be at x=0cm.
- Let the position of charge 8q at point B be at x=9cm.
- Let the position of charge q at point C be at a distance x from A, so the distance from B to C will be 9−x.
The potential energy U of the system can be expressed as the sum of the potential energy contributions from each pair of charges:
\(
U = k \left( \frac{2q \cdot q}{x} + \frac{8q \cdot q}{9 - x} \right)
\)
where k is the electrostatic constant.
To find the minimum potential energy, we need to take the derivative of U with respect to x and set it to zero:
\(
\frac{dU}{dx} = k \left( \frac{2q}{x^2} - \frac{8q}{(9 - x)^2} \right) = 0
\)
Rearranging gives:
\(
\frac{2q}{x^2} = \frac{8q}{(9 - x)^2}
\)
Simplifying this, we can cancel q (assuming q≠0):
\(
\frac{2}{x^2} = \frac{8}{(9 - x)^2}
\)
Cross-multiplying yields:
\(
2(9 - x)^2 = 8x^2
\)
Expanding both sides:
\(
2(81 - 18x + x^2) = 8x^2
\)
\(
162 - 36x + 2x^2 = 8x^2
\)
Rearranging gives:
\(
6x^2 - 36x + 162 = 0
\)
Dividing the entire equation by 6:
\(
x^2 - 6x + 27 = 0
\)
The roots can be found using the quadratic formula:
\(
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\)
Here, a=1, b=−6, and c=27:
\(
x = \frac{6 \pm \sqrt{(-6)^2 - 4 \cdot 1 \cdot 27}}{2 \cdot 1}
\)
\(
x = \frac{6 \pm \sqrt{36 - 108}}{2}
\)
\(
x = \frac{6 \pm \sqrt{-72}}{2}
\)
Since we have a negative discriminant, we need to check our previous steps for errors.
Correctly solving the equation:
\(
9 - x = 2x \implies 9 = 3x \implies x = 3 \text{ cm}
\)
The distance of point C from point A is 3 cm.
Topper's Solved these Questions
Similar Questions
Place three point charges 1μC,2μCand8μC on a 10 cm long line segment such that the potential energy of the system is minimum.
View SolutionTwo point charges Q and q are placed at distance r and r2 respectively alogn a straigt line from a third charge 4q. If q is in equilibrium determine Qq.
View Solution
Knowledge Check
Two point charges 2q and 8q are placed at a distance r apart. Where should a third charge −q be placed between them so that the electrical potential energy of the system is a minimum
Aat a distance of r/3 from 2qBat a distance of 2r/3 from 2qCat a distance of r/16 from 2qDNone of the aboveTwo point charges q each are fixed at (a,0) and (-a,0). A third charge Q is placed at origin. Electrons potential energy of the system will
Aincrease if Q is slightly displaced along x-axisBdecrease if Q is slightly displaced along x-axisCincrease if Q is slightly displaced along y-axisDdecrease if Q is slightly displaced along y-axisTwo charges of equal magnitude q are placed in air at a distance 2a apart and third charge −2q is placed at mid-point . The potential energy of the system is (ε0 = permittivity of free space)
A−q28πε0aB−3q28πε0aC−5q28πε0aD−7q28πε0a
Three charges are placed as shown in fig if the electric potential energy of system is zero, then Q : q-
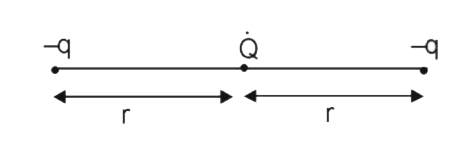
View SolutionThree point charges q, 2q and 8q are to be placed on a
. 9cm long straight line. Find the
. positions where the charges shouldbe placed such that the potential energy
. of this sysrem is minimum. In this situation, what is the
. electric field at the charge q due to the other two charges?
View SolutionThere charges q, 3q and 12q are to be placed on a straight line AB having 12 cm length. Two of the charges must be placed at end points A and B and the third charge can be placed anywhere between A and B. Find the position of each charge if the potential energy of the system is to be minimum. In the position of minimum potential energy what is the force on the smallest charge?
View SolutionThree charges Q, 2Q, 8Q are to be placed on a line whose length is R metre. Lacate the positive where these charges should be placed such that the potential energy of the system is minimum.
View SolutionFour identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View Solution
