A proton and α-particle are placed at separation d on a smooth surface . The ratio of their speed when separation becomes infinite will be
4:1
2:1
1:4
1:2
The correct Answer is:1
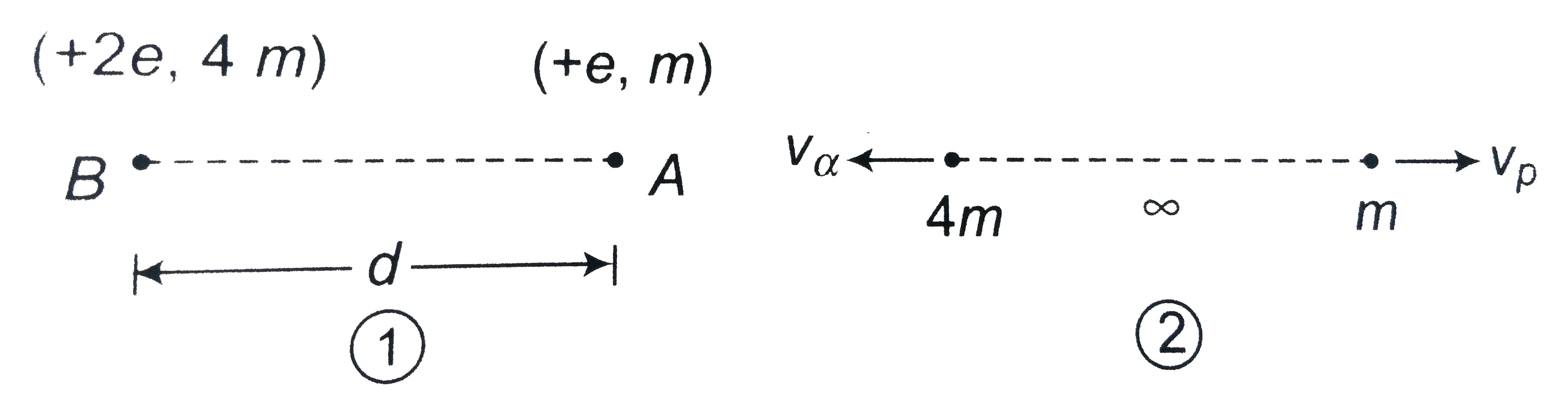
By momentum conservation in situation ① and ②
0=−4mvα+mvp
vpvα=41
Topper's Solved these Questions
Similar Questions
(a) Two protons are placed at separation d on a smooth surface. Find the speed of each proton when separation between them is doubled.
(b) An α-particle and a proton are placed at a separation d on a smooth surface.Find the speed of each when separation between them becomes infinite. (m: mass of proton , e: charge of proton.)
View SolutionA proton and an a-particle have the same de-Broglie wavelength. Determine the ratio of
their speeds.
View Solution
Knowledge Check
A proton and and α- particle are accelerated through a potential difference of 100V. The ratio of the wavelength associated with the proton to that associated with an α - particle is
A√2:1B2:1C2√2:1D12√2:1A proton and an alpha particle are accelerated through the same potential difference. The ratio of the wavelengths associated with the proton to that associated with the alpha particle is
A4B2C√8D1√8A proton and an α - particle are accelerated through same potential difference. Then, the ratio of de-Broglie wavelength of proton and α-particle is
A√2B1√2C2√2DNone of these
Two particles of masses 2m and 3m are placed at separation d on a smooth surface. They move towards each other due to mutual attractive force. Find (a) acceleration of c.m. (b) Velocity of c.m. when separation between particles becomes d/3. ( c) At what distance from the initial position of mass 2m, the particles collide.
View SolutionFind out speed of particles when separation between them is r.

View SolutionA proton and an α-particle have the same de-Broglie wavelength. Determine the ratio of
(i) their accelerating potentials (ii) their speeds.
View SolutionThe force between an α-particle and an electron separated by a distance of 1Å is -
View SolutionA proton and an α− particle enter a uniform magnetic field at right angles to the field with same speed. The ratio of the periods of α− particle and proton is
View Solution