A particle moves along the x-axis according to $s = t^{3} - \frac{15}{2} t^{2} + 12 t + 5$ , where the symbols have their usual meaning.
(a) Determine the time when speed is increasing//decreasing.
(b) At what time, the velocity changes its direction?
(c ) Find the distance traveled in the first six seconds.

Video Solution

Text Solution

Verified by Experts

$s = t^{3} - \frac{15}{2} t^{2} + 12 t + 5$
$v = \frac{d s}{d t} = 3 t^{2} - 15 t + 12 = 3 (t^{2} - 5 t + 4) = 3 (t - 1) (t - 4)$
$a = \frac{d v}{d t} = 6 t - 15 = 3 (2 t - 5)$
When the velocity and acceleration have the same sign, the speed is increasing.
When the velocity and acceleration have opposite signs, the speed is decreasing.
When $t < 2.5 s, a < 0$
When $t > 2.5 s, a > 0$
(a) When $t < 1 s, v > 0, a < 0$ , the speed is decreasing
When $1 s < t < 2.5 s, v < 0, a < 0$ , the speed is increasing
When $2.5 s < t < 4 s, v < 0, a > 0$ , the speed is decreasing
When $t > 4 s, v > 0, a > 0$ , the speed is increasing
(b) $v = 0 = 3 (t - 1) (t - 4) \Rightarrow t = 1 s, 4 s$
At $t = 1 s$ and $t = 4 s$ , the velocity changes direction
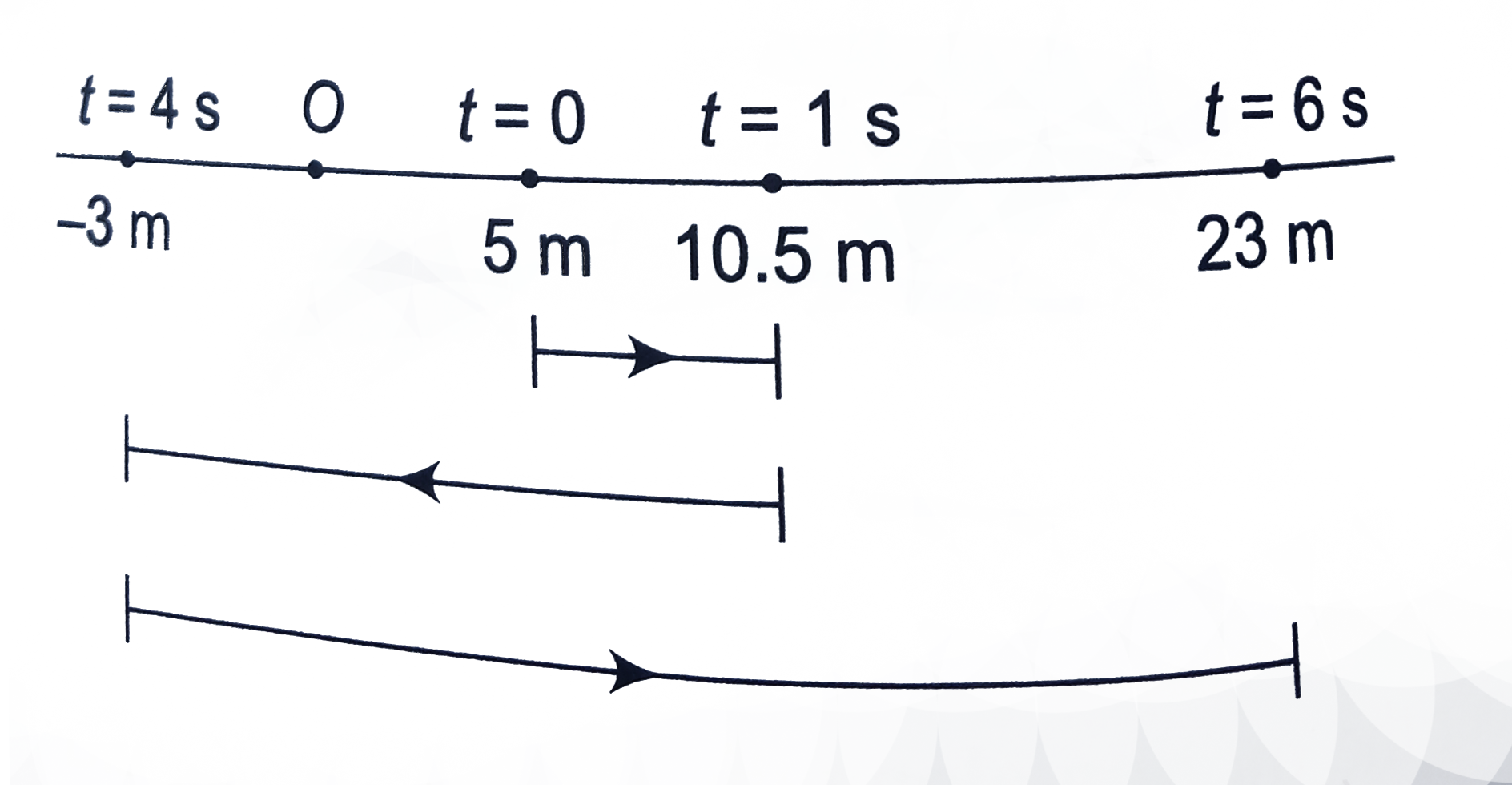
(c ) At $t = 0, s = 5 m$
At $t = 1 s, s = 10.5 m$
At $t = 4 s, s = - 3 m$
At $t = 6 s, s = 23 m$

Updated on:21/07/2023

A particle moves along $x$ -axis. The position of the particle at time $t$ is given as $x = t^{3} - 9 t^{2} + 24 t + 1$ . Find the distance traveled in first $5$ seconds.
A $20 m$
B $10 m$
C $18 m$
D $28 m$
A particle moves along the x - axis according to $x = A [1 + sin ω t]$ . What distance does is travel in time interval from $t = 0$ to $t = 2.5 π / ω$ ?
A $4 A$
B $6 A$
C $5 A$
D $3 A$
A particle moves according to the law $x = t^{3} - 6 t^{2} + 9 t + 5$ . The displacement of the particle at the time when its acceleration is zero, is
A9
B-7
C7
D0