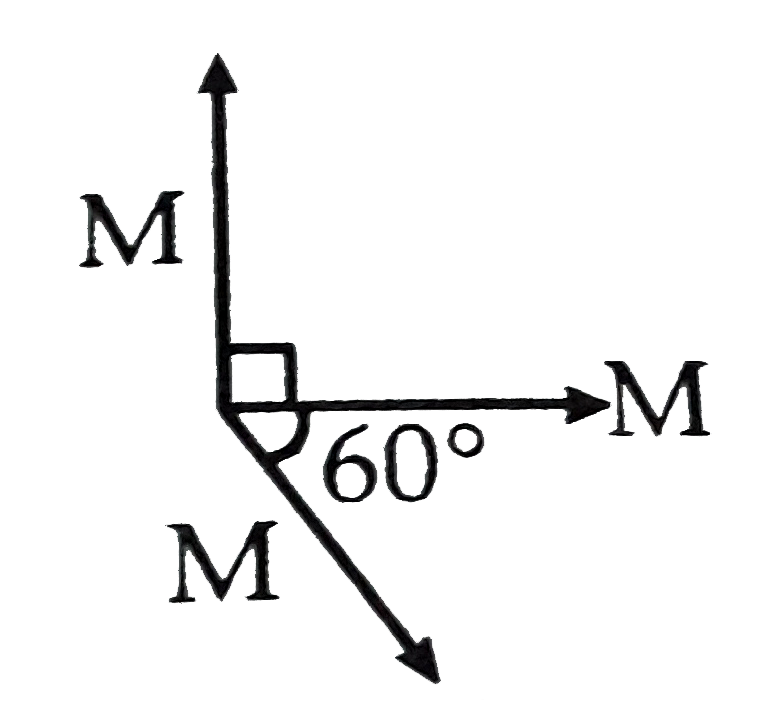
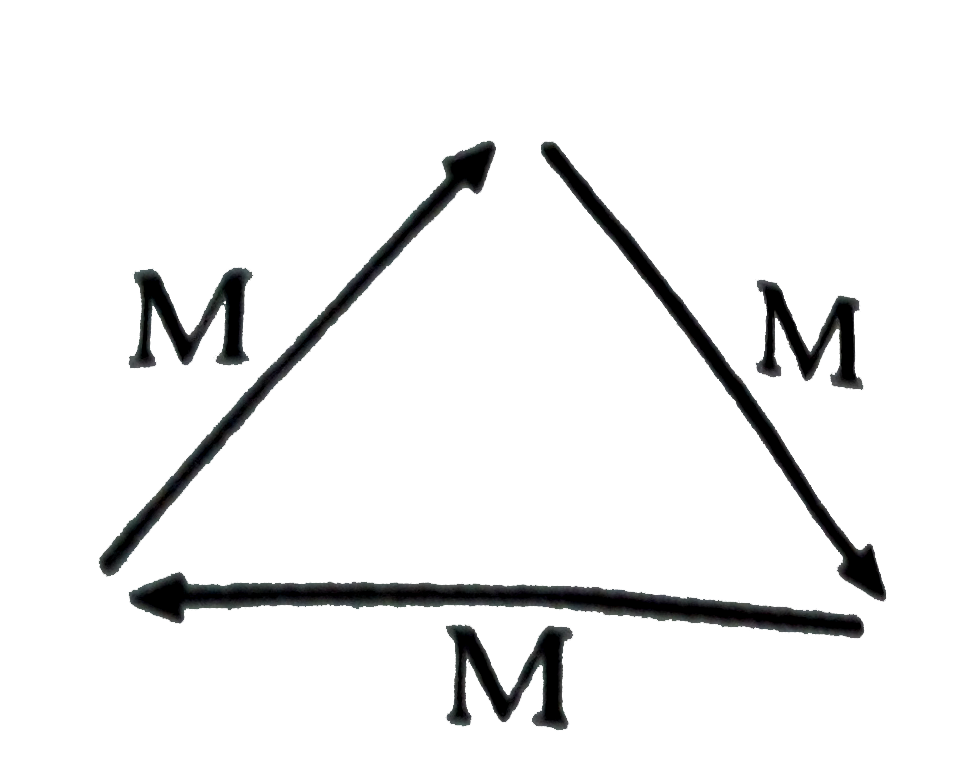
Three magnets of same length but moments M,2M and 3M are arranged in the form of an equilateral triangle with oppositive poles nearer, the resultnat magnetic moment of the arrangement is
6M
zero
√3M
√32M
The correct Answer is:C
To find the resultant magnetic moment of three magnets arranged in an equilateral triangle with moments M, 2M, and 3M, we can follow these steps:
Step 1: Understand the Arrangement
We have three magnets with magnetic moments M, 2M, and 3M arranged in an equilateral triangle. The magnets are positioned such that opposite poles are closer together.
Step 2: Calculate the Pole Strengths
The magnetic moment m of a magnet is given by the formula:
m=p⋅L
where p is the pole strength and L is the length of the magnet. Since the lengths of the magnets are the same, we can express the pole strengths as:
- For magnet with moment M: p1=ML
- For magnet with moment 2M: p2=2ML
- For magnet with moment 3M: p3=3ML
Step 3: Determine the Net Pole Strength
Since the magnets are arranged with opposite poles closer, we need to consider the net pole strength. The net pole strength can be calculated by subtracting the pole strengths of the magnets:
Net Pole Strength=p3−p1−p2=3ML−ML−2ML
This simplifies to:
Net Pole Strength=3M−M−2ML=0L=0
This means that the net effect of the pole strengths cancels out.
Step 4: Calculate Resultant Magnetic Moment
Since the net pole strength is zero, we need to consider the arrangement of the magnets. The effective magnetic moment can be calculated using vector addition, as the magnets are not aligned in a straight line but in an equilateral triangle.
Using the parallelogram law of vector addition for the two resultant magnetic moments:
Let m1=M and m2=2M, and the angle between them is 60∘:
R=√m21+m22+2m1m2cos(60∘)
Substituting the values:
R=√M2+(2M)2+2⋅M⋅2M⋅12
=√M2+4M2+2M2=√7M2=√7M
Step 5: Final Result
The resultant magnetic moment of the arrangement is:
R=√7M
We have three magnets with magnetic moments M, 2M, and 3M arranged in an equilateral triangle. The magnets are positioned such that opposite poles are closer together.
The magnetic moment m of a magnet is given by the formula:
m=p⋅L
where p is the pole strength and L is the length of the magnet. Since the lengths of the magnets are the same, we can express the pole strengths as:
- For magnet with moment M: p1=ML
- For magnet with moment 2M: p2=2ML
- For magnet with moment 3M: p3=3ML
Since the magnets are arranged with opposite poles closer, we need to consider the net pole strength. The net pole strength can be calculated by subtracting the pole strengths of the magnets:
Net Pole Strength=p3−p1−p2=3ML−ML−2ML
This simplifies to:
Net Pole Strength=3M−M−2ML=0L=0
This means that the net effect of the pole strengths cancels out.
Since the net pole strength is zero, we need to consider the arrangement of the magnets. The effective magnetic moment can be calculated using vector addition, as the magnets are not aligned in a straight line but in an equilateral triangle.
Let m1=M and m2=2M, and the angle between them is 60∘:
R=√m21+m22+2m1m2cos(60∘)
Substituting the values:
R=√M2+(2M)2+2⋅M⋅2M⋅12
=√M2+4M2+2M2=√7M2=√7M
The resultant magnetic moment of the arrangement is:
R=√7M
Topper's Solved these Questions
- MAGNETISMBook:NARAYNAChapter:MAGNETISMExercise:LEVEL-II(C.W)
Explore 42 Videos
- MAGNETISMBook:NARAYNAChapter:MAGNETISMExercise:LEVEL-III(C.W)
Explore 30 Videos
- MAGNETISMBook:NARAYNAChapter:MAGNETISMExercise:C.U.Q (ASSERTION & REASON)
Explore 13 Videos
- EXPERIMENTAL PHYSICSBook:NARAYNAChapter:EXPERIMENTAL PHYSICSExercise:Comprehension type
Explore 6 Videos
- MAGNETISM AND MATTERBook:NARAYNAChapter:MAGNETISM AND MATTERExercise:EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
Explore 17 Videos
Similar Questions
Knowledge Check
The resultant magnetic moment for the following arrangement is
 AMB2MC3MD4M
AMB2MC3MD4MThe resultant magnetic moment for the following arrangement is
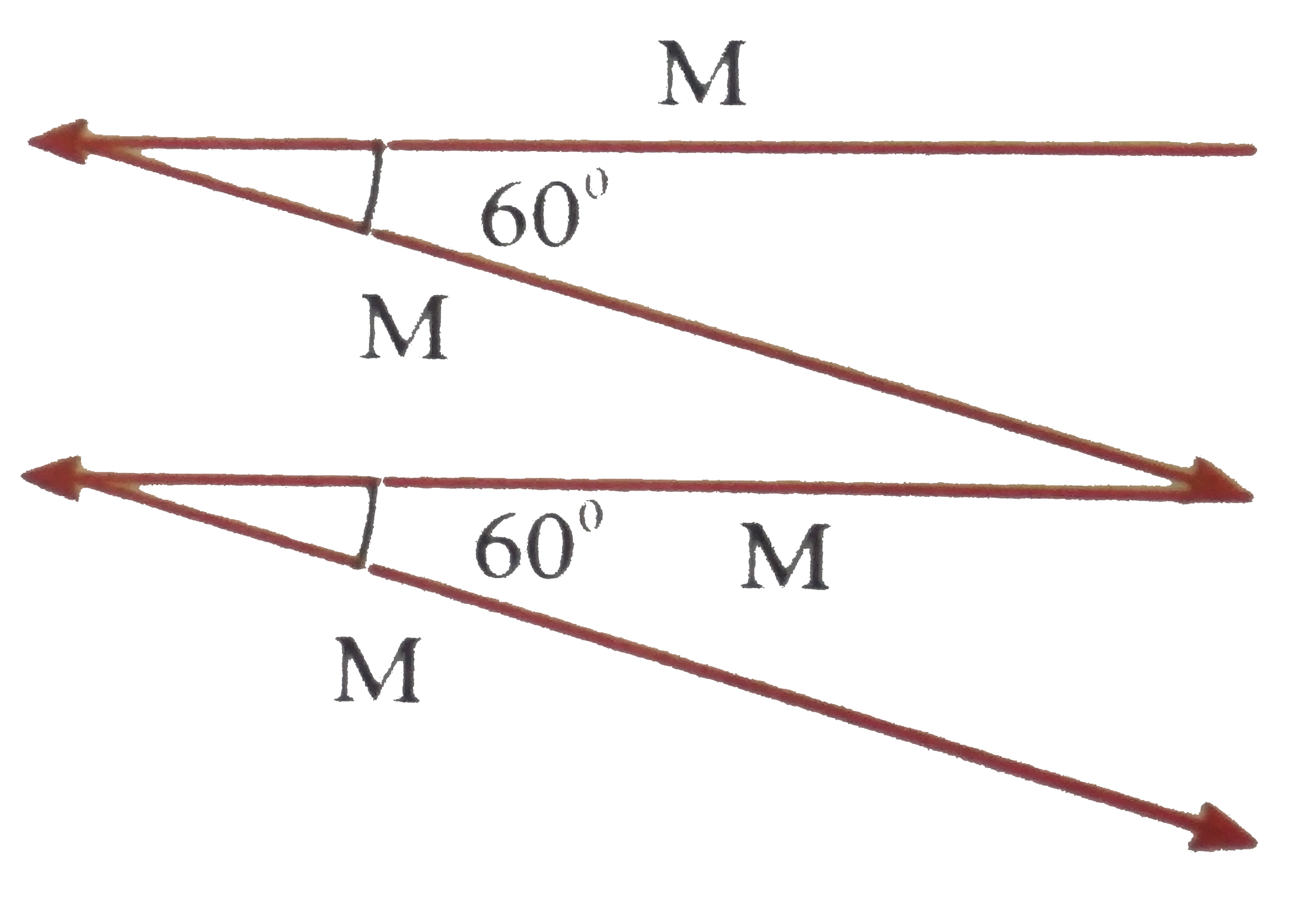 AMB2MC3MD4M
AMB2MC3MD4MThe resultant magnetic moment for the following arrangement
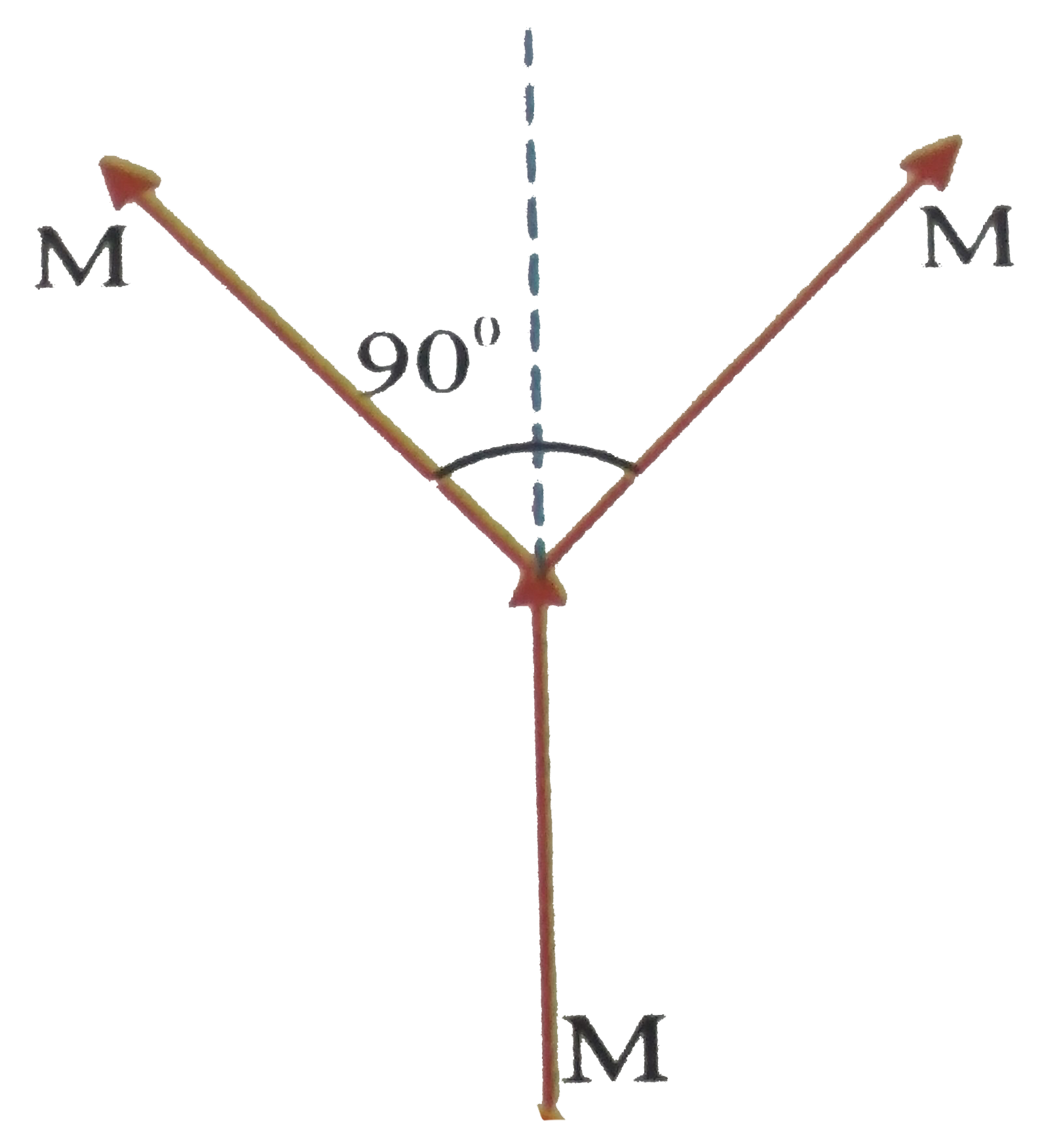 A√2MB(√2+1)MC(√2−1)MDM
A√2MB(√2+1)MC(√2−1)MDM
The resultant magnetic moment for the following arrangement is
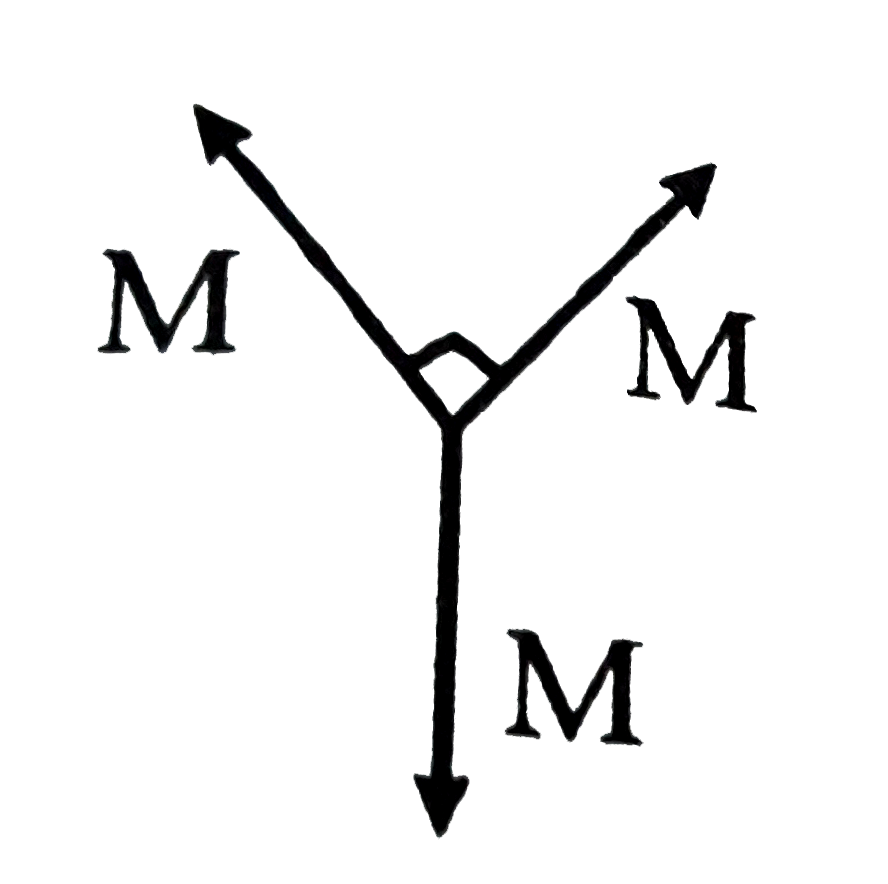
View SolutionThe resultant magnetic moment for the following arrangement is
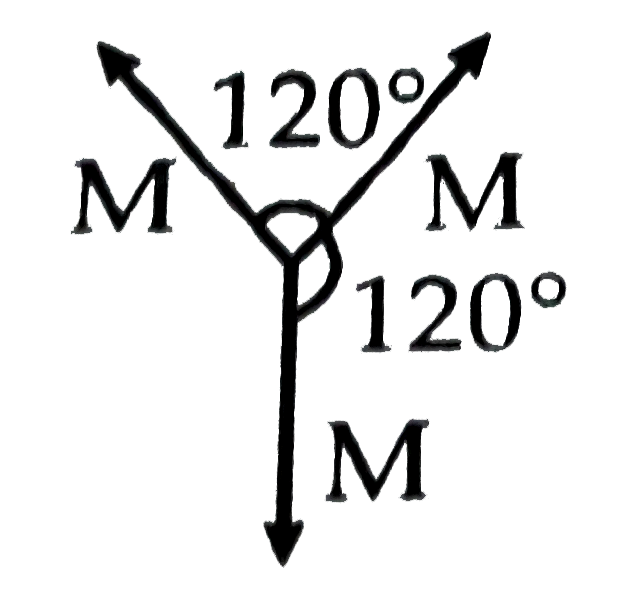
View SolutionThe resulting magnetic moment for the following arrangement is
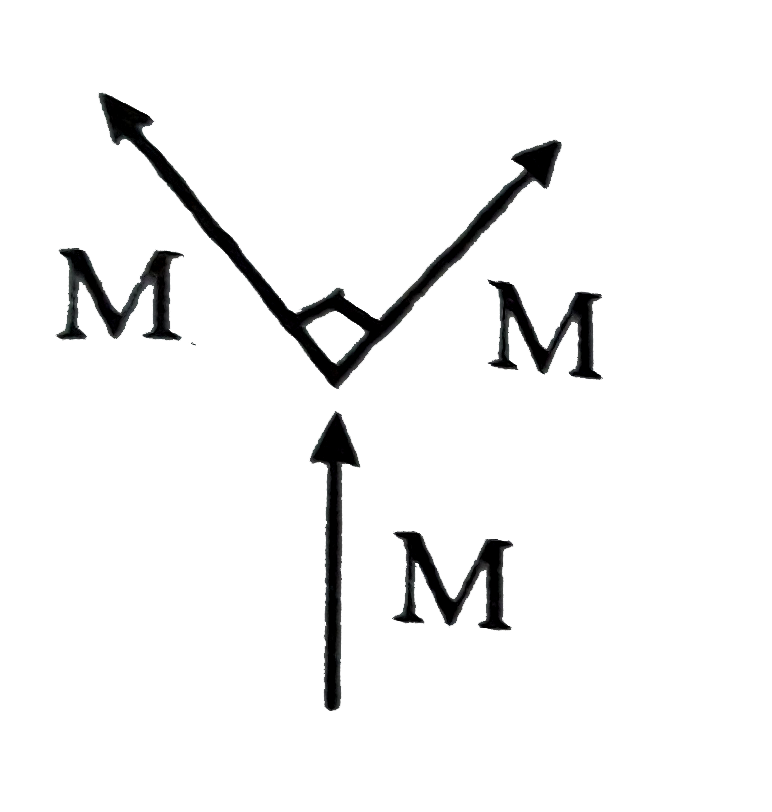
View SolutionThree identical bar magnets each of magnetic moment M are arranged in the form of an equilanteral triangle such that at two vetices like poles are in contact. The resultant magnetic moment will be
View SolutionFour magnets of magnetic momnets M,2M,3M and 4M are arranged in the fome of a square such that unlike poles are in contact. Then the resultant magnetic moment will be
View Solution