A "bar" magnet of moment M is cut into two identical pieces along the length. One piece is bent in the form of a semi circle. If two pieces are perpendicular to each other, then resultant magnetic moment is
(MR)2+(M2)2
√(MR)2+(M2)2
√(MR)2−(M2)2
Mπ+M2
The correct Answer is:C
To solve the problem step by step, we will analyze the situation involving the bar magnet and its resultant magnetic moment after being cut and bent.
Step 1: Understand the Initial Magnetic Moment
The magnetic moment of the original bar magnet is given as M. The length of the magnet is denoted as L.
Step 2: Cut the Magnet
When the bar magnet is cut into two identical pieces along its length, each piece will have:
- Magnetic moment M1=M2
- Length L remains the same for each piece.
Step 3: Bend One Piece into a Semicircle
One of the pieces is bent into the shape of a semicircle. The pole strength of this semicircular piece remains M2.
Step 4: Determine the Radius of the Semicircle
The length of the semicircle is equal to the original length of the magnet, L. The length of a semicircle is given by:
L=πR
From this, we can find the radius R:
R=Lπ
Step 5: Calculate the Magnetic Moment of the Semicircular Piece
The distance between the north and south poles of the semicircular magnet is the diameter, which is:
2R=2×Lπ=2Lπ
The magnetic moment M1 of the semicircular piece can be calculated as:
M1=Pole Strength×Distance=(M2)×(2Lπ)=MLπ
Step 6: Calculate the Magnetic Moment of the Straight Piece
The other piece remains straight and has a magnetic moment M2:
M2=Pole Strength×Length=(M2)×L=ML2
Step 7: Resultant Magnetic Moment Calculation
Since the two pieces are perpendicular to each other, we can use the formula for the resultant of two perpendicular vectors:
Mresultant=√M21+M22
Substituting the values of M1 and M2:
Mresultant=√(MLπ)2+(ML2)2
Step 8: Simplifying the Resultant
Calculating the squares:
Mresultant=√M2L2π2+M2L24=√M2L2(1π2+14)
Factoring out M2L2:
Mresultant=ML√1π2+14
Step 9: Final Expression
Thus, the resultant magnetic moment is:
Mresultant=ML√1π2+14
The magnetic moment of the original bar magnet is given as M. The length of the magnet is denoted as L.
When the bar magnet is cut into two identical pieces along its length, each piece will have:
- Magnetic moment M1=M2
- Length L remains the same for each piece.
One of the pieces is bent into the shape of a semicircle. The pole strength of this semicircular piece remains M2.
The length of the semicircle is equal to the original length of the magnet, L. The length of a semicircle is given by:
L=πR
From this, we can find the radius R:
R=Lπ
The distance between the north and south poles of the semicircular magnet is the diameter, which is:
2R=2×Lπ=2Lπ
The magnetic moment M1 of the semicircular piece can be calculated as:
M1=Pole Strength×Distance=(M2)×(2Lπ)=MLπ
The other piece remains straight and has a magnetic moment M2:
M2=Pole Strength×Length=(M2)×L=ML2
Since the two pieces are perpendicular to each other, we can use the formula for the resultant of two perpendicular vectors:
Mresultant=√M21+M22
Substituting the values of M1 and M2:
Mresultant=√(MLπ)2+(ML2)2
Calculating the squares:
Mresultant=√M2L2π2+M2L24=√M2L2(1π2+14)
Factoring out M2L2:
Mresultant=ML√1π2+14
Thus, the resultant magnetic moment is:
Mresultant=ML√1π2+14
Topper's Solved these Questions
- MAGNETISMBOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - LEVEL-II(C.W)
42 Videos
- MAGNETISMBOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - LEVEL-III(C.W)
30 Videos
- MAGNETISMBOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - C.U.Q (ASSERTION & REASON)
13 Videos
- EXPERIMENTAL PHYSICSBOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Comprehension type
6 Videos
- MAGNETISM AND MATTERBOOK - NARAYNACHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
17 Videos
Similar Questions
A bar magnet of moment M is cut into two identical pieces the length.One piece is bent in the form of a semi circle.The two pieces are arranged as shown.The resulting moment is

View SolutionA bar magnet of magnetic moment M is cut into four parts of equal length. The magnetic moment of each part is
View Solution
Knowledge Check
A bar magnet of moment M is bent into arc, its moment
AdecreasesBincreasesCdoes not changeDmay changeWhen a magnet is cut into two pieces along its length,then each piece of magnet will have
ASame pole strengthBReduced pole strengthCIncreased pole strengthDCan not be predictedA bar magnet of magnetic moment M1 is axially cut into two equal parts. If these two pieces are arranged perpendiucular to each other, the resultant magnetic moment is M2.
The the vale of M1M2 isA12√2B1C1√2D√2
A magnet is cut into two pieces perpendicular to its legth,then each piece will be a magnet of
View SolutionA bar magnet of magnetic moment M is cut into two parts of equal length/breadths.The magnetic moment of each part will be
View SolutionA magnet of length 2L abd moment 'M' is axially cut into equal halves 'P' and 'Q'. The piece 'P' is bent in the form of semi circle and 'Q' is attached to it as shown. Its moment is
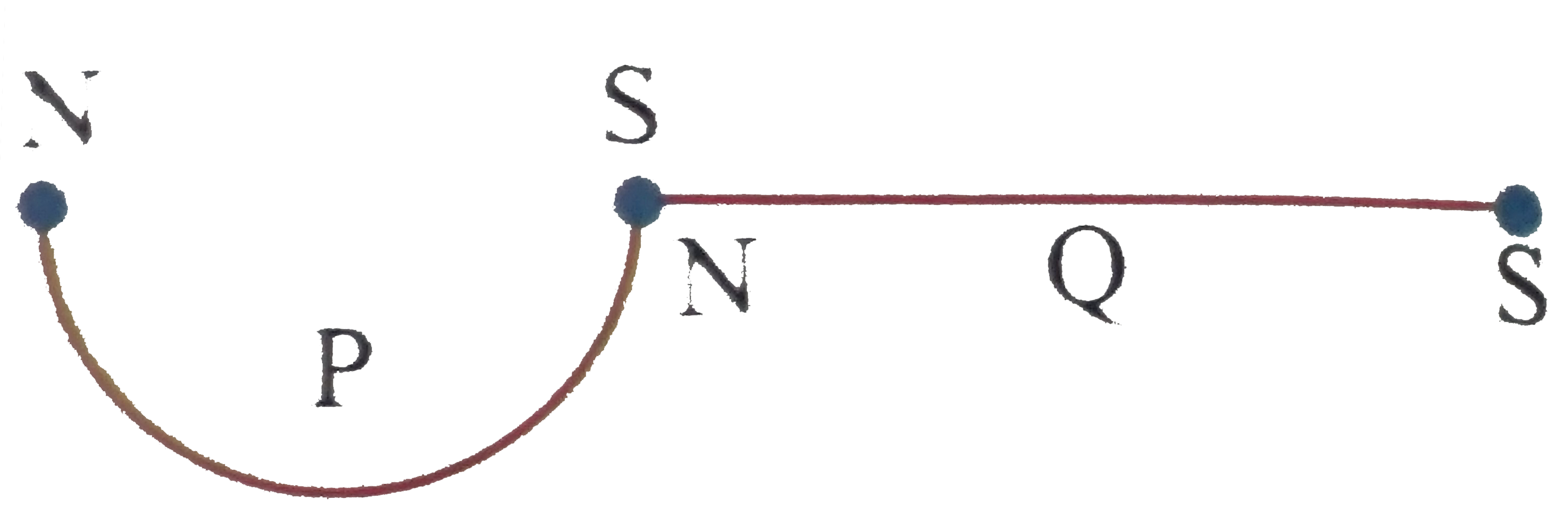
View SolutionA bar magnet of magnetic moment M is cut into two parts of equal length/breadths.The magnetic moment of each part will be
View SolutionA bar magnet of moment 'M' is bent into a shape
 . If the length of the each part is same, its new magnetic moment will be
. If the length of the each part is same, its new magnetic moment will be
View Solution
