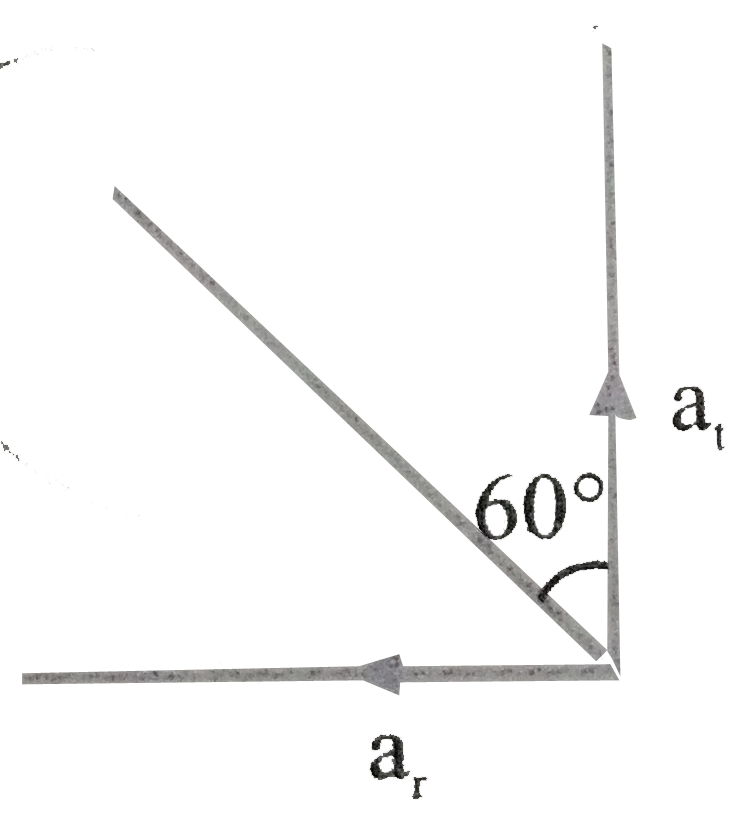
A solid body starts rotating about a stationary axis with an angular a

A body starts rotating from rest and completes 10 revolutions in 4 s .Find its angular acceleration
A $2.5 π r a d / s^{2}$
B $5 π r a d / s^{2}$
C $7.5 π r a d / s^{2}$
D $10 π r a d / s^{2}$
A stationary wheel starts rotating about its own axis at uniform rate amgular acceleration $8 r a d / s^{2}$ .The time taken by its to complete $77$ rotation is
A $5.5 sec$
B $7 sec$
C $11 sec$
D $14 sec$
A stationary wheel starts rotating about its own axis at an angular acceleration $5.5 r a d / s^{2}$ . To acquire an angular velocity 420 revolutions per minute , the number of rotations made by the wheel is
A14
B21
C28
D35

A body starts rotating from rest and completes 10 revolutions in 4 s .Find its angular acceleration