A circuit consists of a capacitor with capacitance C and a coil with active resistance R and inductance L connected in parallel. Find the impedane of the circuit at frequency ω of alternating voltage.
i1=VXC current leads the voltage by π/2
i2=V/√R2+X2L current lags the voltage by phi
where tanϕ=XL/R
i=√i21+22+2i1i2cos(90+ϕ)=√i21+i22−2i1i2sinϕ
VZ=
⎷V2X2C+V2R2+X2L−2VXC.V√R2+X2C.XL√R2+X2L
1Z=[1X2C+1R2+X2L−2(XL/XC)(R2+X2L)]12
=[R2+X2L+X2C−2XLXCX2C(R2+X2L)]12
=⎡⎢
⎢
⎢
⎢⎣R2X2C+(XL−XCXC)2(R2+X2L)⎤⎥
⎥
⎥
⎥⎦12
Z=√R2+ω2L2(ωCR)2+(ω2LC−1)2
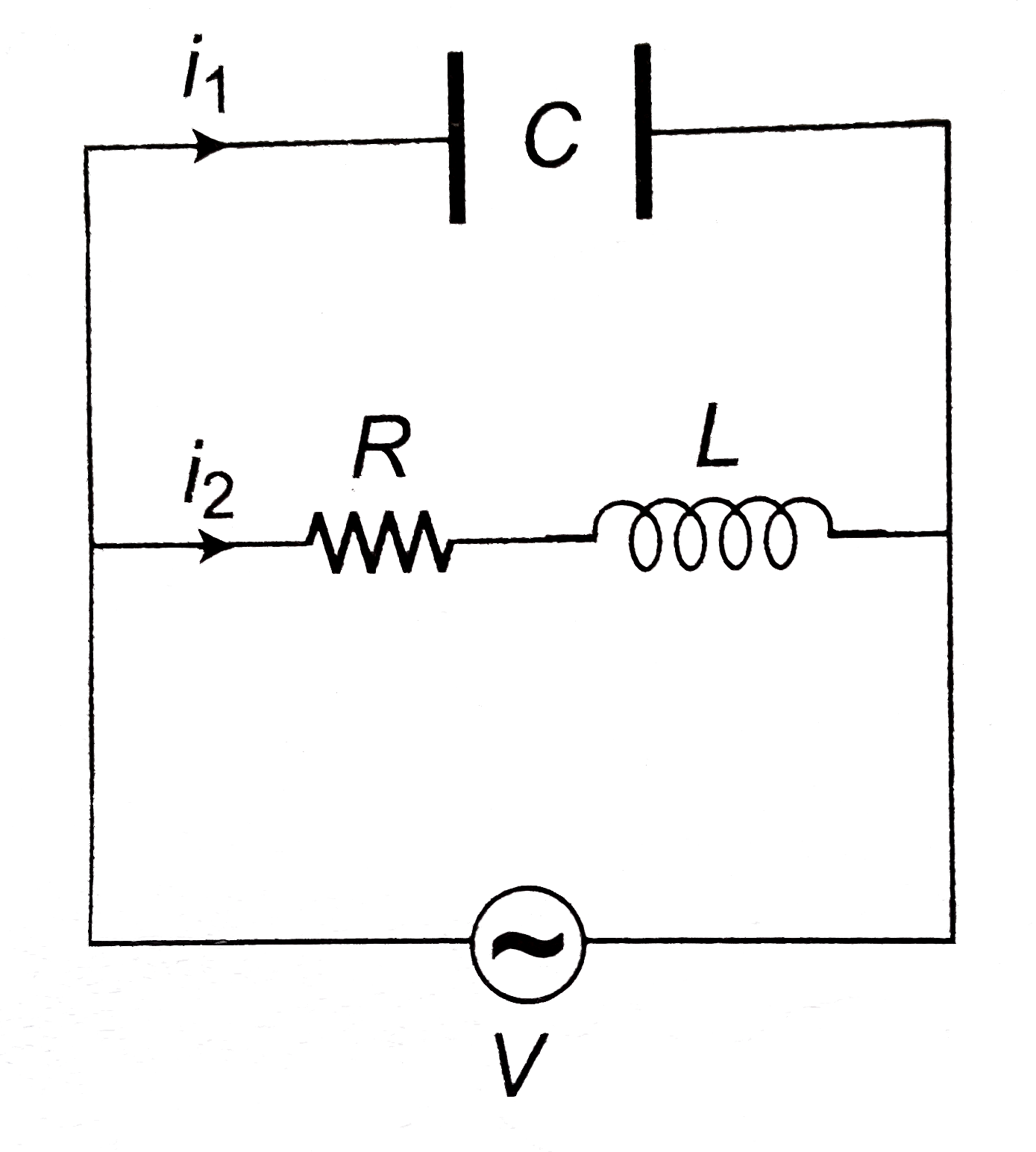
 .
.
Topper's Solved these Questions
Similar Questions
An alternating current of frequency ω=314s−1 is fed to a circuit consisting of a capacitor of capacitance C=73μF and an active resistance R=100Ω connected in parallel. Find the impedance of the circuit.
View SolutionA capacitor with capacitance C and a coil with active resistance R and inductance L are connected in parallel to a source of sinusoidal voltage of frequency ω. Find the phase difference between the current fed to the circuit and the source voltage.
View Solution
Knowledge Check
The power factor of an AC circuit having resistance (R) and inductance (L) connected in series and an angular velocity ω is
AR/ωLBR/(R2+ω2L2)1/2CωL/RDR/(R2−ω2L2)1/2
A series circuit consisting of a capacitor with capacitance C=22μF and a coil with active resistance R=20Ω and iniductance L=0.35H is connected to a source of alternating voltage with amplitude Vm=180V and frequency ω=314s−1. Find :
(a) the current amplitude in the circuit,
(b) the phase difference between the current and the external voltage ,
(c) the amplitudes of voltage across the capacitor and the coil.
View SolutionAn oscillating circuit consist of a capacitor with capacitance C=4.0μF and a coil with inductance L=2.0μH and active resistance R=10Ω. Find the ration of the energy of the coil's magnetic field to that of the capacitor's electric field at the moment when the current has the maximum value .
View SolutionA certain oscillating circuit consits of a capacitor with capacitance C, a coil with inductance L and active resistance R, and a switch . When the swith was disconnected, the capacitor was charged, then the switch was closed and oscillations set in. Find the ratio of the voltage across the capacitor to its peak value at the moment immediatel after closing the switch.
View SolutionA series circuit consisting of a capacitor with capacitance with capacitance C, a rasistance R, and a coil with inductance L and negligible active resistance is connected to an oscillator whose frequency can be varied without changing the voltag amplitude. Find the frequency at which the voltage amplitude is maximum
(a) across the capacitor ,
(b) across the coil.
View SolutionA circuit consists of a capacitor with capacitance C and a coil of inductance L connected in series, as well as a switch and a resistance equal to the critical value for this circuit. With the switch disconnected, the capacitor was charged to a voltage V0, and at the moment t=0, the switch was closed . Find the corrent I in the circuit as a function of time t.What is Imax equal to ?
View SolutionA series circuit consisting of a capacitor XC=80Ω and a coil with active resistance R=300Ω and inductance XL=40ω is connected to a source of alternating voltage with amplitude V0=200V Find
(a) the current amplitude in the circuit
(b) the phase difference between the current and voltage
(c) the amplitudes of voltage across the capacitor and the coli .
View SolutionA circuit consisting of a capacitor with capacitance C and a resistance R connected in series was connected at the moment t=0 to a source of ac voltage V=Vmcosωt . Find the current in the circuit as a function of time t.
View Solution
