A heated object (at time t=0 and temperature T=T0) is taken out of the oven to cool and placed on a table near an open window Write an expression for its temperature as function of time T where Ts is the surrounding temperature .
T=Ts−(T0+Ts)e−kt
T=Ts+(T0+Ts)e−kt
T=Ts+(T0−Ts)e−kt
T=Ts−(T0−Ts)e−kt
The correct Answer is:C
dθdt=−K(T−Ts)
T∫T0dθT−Ts=−Kt∫0dtlog(T−TsT0−Ts)=−Kt+C .
Topper's Solved these Questions
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - NCERT BASED QUESTIONS
5 Videos
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - SINGLE ANSWER QUESTIONS Passage
1 Video
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-III (C.W)
1 Video
- THERMODYNAMICSBOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise
187 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - STATEMENT TYPE QUESTION
23 Videos
Similar Questions
A rod of length l with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as k=a/T, where a is a constant. The ends of the rod are kept at temperatures T1andT2. Find the function T(x), where x is the distance from the end whose temperature is T1.
View SolutionA cylindrical conductor has a resistance R. When the conductor is at a temperature (T) above its surrounding temperature (T0), the ratio of thermal power dissipated by the conductor to its excess temperature (ΔT=T–T0) above surrounding is a known constant k. The conductor is connected to a cell of emf V. Initially, the conductor was at room temperature T0. Mass and specific heat capacity of the conductor are m and s respectively.
(i) find the time (t) dependence of the temperature (T) of the conductor after it is connected to the cell. Assume no change in resistance due to temperature.
(ii) find the temperature of the conductor after a long time.
View Solution
Knowledge Check
If a piece of metal is heated to temperature θ and the allowed to cool in a room which is at temperature θ0, the graph between the temperature T of the metal and time t will be closet to
A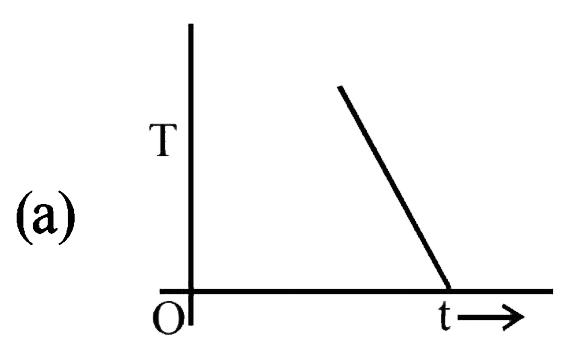 B
B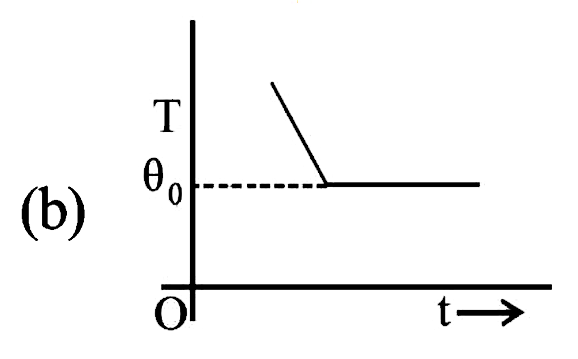 C
C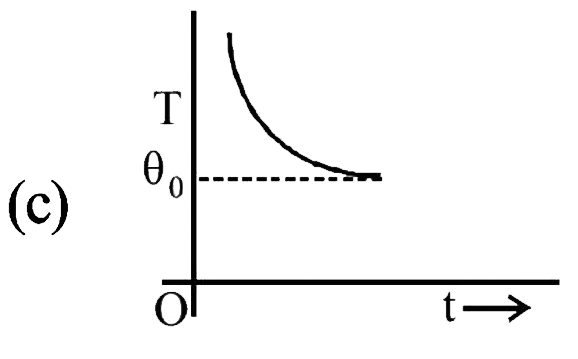 D
D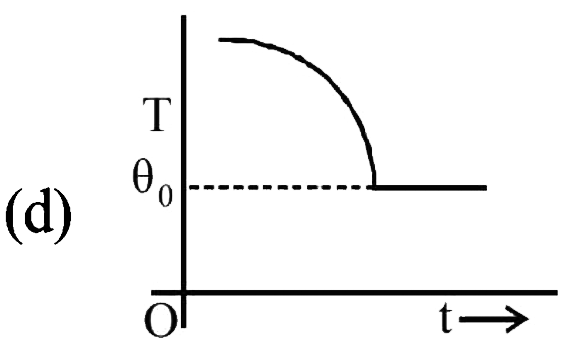
Two identical objects A and B are at temperature TA and TB respectively. Both objects are placed in a room with perfectly absorbing walls maintained at temperature T(TA>T>TB).
Statement-1: The objects A and B attain the temperature T eventually
Statement-2: A only emits radiation while B only absorbs until radiation both attain the temperature TAStatement-1 is true, statement-2 is true and statement-2 is correct explanation for statement-1.BStatement-1 is true, statement-2 is true and statement-2 is NOT the correct explanation for statement-1.CStatement-1 is true, statement-2 is false.DStatement -1 is false, statement -2 is true.The graph shows the variation of lnRv/s1T2, where R is resistance and T is temperature. Then find R as function T.
_2019_CBT5_E01_037_Q01.png) AR−R0eT02/T2BR−R0e−T2/T02CR−R0eT3/T0DR−R0e−T3/T03
AR−R0eT02/T2BR−R0e−T2/T02CR−R0eT3/T0DR−R0e−T3/T03
A solid body A of mass m and specific heat capacity ‘s’ has temperature T1=400K. It is placed, at time t=0, in atmosphere having temperature T0=300K. It cools, following Newton’s law of cooling and its temperature was found to be T2=350Kat time t0. At time t0, the body A is connected to a large water bath maintained at atmospheric temperature T0, using a conducting rod of length L, cross section A and thermal conductivity k. The cross sectional area A of the connecting rod is small compared to the overall surface area of body A. Find the temperature of A at time t=2t0.
View SolutionTwo rigid boxes containing different ideal gases are placed on a table. Box A contains one mole of nitrogen at temperature T0, while Box contains one mole of helium at temperature (73)T0. The boxes are then put into thermal contact with each other, and heat flows between them until the gasses reach a common final temperature (ignore the heat capacity of boxes). Then, the final temperature of the gasses, Tf in terms of T0 is
View SolutionA body at temperature θ0 having Newton's cooling constant K is placed in a surrounding having temperature T0 at time t = 0. The graph of temperature of the body as a function of time t is shown in the adjacent figure. A tangent on the curve is drawn at t = 0 as shown in the figure. Find the value of τ.

View SolutionIf the temperature of the body θ and time of cooling 't' and room temperature of the surrounding θ0. The cooling curve is
View SolutionThe resistance of an electrolyte varies with temperature T as RT=RO1+αT, where R0 and are constants. A constant potential difference V is applied to the two electrodes (each of surface area A) which are dipped in this electrolyte. If T0 is the temperature of the surroundings, the loss of heat to the surroundings per unit surface area put unit time is governed by the same relation H=k(T−T0)A, the steady state temperature is
View Solution
