Angular acceleration of the cylinder C shows in the figure is (all strings and the pulley are ideal)
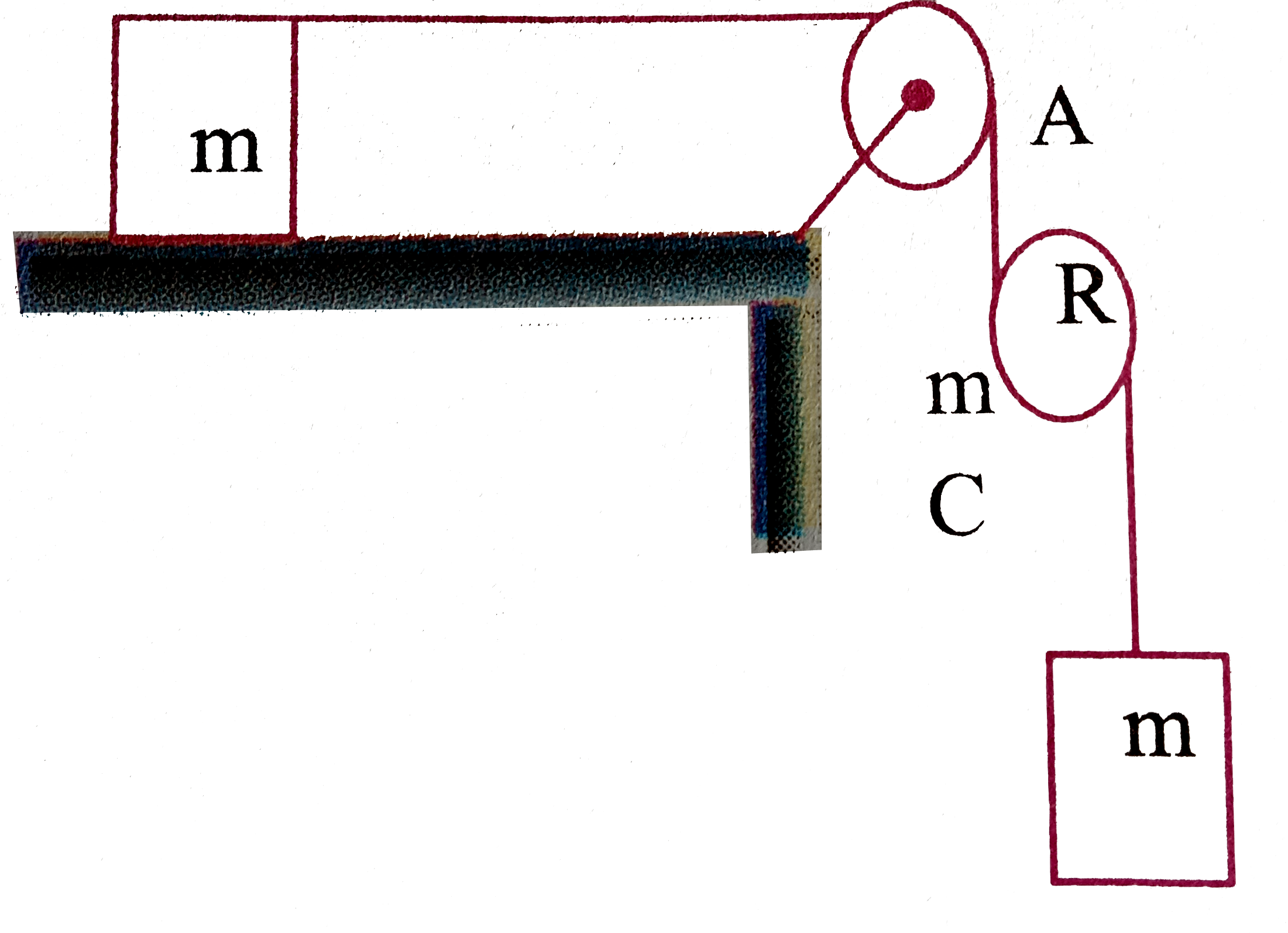
2g3R
2g5R
2gR
g2R
The correct Answer is:B
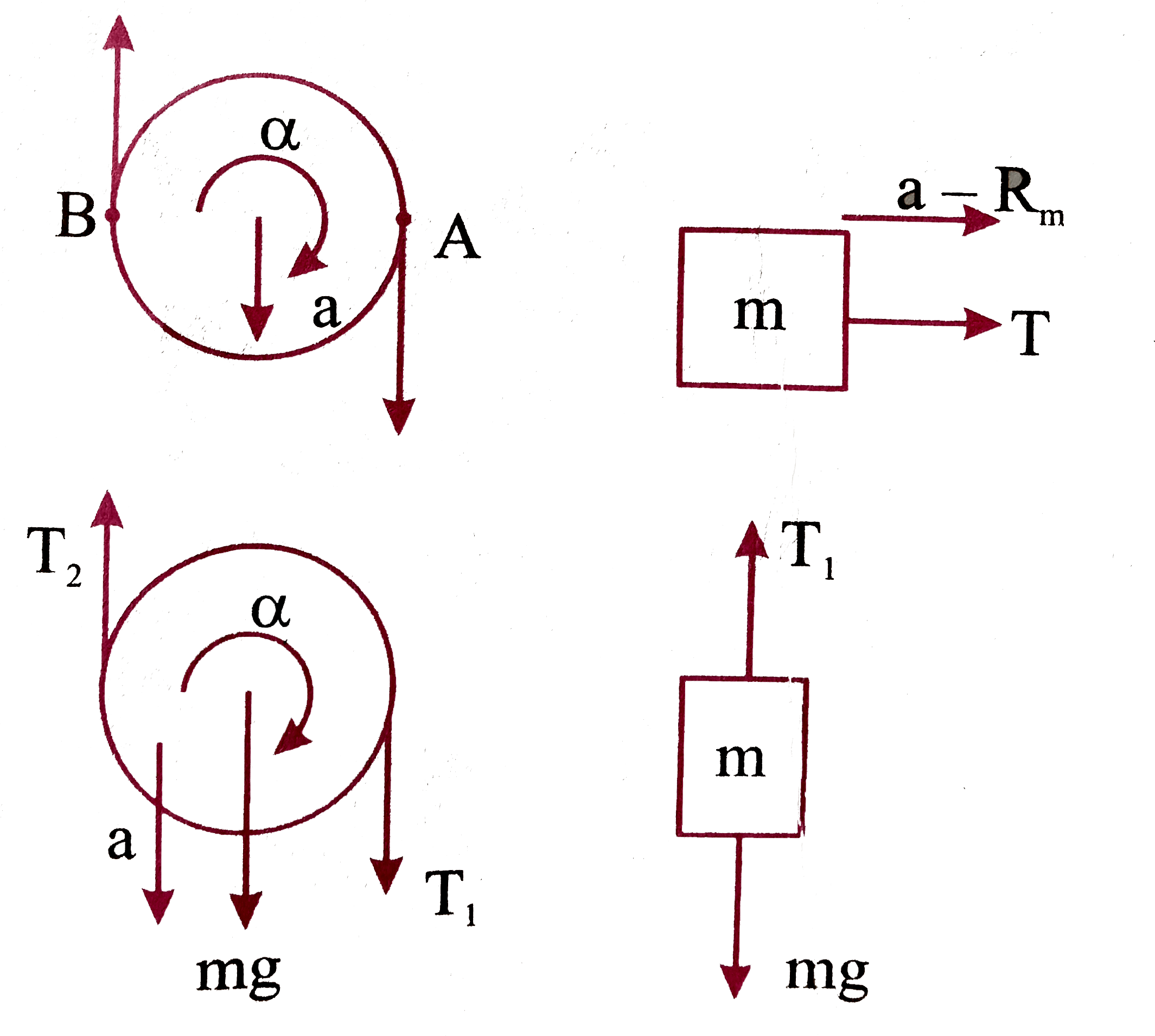
|→aA|=a+Rα
|→aB|=a−Rα
mg−T1=m(a+Rα)......(1)
T1+mg−T2=ma......(2)
T2=m(a−Rα)......(3)
T1R+T2R=mR22α
On solving these eqn. we get
a=2g3,α=2g5R
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-V
Explore 72 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
Calculate the accelerations of the pulleys B and C and the tension in the string passing over the pulley A of the figure Figure 5.22.
View SolutionConsider the situation shown in the figure. The surface is smooth and the string and the pulley are light. Find the acceleration of each block and tension in the string.
View Solution
Knowledge Check
A string is warapped around a cylinder of mass m and radius r. The string is also connected to a block of same mass m with the help of another pulley as shown in figure. The angular acceleration of the cylinder is ( friction is sufficient for rolling ) ( all pulleys are ideal)
 Ag(2−sinθ)16RB2g(4−sinθ)35RC7g(3−sinθ)25RDg(2−sinθ)12R
Ag(2−sinθ)16RB2g(4−sinθ)35RC7g(3−sinθ)25RDg(2−sinθ)12RFind the acceleration of the 6 kg block in the figure. All the surfaces and pulleys are smooth. Also the strings are inextensible and light. [Take g=10m/s2]
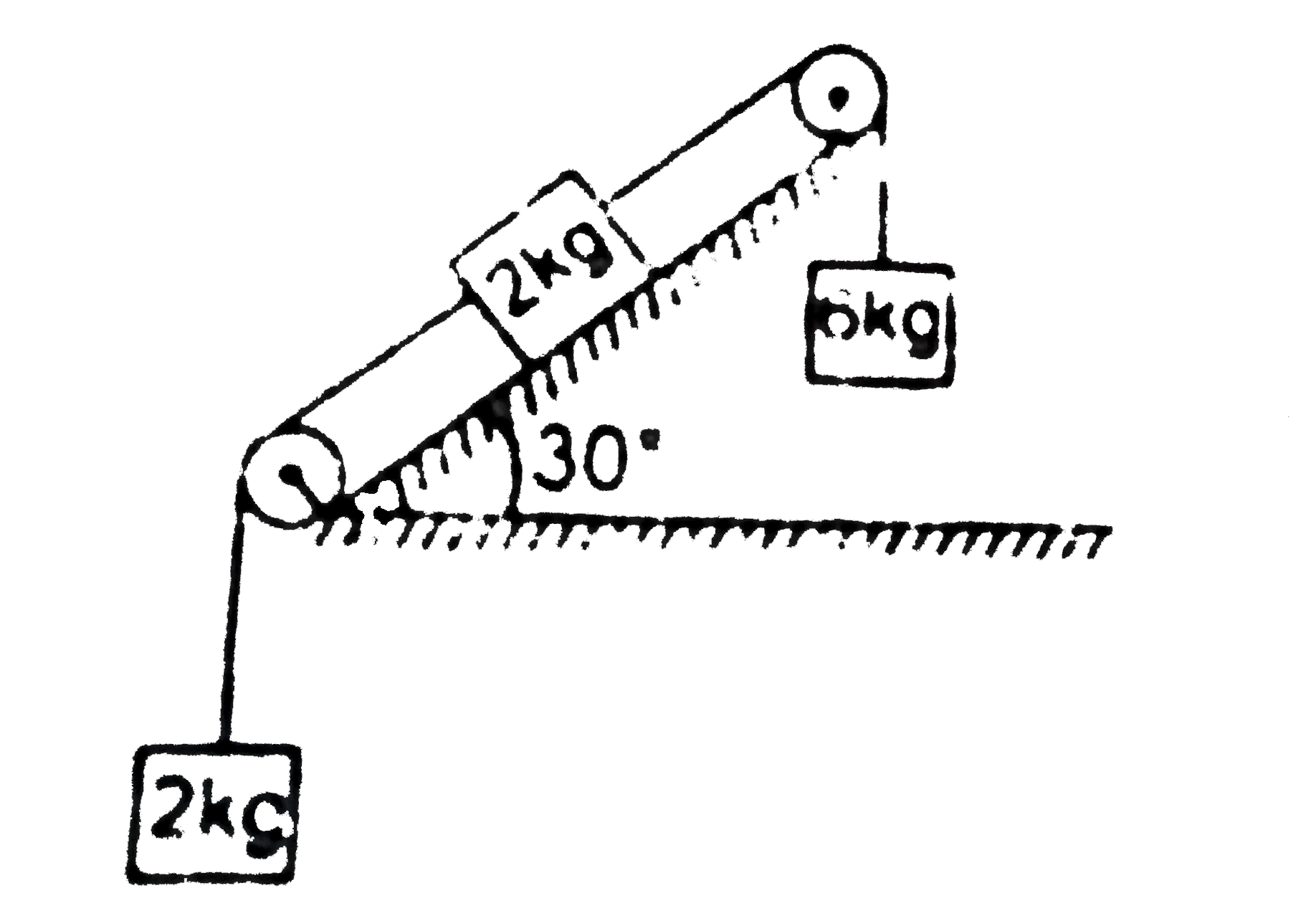 .A3m/s2B4m/s2C5m/s2D2m/s2
.A3m/s2B4m/s2C5m/s2D2m/s2Acceleration of pulleys and blocks are as shown in the figure. All pulleys & strings are massless & frictionless magnitude of aA and aB are :
 .AaA=0,aB=7BaA=0,aB=5CaA=0,aB=12DaA=5,aB=7
.AaA=0,aB=7BaA=0,aB=5CaA=0,aB=12DaA=5,aB=7
A belt moves over two pulleys A and B as shown in the figure. The pulleys are mounted on two fixed horizontal axles. Radii of the pulleys A and B are 50 cm and 80 cm respectively. Pulley A is driven at constant angular acceleration of 0.8 rad/s2 unitl pulley B acquries an angular velocity of 10 rad/s. The belt does not slide on either of the pulleys.
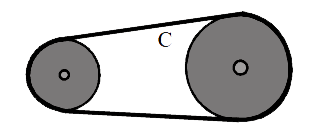
(a)Find the acceleration of a point C on the belt and angular acceleration of the pulley B.
(b) How long does it take for the pulley B to acquire an angular velocity of 10 rad/s ?
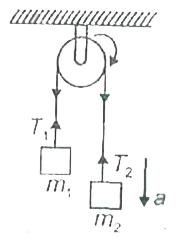
View SolutionFigure shows a pulley of mass m and radius r with two blocks of masses m1 and m2 attached with a light and unstretchable string. Find the acceleration of the blocks, tensions in the string and the force exerted by the pulley on the celling from which it is hanging. Assume no slipping between the string and the wheel.
View SolutionConsider the situation shown in figure. All the surfaces are frictions and the string and the pulley are light. Find the magnitude of the acceleration of the two blocks.

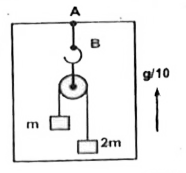
View SolutionIn the arrangement shown in the figure the elevator is going up with an acceleration of g/10. If the pulley and the string are light and the pulley is smooth, the tension in the string AB is
View SolutionThe acceleration of the block (A) and (B) respectively in situation shown in the figure is: (pulleys and string are massless).
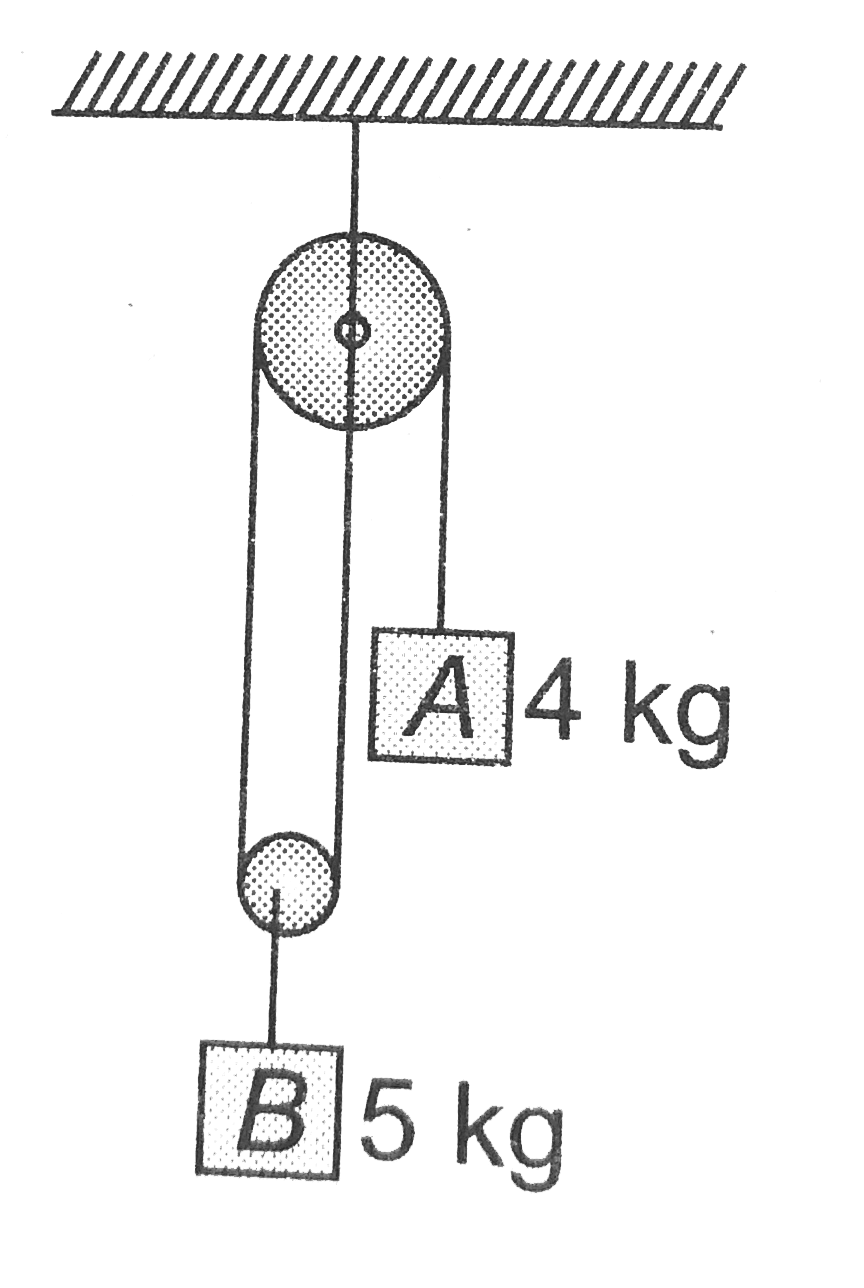
View Solution