A circular hoop of mass m and radius R rests flat on a horizontal smooth surface. A bullet of same mass m and moving with velocity υ strikes the hoop tangentially and gets embedded in it. Neglect the thickness of hoop in comparision to radius of hoop.

Mark the correct option
energy of system (hoop+ bullet) is conserved
momentum of system is conserved
angular momentum of system is conserved about any point in the plane of hoop
angular momentum is not conserved due to torque of gravitational force.
The correct Answer is:C
Let velocity of CM of hoop be V1 and angular velocity of hoop be ω, resultant velocity of bullet just after so collision is V1+Rω
From conservation of momentum, we get
Mυ0=mV1+m(V1+ωR)...(1)
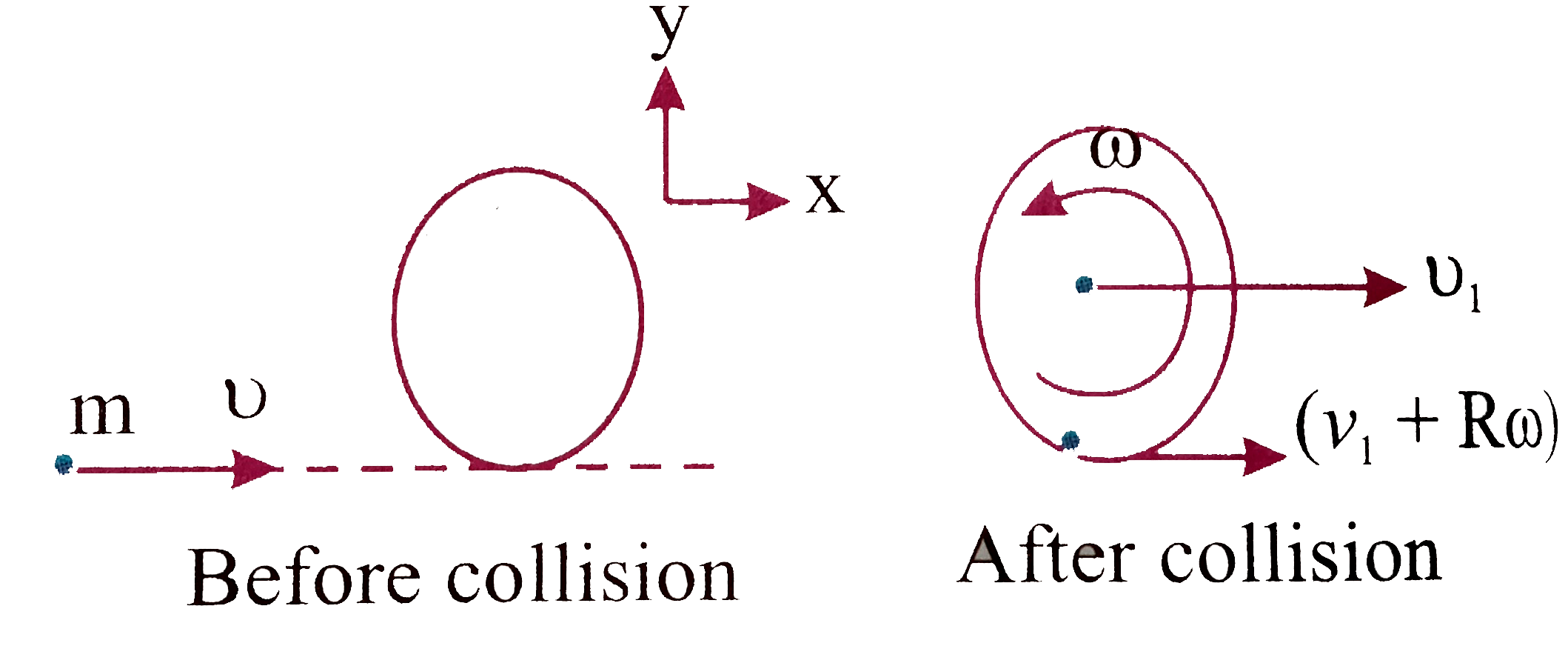
From conservation of angular momentum about O,
Which is a point on ground just below the point of impact
Li=0
Lf=−mV1R+mR2ω
Li=Lf
V1=Rω
from eqns (1) and (2), we get
ω=υ03R and V1=υ03
Absolute velocity of bullet=V1+Rω=2υ03
Impulse on bullet
=4→P=2mυ03ˆi−mυ0ˆi=−mυ03i
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-VI
Explore 78 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level -II(H.W)
Explore 47 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
A circular wooden hoop of mass m and radius R rests fiat on a frictionless surface. A bullet, also of mass m and moving with a velocity v, strikes the hoop and gets embedded in it. The thickness of the hoop is much smaller than R. Find the angular velocity with which the system rotates in after the bullet strikes the hoop.
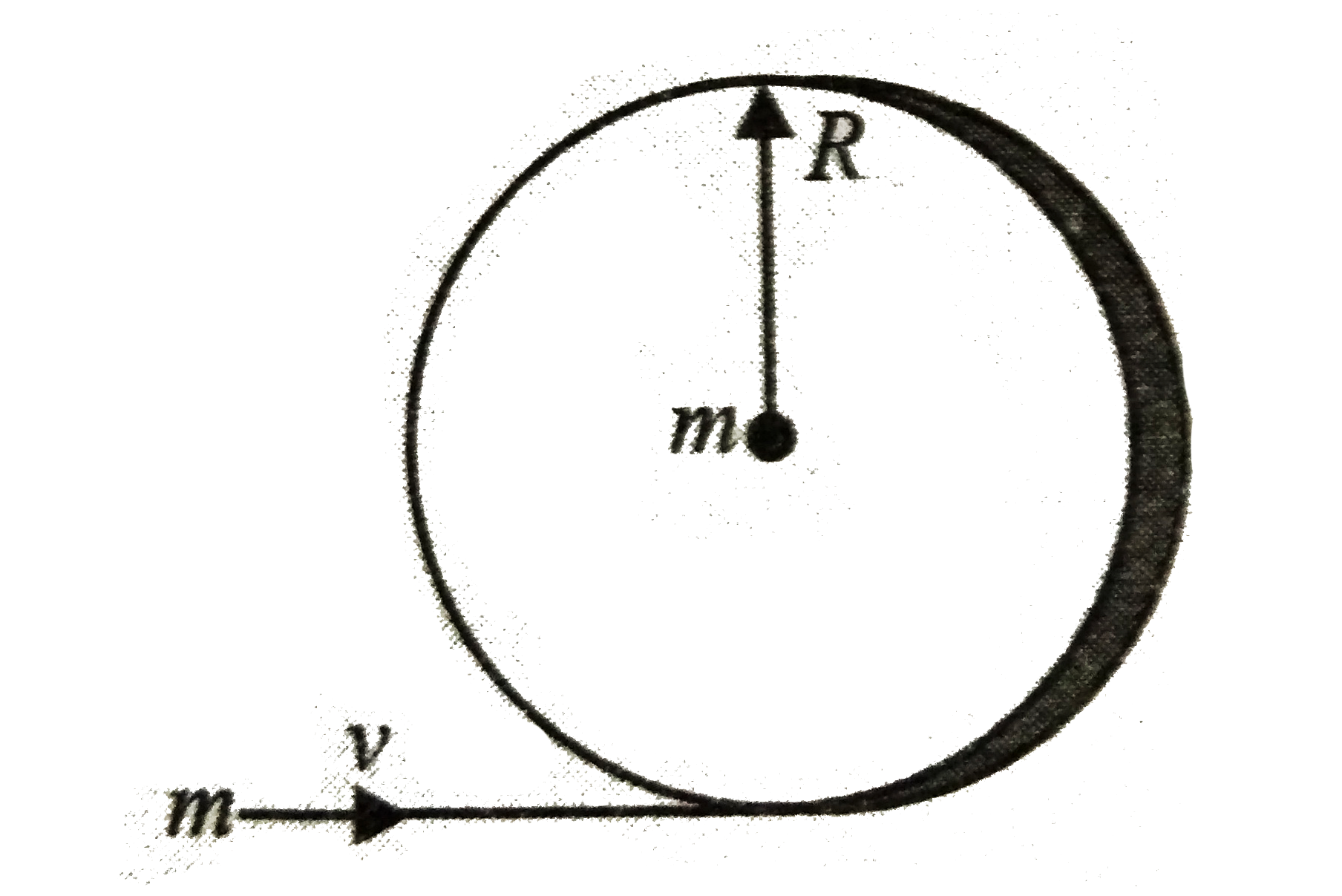
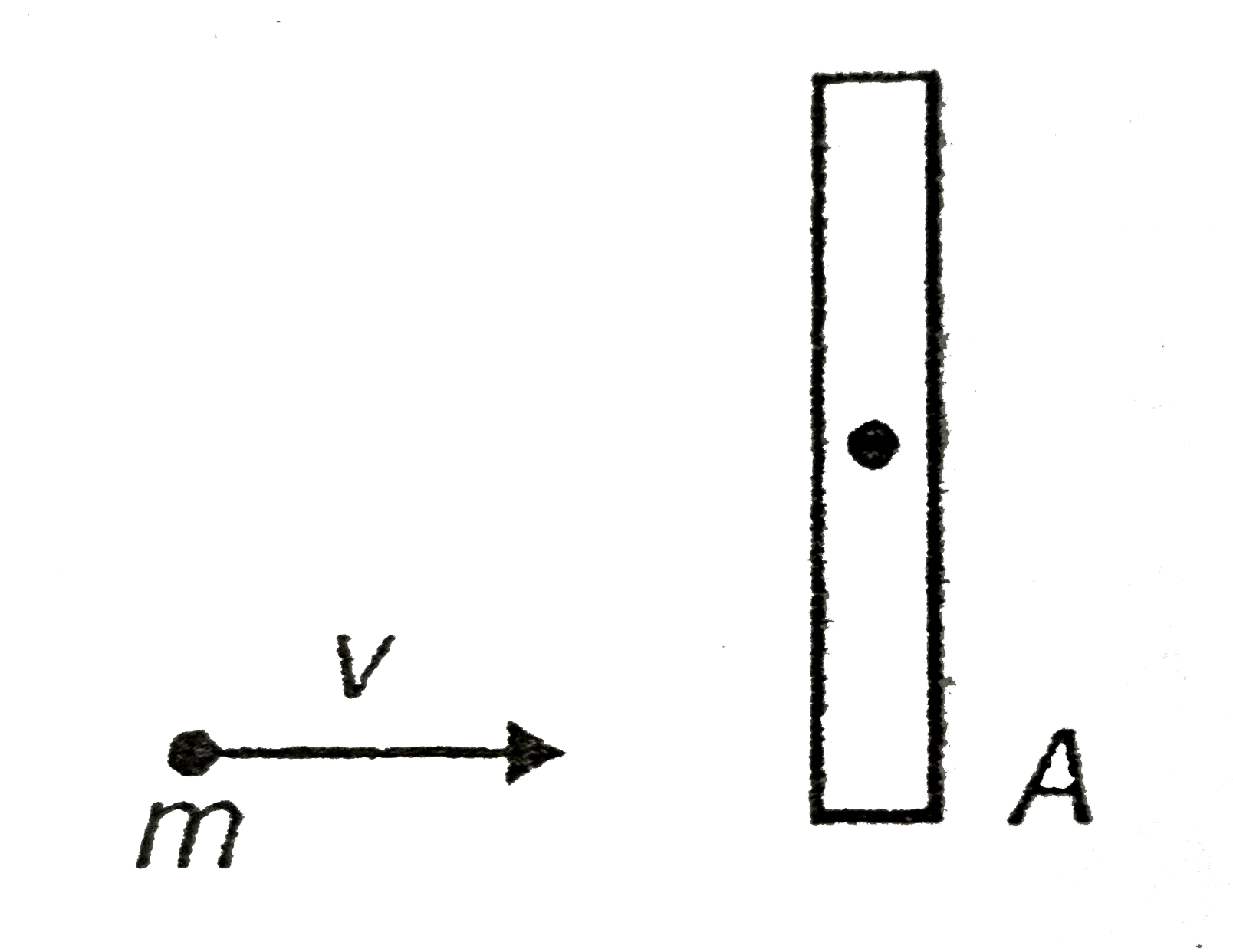
View SolutionA rod of mass m and length l hinged at the centre is placed on a horizontal surface. A bullet of mass m moving with velocity v strikes the end. A of the rod and gets embedded in it. The angular velocity with which the systems rotates about its centre of mass after the bullet strikes the rod
View Solution
Knowledge Check
A circular hoop of mass m and radius R rests flat on a horizontal smooth surface. A bullet of same mass m and moving with velocity υ strikes the hoop tangentially and gets embedded in it. Neglect the thickness of hoop in comparision to radius of hoop.

What is the impulse on bullet?A−2μψlon03ˆiB−μψlon03ˆiCmυ0ˆiDmυ02ˆiA circular hoop of mass m and radius R rests flat on a horizontal smooth surface. A bullet of same mass m and moving with velocity υ strikes the hoop tangentially and gets embedded in it. Neglect the thickness of hoop in comparision to radius of hoop.
Velocity of CM of hoop (ground frame)isAυ03B2υ03Cυ02Dυ04A circular hoop of mass m and radius R rests flat on a horizontal smooth surface. A bullet of same mass m and moving with velocity υ strikes the hoop tangentially and gets embedded in it. Neglect the thickness of hoop in comparision to radius of hoop.
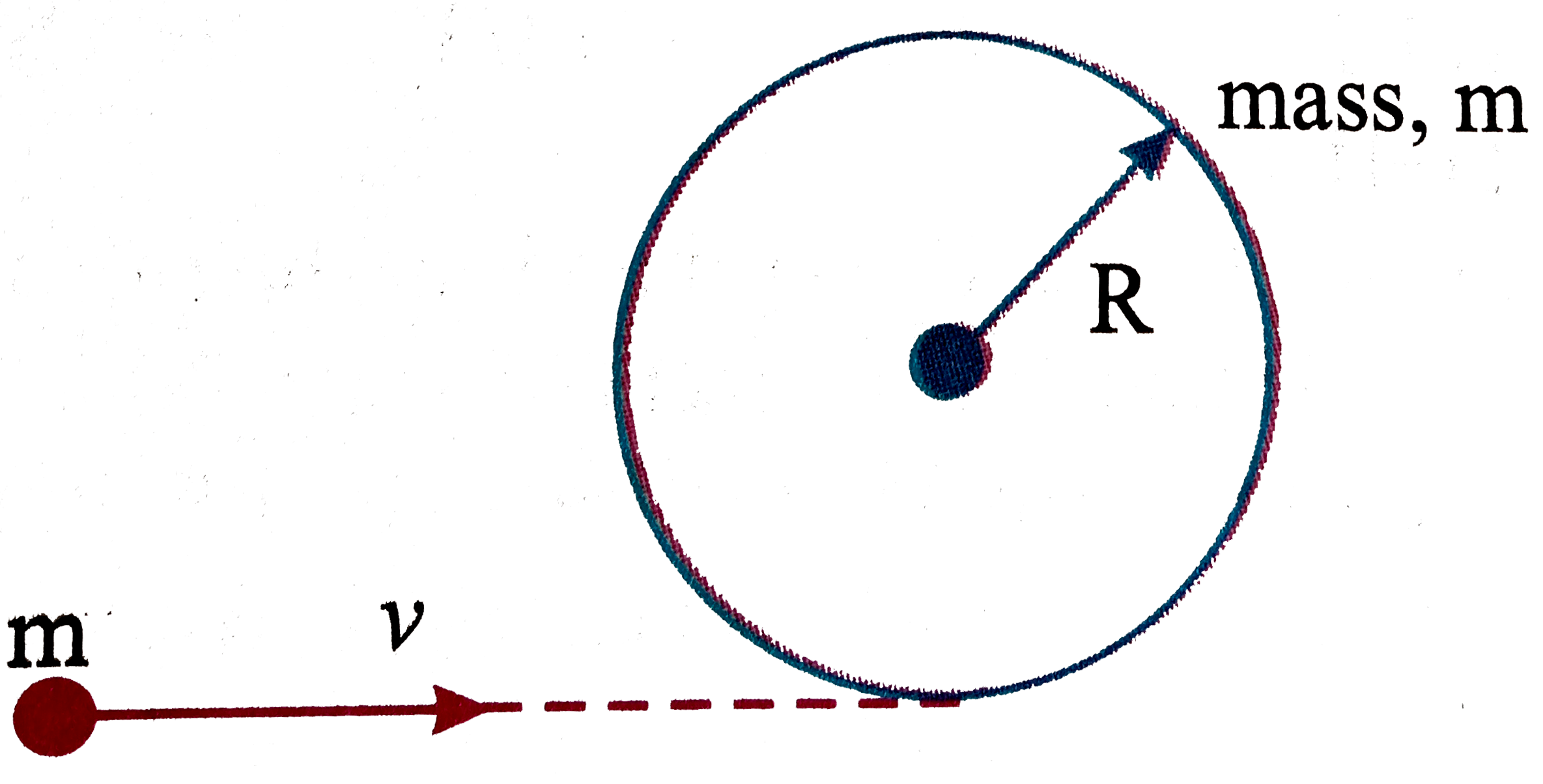
Velocity of bullet in ground frame, after collision isA2υ03Bυ03Cυ02Dυ04
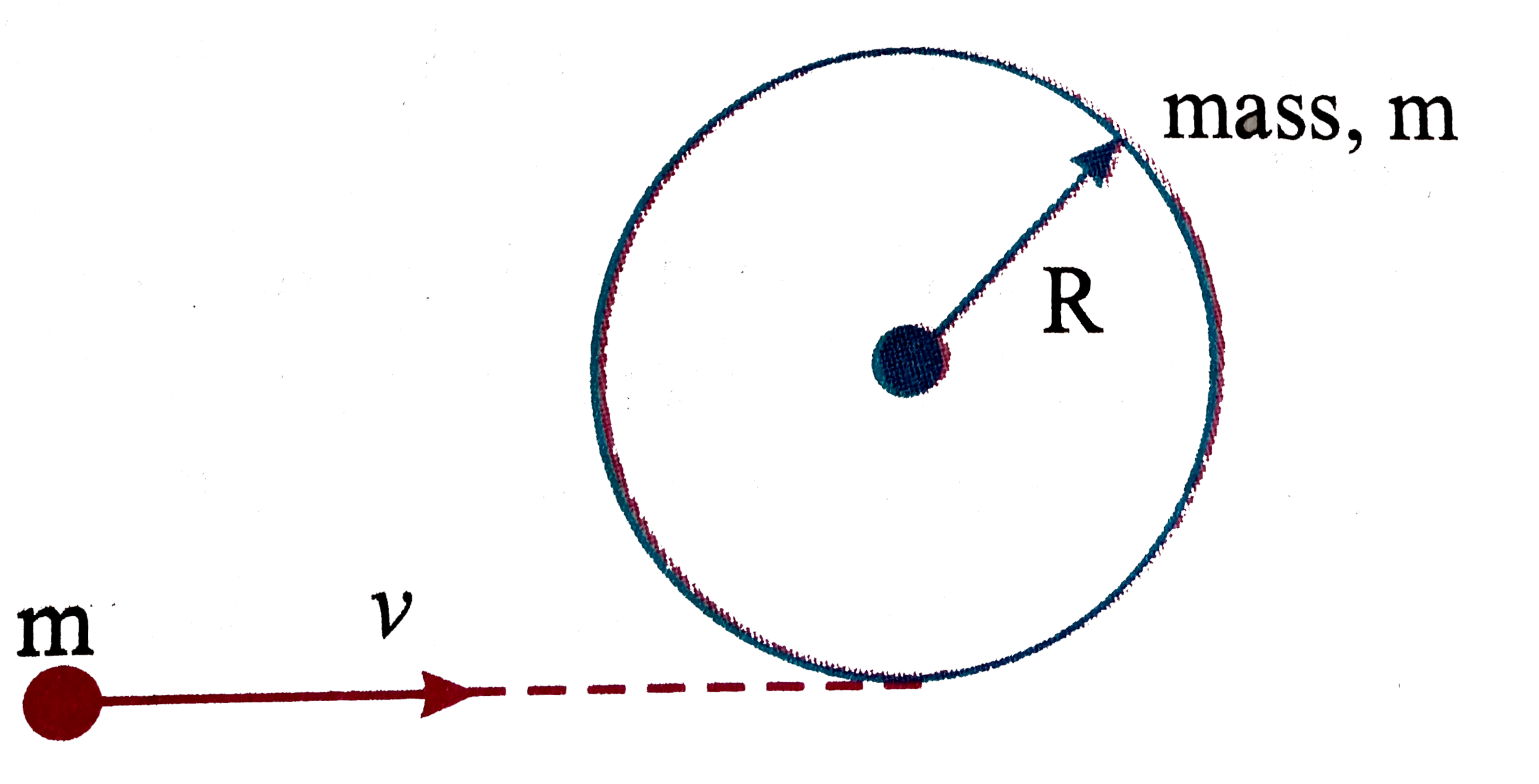
A circular hoop of mass m and radius R rests flat on a horizontal frictionless surface A bullet also of mass m and moving with a velocity v strikes the hoop and gets embedded in it the thickness of the hoop is much smaller then R the angular velocity with which the system rotates after the bullet strikes the hoop is
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View Solution
A circular ring of mass m and radius R rests flat on a horizontal smooth surface as shown in figure. A particle of mass m, and moving with a velocity v. Collides inelastically (e=0) with the ring the angular velocity with which the system rotates after the particle strikes the ring is
View SolutionA bullet of mass m moving with a speed v strikes a wooden block of mass M & gets embedded into the block. The final speed is
View Solution