Moment of inertia of a hoop suspended from a peg about the peg is
MR2
MR22
2MR2
3MR22
The correct Answer is:C
To find the moment of inertia of a hoop suspended from a peg about the peg, we can follow these steps:
Step 1: Understand the System
A hoop is a circular object with mass distributed uniformly along its circumference. When suspended from a peg, it can rotate about the peg.
Step 2: Define the Parameters
Let:
- R = radius of the hoop
- m = mass of the hoop
Step 3: Use the Formula for Moment of Inertia
The moment of inertia I of a hoop about its center is given by:
Icenter=mR2
Step 4: Apply the Parallel Axis Theorem
Since the hoop is rotating about a peg (which is not at its center), we need to use the parallel axis theorem to find the moment of inertia about the peg. The parallel axis theorem states:
I=Icenter+md2
where d is the distance from the center of mass to the new axis (the peg).
Step 5: Calculate the Distance d
In this case, the distance d from the center of the hoop to the peg is equal to the radius R of the hoop.
Step 6: Substitute Values into the Formula
Now substituting the values into the parallel axis theorem:
I=mR2+mR2=2mR2
Step 7: Conclusion
Thus, the moment of inertia of the hoop about the peg is:
I=2mR2
Final Answer
The moment of inertia of a hoop suspended from a peg about the peg is 2mR2.
---
A hoop is a circular object with mass distributed uniformly along its circumference. When suspended from a peg, it can rotate about the peg.
Let:
- R = radius of the hoop
- m = mass of the hoop
The moment of inertia I of a hoop about its center is given by:
Icenter=mR2
Since the hoop is rotating about a peg (which is not at its center), we need to use the parallel axis theorem to find the moment of inertia about the peg. The parallel axis theorem states:
I=Icenter+md2
where d is the distance from the center of mass to the new axis (the peg).
In this case, the distance d from the center of the hoop to the peg is equal to the radius R of the hoop.
Now substituting the values into the parallel axis theorem:
I=mR2+mR2=2mR2
Thus, the moment of inertia of the hoop about the peg is:
I=2mR2
The moment of inertia of a hoop suspended from a peg about the peg is 2mR2.
Topper's Solved these Questions
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level -II(H.W)
47 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-V
72 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - NCERT based questions
15 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV
39 Videos
Similar Questions
Knowledge Check
A hoop of mass 500gm & radius 10cm is placed on a nail, then the moment of inertia of the hoop, when it is rotated about the nail will be ____kgm2
A0.05B0.02C0.01D0.03Moment of inertia of a disc of mass 'M' and radius 'R' about the diameter is
AMR22BMR24CMR23DMR212The moment of inertia of a sphere is 20 kg-m2 about the diameter. The moment of inertia about any tangent is
A25Kgm2B50kgm2C70kgm2D80kgm2
Calculate the moment of inertia of each particle in Fig. about the indicated axis of rotation.

View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be :-

View SolutionWhat is the moment of inertia of a uniform circular ring about its diameters ?
View SolutionCalculate the moment of inertia of the given rod about an axis CD
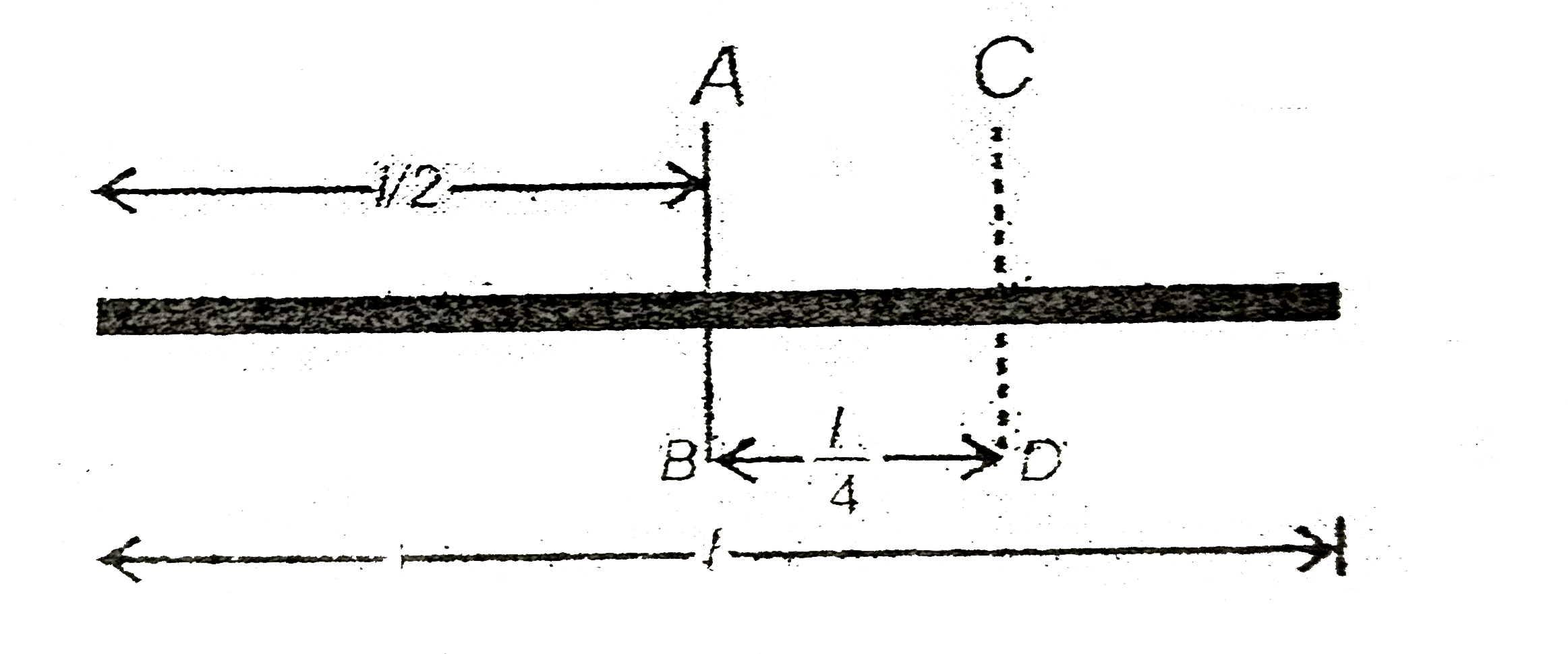
View SolutionThe moment of inertia of a soild sphere about a tangent is 53mr2. What would be its moment of inertia about its diameter.
View Solution
