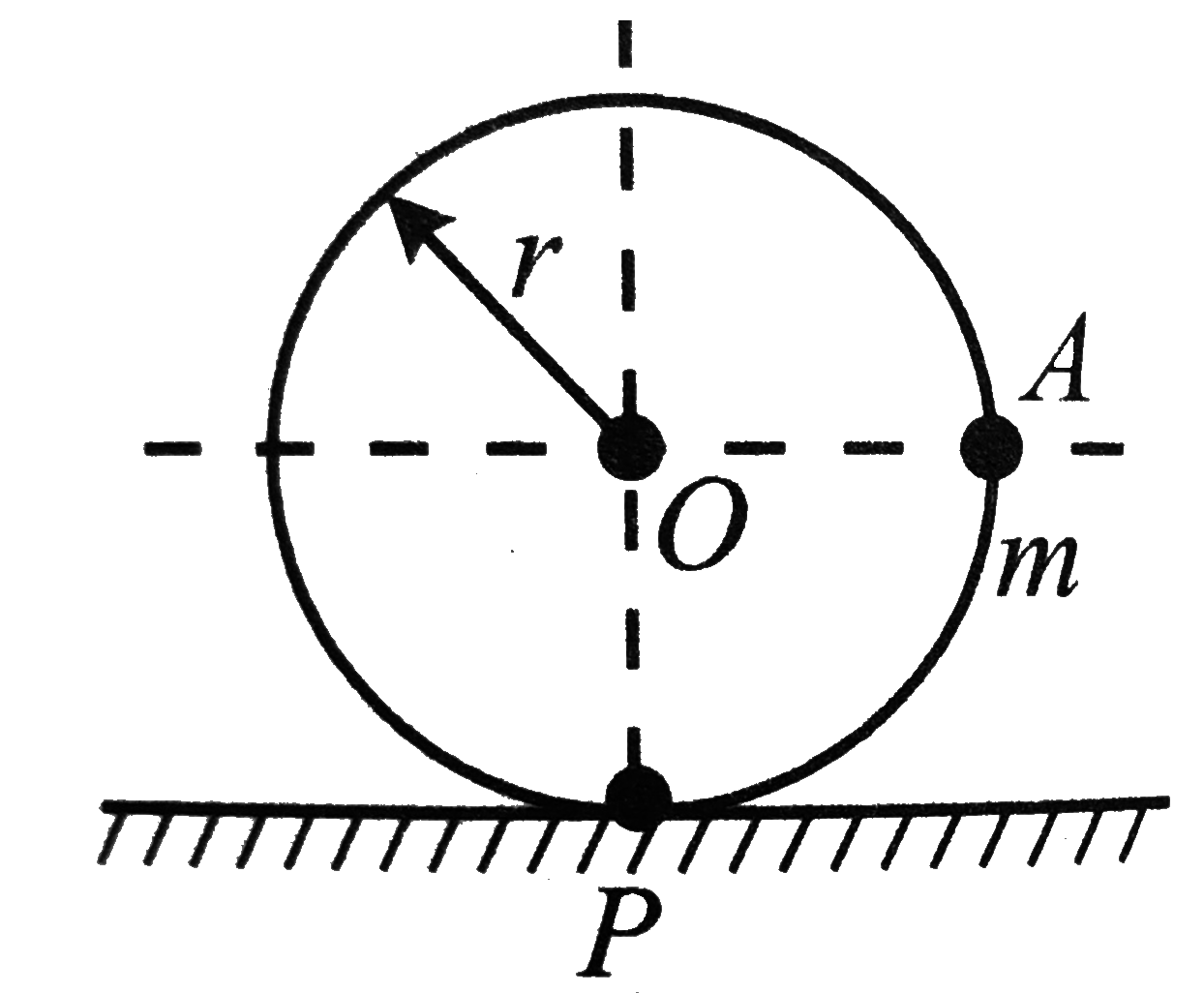
A particle of mass 'm' is rigidly attached at 'A' to a ring of mass 3m and radius 'r' . The system is released from rest and rolls without sliding. The angular release is acceleration of ring just after release after
g4r
g6r
g8r
g2r
The correct Answer is:B
The distance of CM from the ring centre O
x=3m(0)+m(r)3m+m=r4
we can apply torque equation about point of contanct as the ring is rolling
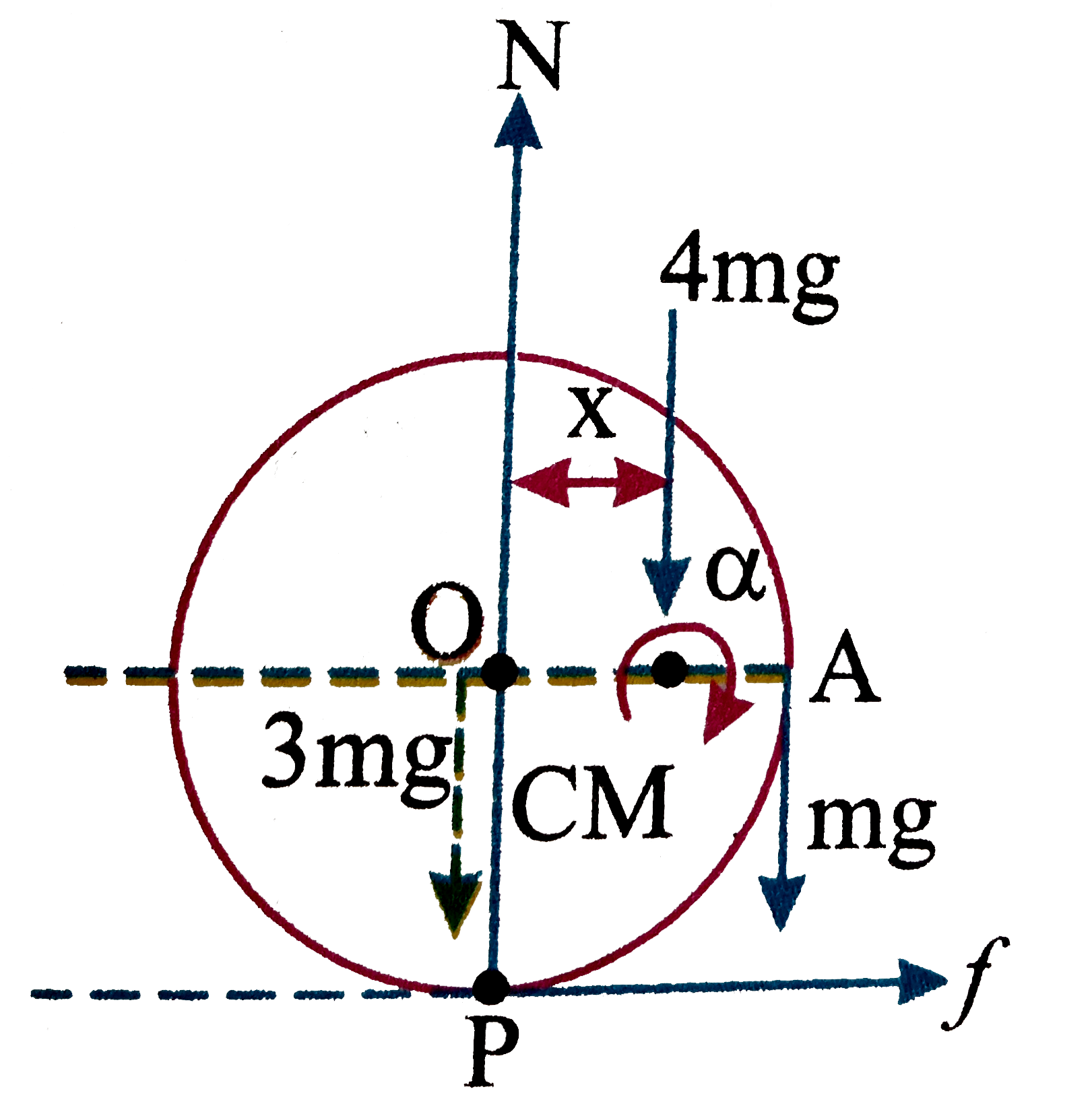
4mg(r4)=[(3mr2+mr2)+m(AP)2]α
⇒mgr=[4mr2+m(√2r)2]α
⇒mgr=6mr2α⇒a=g6r
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:NCERT based questions
Explore 15 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level -I(H.W)
Explore 58 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-II(C.W.)
Explore 64 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
Knowledge Check
A particle of mass 'm' is rigidly attached at 'A' to a ring of mass 3m and radius 'r' . The system is released from rest and rolls without sliding. The angular release is acceleration of ring just after release after
 Ag4rBg6rCg8rDg2r
Ag4rBg6rCg8rDg2rA stone is released from a rising balloon accelerating upward with acceleration a. The acceleration of the stone just after the release is
Aa upwardBg downwardC(g−a) downwardD(g+a) downwardThe total energy of rolling ring of mass m and radius R is
A3/2mv2B1/2mv2Cmv2D5/2mv2
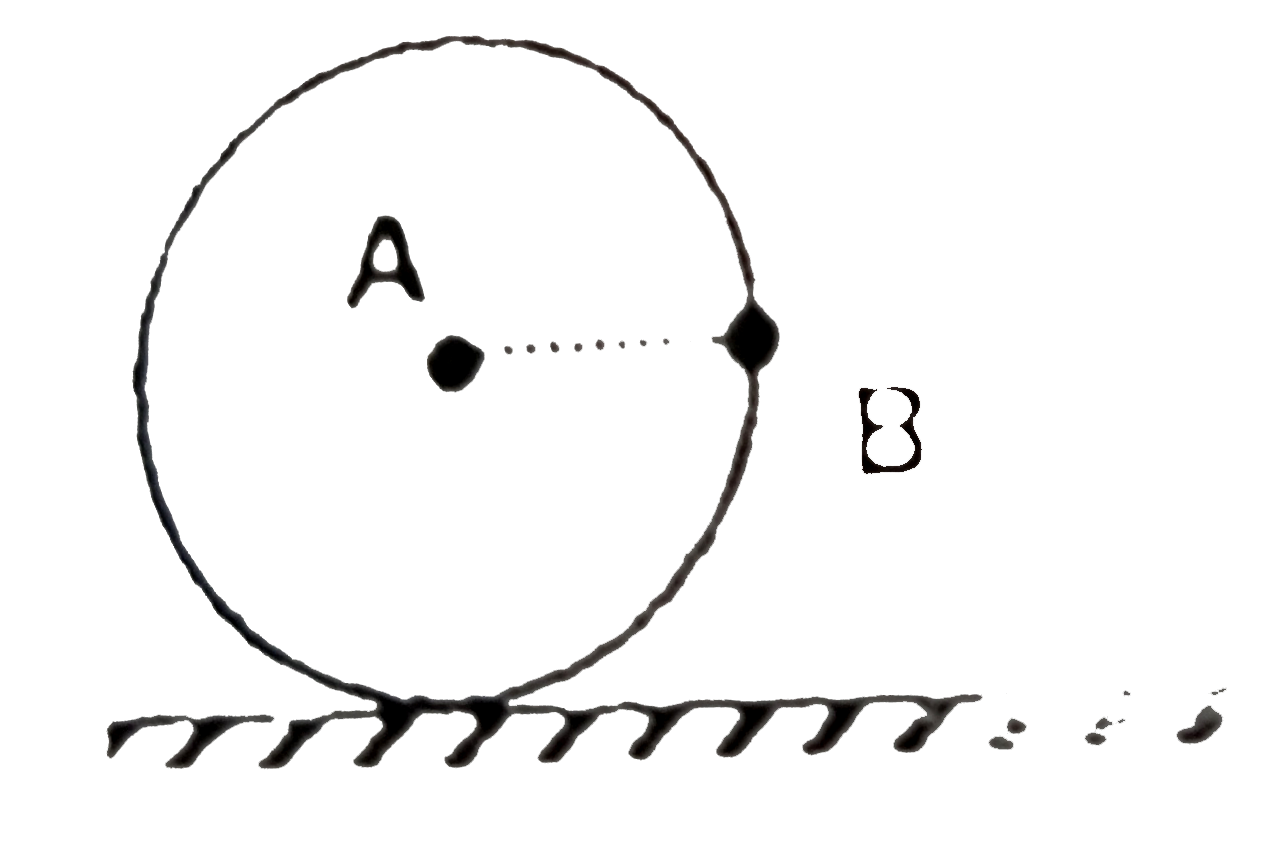
A small particle of mass m is attached at B to a hoop of mass m and radius r, whole system is placed on the rough horizontal ground. The system is released from rest when B is dierctly above A and rolls without slipping. Find the angular acceleration of the system at the instant when AB becomes horizontal as shown in the figure.
View SolutionA ring of mass m and radius R is placed on a rough inclined plane so that it rolls without slipping . Match the following table.



View SolutionIn the system shown, the masses are released from rest. What shall be the acceleration of the moving masses?
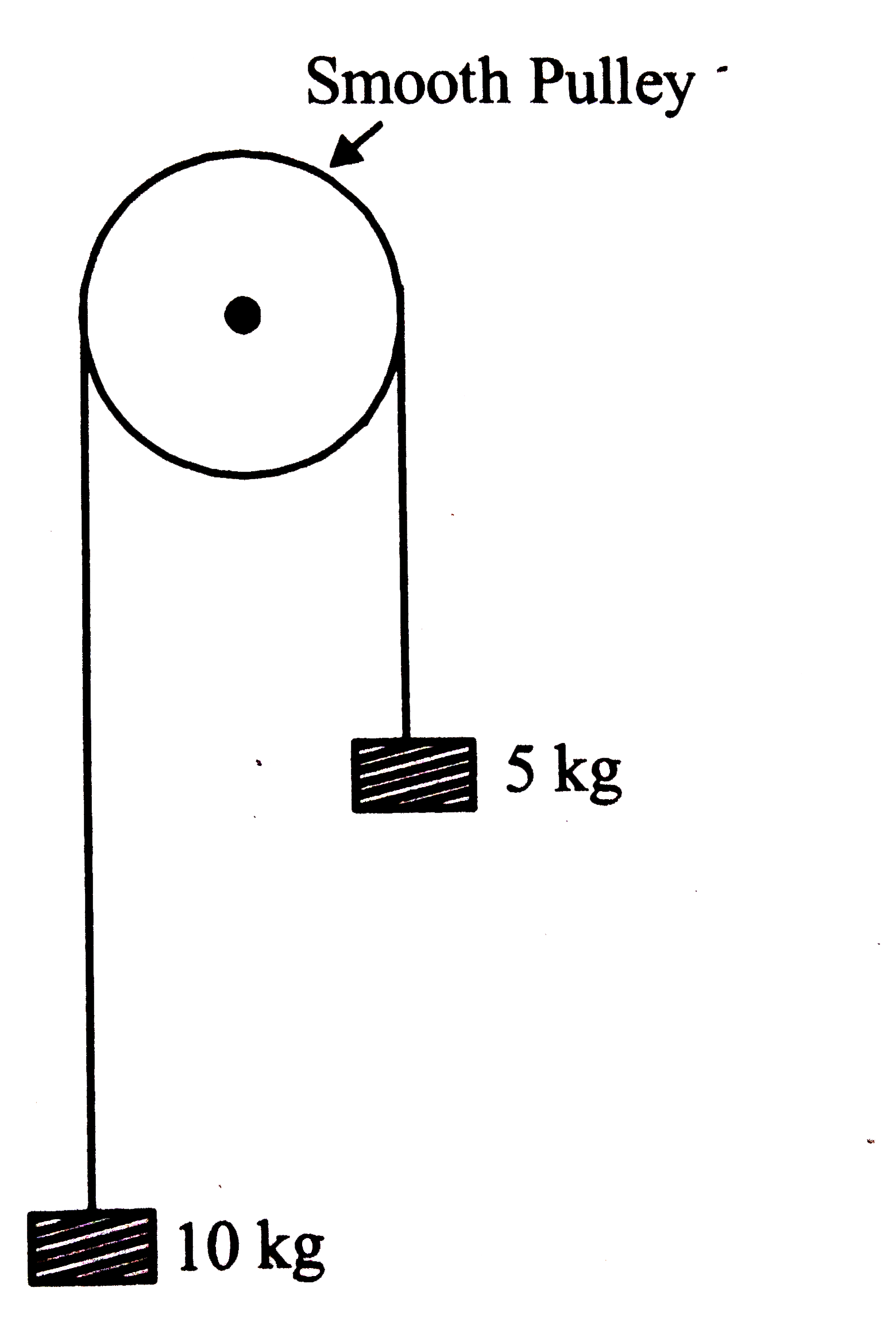
View SolutionA half section of thin circular pipe of mass m and radius 'r' is released from rest in the position shown. Pipe rolls without sliding. The change in potential energy of pipe after it has rolled through 90∘ :
View SolutionA disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:

View Solution