Four holes of radius R are cut from a thin square plate of side 4R and mass M. The moment of inertia of the remaining portion about z-axis is :
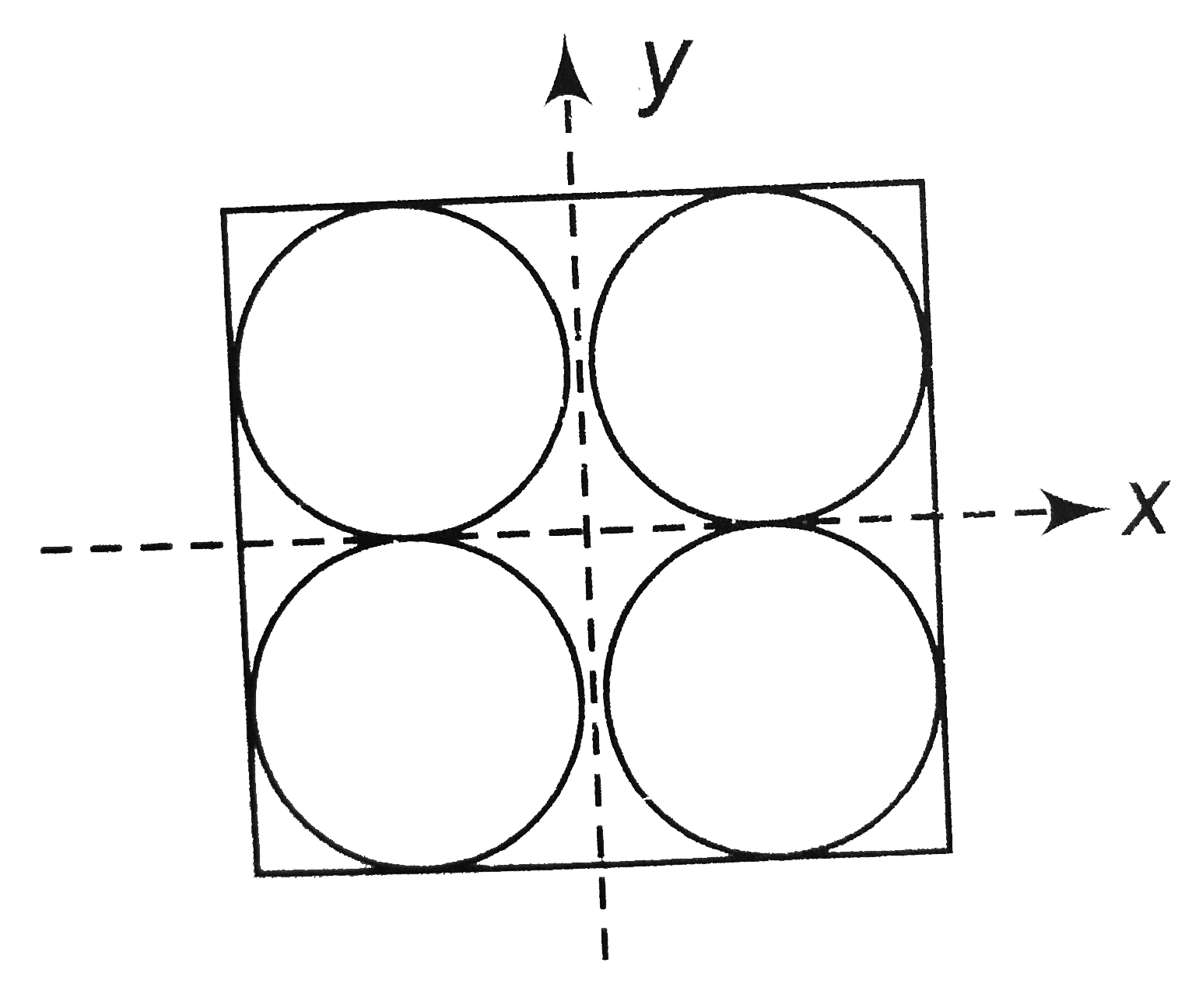 .
.
M is the mass of the square plate before cutting the holes.
Mass of one hole m=[M16R2]πR2=π16M
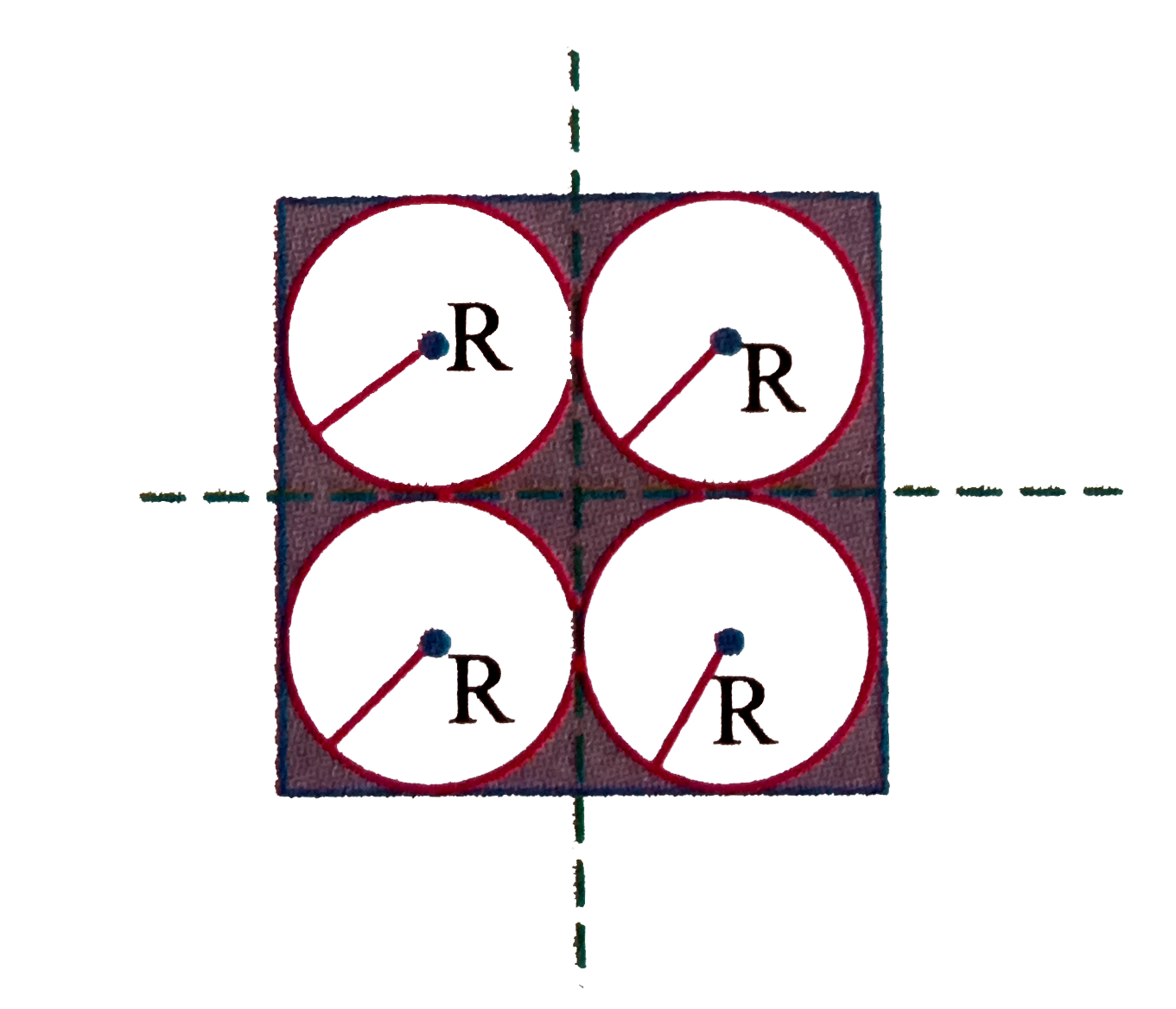
Moment of inertia of remaining portion
I=Isquare−4lhole
I=M12[16R2+16R2]−4[mR22+m(2R2)]
=83MR2−10mR2=(83−10π16)MR2
Similar Questions
Knowledge Check
A concentric hole of radius R/2 is cut from a thin circular plate of mass M and radius R. The moment of inertia of the remaining plate about its axis will be
A1324MR2B1124MR2C1332MR2D1532MR2A concentric hole of radius R/2 is cut from a thin circular plate of mass M and radius R. The moment of inertia of the remaining plate about its axis will be
A1324MR2B1124MR2C1332MR2D1532MR2A hole of radius R/2 is removed from a thin circular plate of radius R and mass M. The moment of inertia of the remaining plate about an axis through centre O and perpendicular to the plane of the plate is
 A3MR232B3MR213C1332MR2D3213MR2
A3MR232B3MR213C1332MR2D3213MR2
A disc has mass 9 m. A hole of radius R/3 is cut from it as shown in the figure. The moment of inertia of remaining part about an axis passing through the centre 'O' of the disc and perpendicular to the plane of the disc is :

View SolutionA circular of radius R has mass 9m . A hole of radius R3 is cut from it as shown in figure. The moment of inertia of the remaining part about an axis passing through centre 0 of the disc and perpendicular of the plane of disc is xmR2 .Find value of x
View SolutionA square plate of side / has mass M. What is its moment of inertia about one of its diagonals ?
View Solution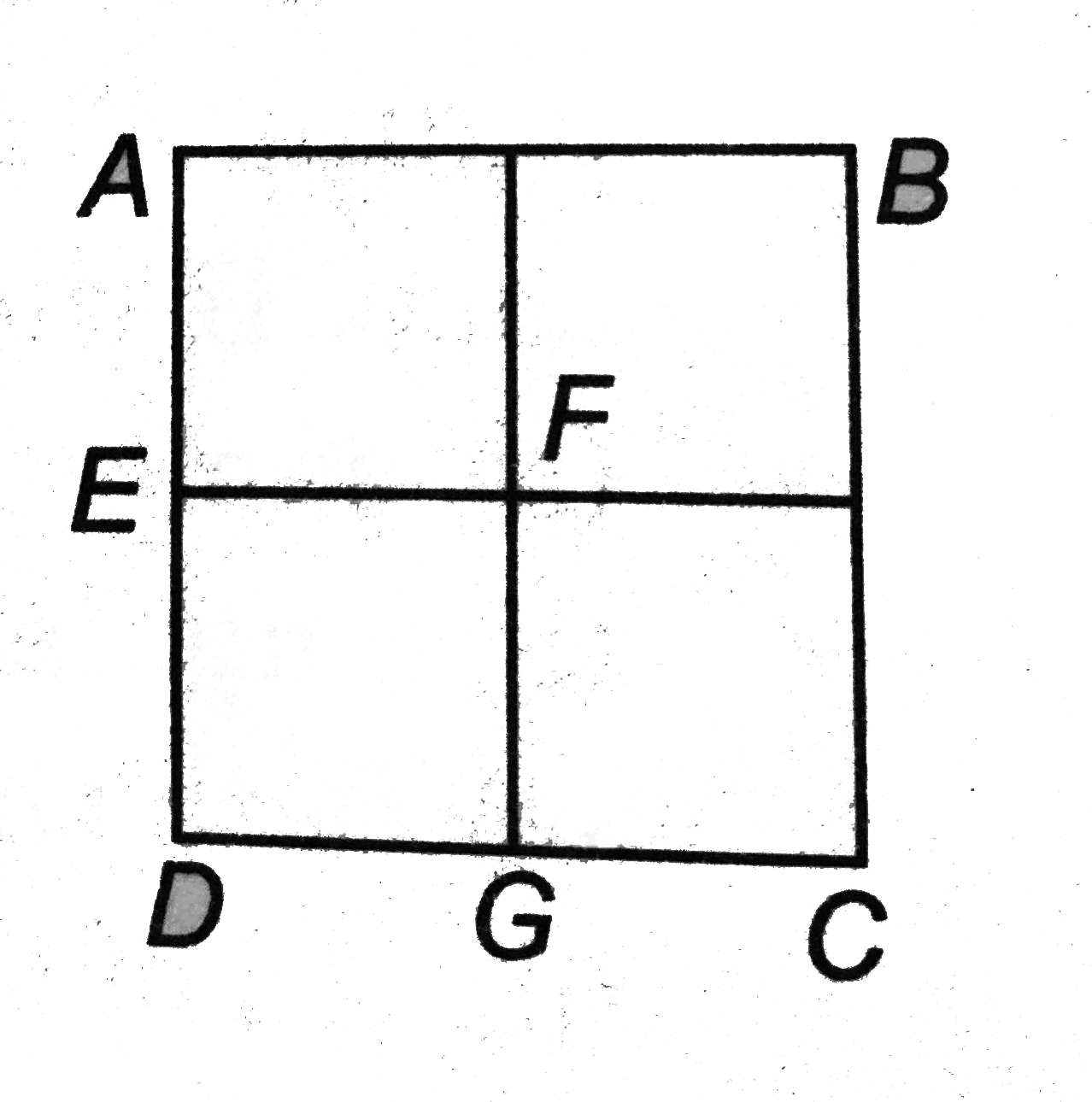
From a uniform square plate of side a and mass m, a square portion DEFG of side a2 is removed. Then, the moment of inertia of remaining portion about the axis AB is
View SolutionA hole of radius R/2 is cut from a thin circular plate of raduis R as shown in the figure. If the mass of the remaining plate is M, then find moment of inertia of the plate about an axis through O perpendicular to plane

View Solution

