A book of weight 20N is pressed between two hands and each hand exerts a force of 40N. If the block just starts to slide down Coefficient of friction is .
0.25
0.2
0.5
0.1
The correct Answer is:A
To solve the problem, we need to determine the coefficient of friction when a book weighing 20 N is pressed between two hands, each exerting a force of 40 N, and the book just starts to slide down.
1. Identify the Forces Acting on the Book:
- The weight of the book (W) is given as 20 N.
- Each hand exerts a force (F) of 40 N, resulting in a total normal force (N) acting on the book from both hands.
2. Calculate the Total Normal Force:
- Since there are two hands, the total normal force acting on the book is:
N=F1+F2=40N+40N=80N
3. Understand the Frictional Forces:
- The frictional force (f) that opposes the motion of the book when it starts sliding is given by:
f=μ⋅N
- Here, μ is the coefficient of friction.
4. Set Up the Equation for Sliding:
- When the book just starts to slide down, the total frictional force must equal the weight of the book:
f=W
- Thus, we have:
μ⋅N=W
5. Substituting Known Values:
- Substitute N=80N and W=20N into the equation:
μ⋅80N=20N
6. Solve for the Coefficient of Friction:
- Rearranging the equation gives:
μ=20N80N=14=0.25
Conclusion:
The coefficient of friction (μ) is 0.25.
- The weight of the book (W) is given as 20 N.
- Each hand exerts a force (F) of 40 N, resulting in a total normal force (N) acting on the book from both hands.
- Since there are two hands, the total normal force acting on the book is:
N=F1+F2=40N+40N=80N
- The frictional force (f) that opposes the motion of the book when it starts sliding is given by:
f=μ⋅N
- Here, μ is the coefficient of friction.
- When the book just starts to slide down, the total frictional force must equal the weight of the book:
f=W
- Thus, we have:
μ⋅N=W
- Substitute N=80N and W=20N into the equation:
μ⋅80N=20N
- Rearranging the equation gives:
μ=20N80N=14=0.25
The coefficient of friction (μ) is 0.25.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:LEVEL -II (C.W)
Explore 71 Videos
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:LEVEL -III
Explore 31 Videos
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:C.U.Q
Explore 73 Videos
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Level 2 H.W
Explore 29 Videos
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EXERCISE - IV
Explore 41 Videos
Similar Questions
A student holds a book of mass 2kg between his hands.Each hand exerts horizontally a force of 50N .Then the coefficient of static friction is
View SolutionA block of mass 1kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5 ,magnitude of the frictional force acting on the block is
View Solution
Knowledge Check
A book of weight 20N is pressed between two hands and each hand exerts a force of 40N. If the book just starts to slide down. Coefficient of friction is
A0.25B0.2C0.5D0.1A20 kg block is initally at rest. A 75 N force is required to set the block in motion. After the motion starts, a force of 60 N is required to keep the block moving with constant speed. The coefficient of static friction is
A0.52B0.44C0.6D0.38A block of mass 10 kg is placed on an inclined plane when the angle of inclination is 30∘. The block just begins to slide down the plane. The force of static friction is
A10 kg wtB89 kg wC49 kg wtD5 kg wt
A block of 50 N is pressed normally against a wall as shown. The frictional force acting on the block is :
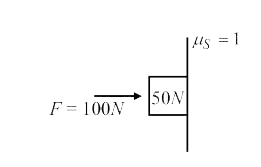 ]
]
View SolutionA man holds a 2 kg book between his palms so that each hand exerts the same horizontal force on the book.The coefficient of static friction between the palms and the book is 0.4 and g= 10ms−2 .If the book is prevented from falling,the least force exerted by each hand on the book is ?
View SolutionA block of weight 30 N is in contact with a rough wall. A force of 50 N is applied as shown in the figure. Find the coefficient of static friction between the wall and the block assuming the block is just about to slip.
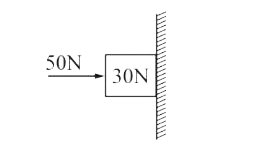
View SolutionA block of weight 10 N lies on a rough table. It just moves when a force of 7N is applied on it. Calculate the coefficient of limiting friction between the block and the table.
View SolutionGiven in figure are two blocks A and B of weight 20N and 100N, respectively. These are being pressed against a wall by a force F as shown. If the coefficient of friction between the blocks is 0.1 and between block B and the wall is 0.15, the frictional force applied by the wall on block B is:
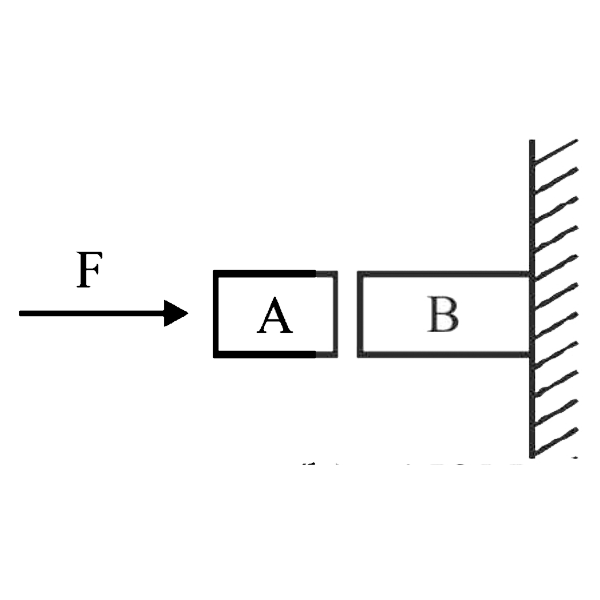
View Solution