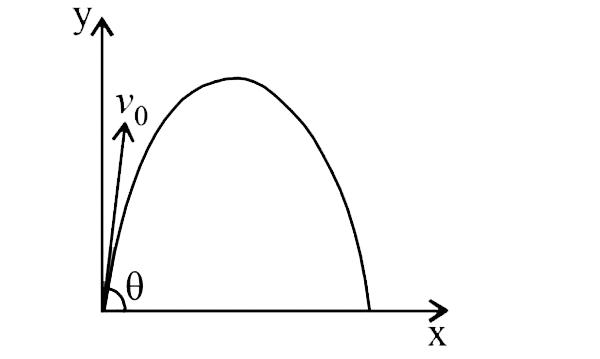
A small particle of mass m is projected at an angle θ with the x- axis with an initial velocity v0 in the x−yplane as shown in the figure . At a time t<v0sinθg, the angular momentum of the particle is
where ˆi,ˆjandˆk are unit vectors along x,yandz - axis respectively.
12mgv0t2cosθˆi
−mgv0t2cosθˆj
mgv0t2cosθˆk
−12mgv0t2cosθˆk
t<v0sinθg
→v=v0cosθˆi+(v0sinθ−gt)ˆj
→r=xˆi+yˆj=v0cosθtˆi+(v0sinθt−12gt2)ˆj
→l0=→r×m→v=m(→r×→v)
=−12mgv0t2cosθˆk
Topper's Solved these Questions
Similar Questions
A small particle of mass m is projected at an angle θ with x-axis with initial velocity υ0 in x-y plane as shown in Fig. Calculate the momentum of the particle
at t<υ0sinθg.
 View Solution
View SolutionA small particle of mass m is projected at an angle θ with the x-axis, with initial velocity v0 in the x-y plane. Just before time 2v0sinθg , the angular momentum of the particle about the point of projection is
View Solution
Knowledge Check
- Question 1 - Select One
The angle which the vector →A=2ˆi+3ˆj makes with the y-axis, where ˆi and ˆj are unit vectors along x- and y-axis, respectively, is
Acos−1(3/5)Bcos−1(2/3)Ctan^(-1)(2//3)Dsin−1(2/3) - Question 2 - Select One
The angle θ between the vector →p=ˆi+ˆj+ˆk and unit vector along X-axis is
Acos−1(1√3)Bcos−1(1√2)Ccos−1(√32)Dcos−1(12) - Question 3 - Select One
If θ is the angle between the vectors 4(ˆi−ˆk)andˆi+ˆj+ˆk, then what is (sinθ+cosθ) equal to ?
A0B12C1D2
A particle moves in x-y plane according to the equation ¯r=(ˆi+2ˆj)Acosωt the motion of the particle is
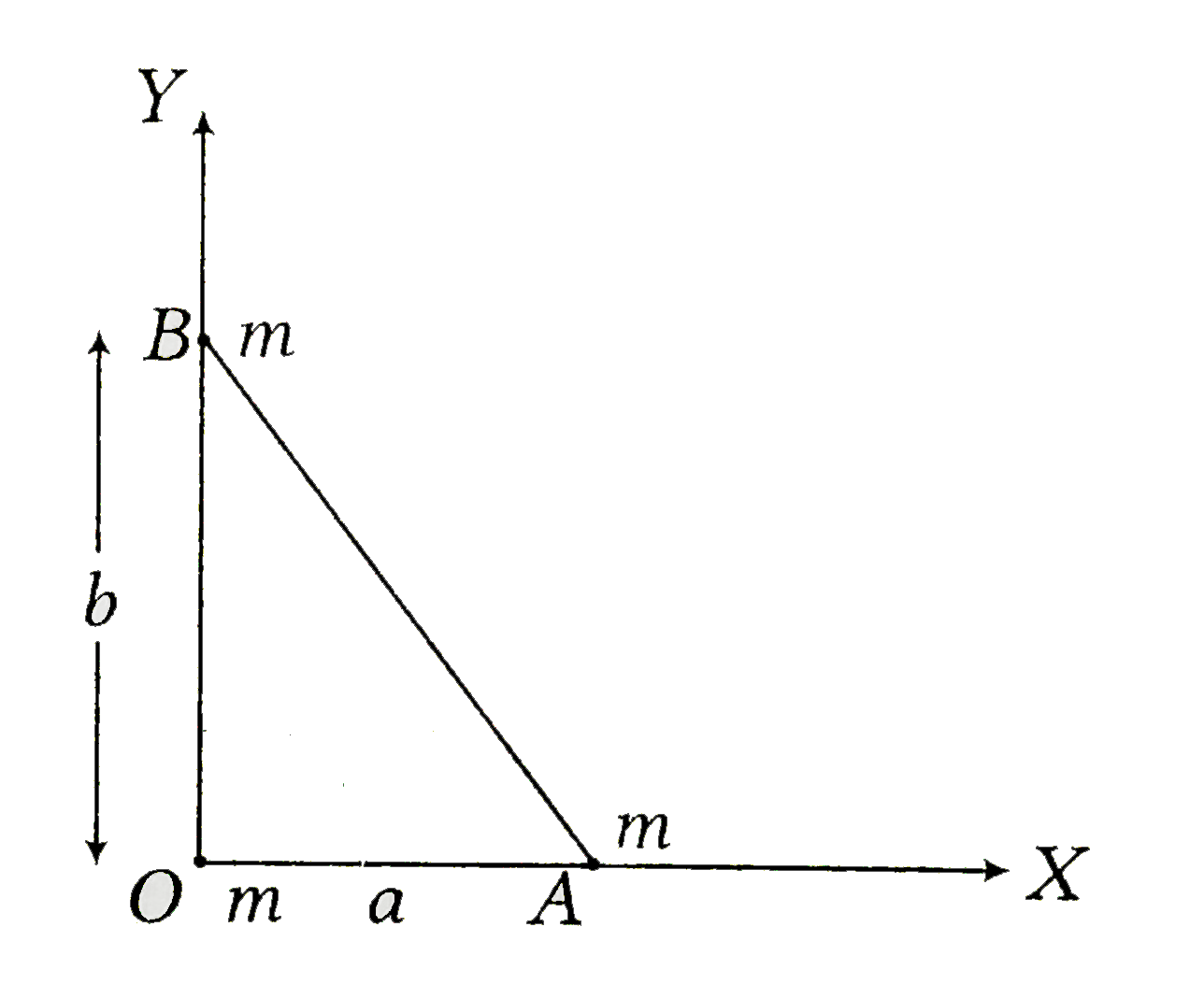
View SolutionThree particles , each of mass m, are placed at the vertices of a right angled triangle as shown in figure. The position vector of the center of mass of the system is (O is the origin and ˆi,ˆj,ˆk are unit vectors)
 View Solution
View SolutionThe unit mass having r=8ˆi−4ˆjandv=8ˆi+4ˆj in its angular momentum is
View SolutionA particle of mass m moves in the xy-plane with velocity of →v=vxˆi+vyˆj. When its position vector is →r=x→i+y→j, the angular momentum of the particle about the origin is.
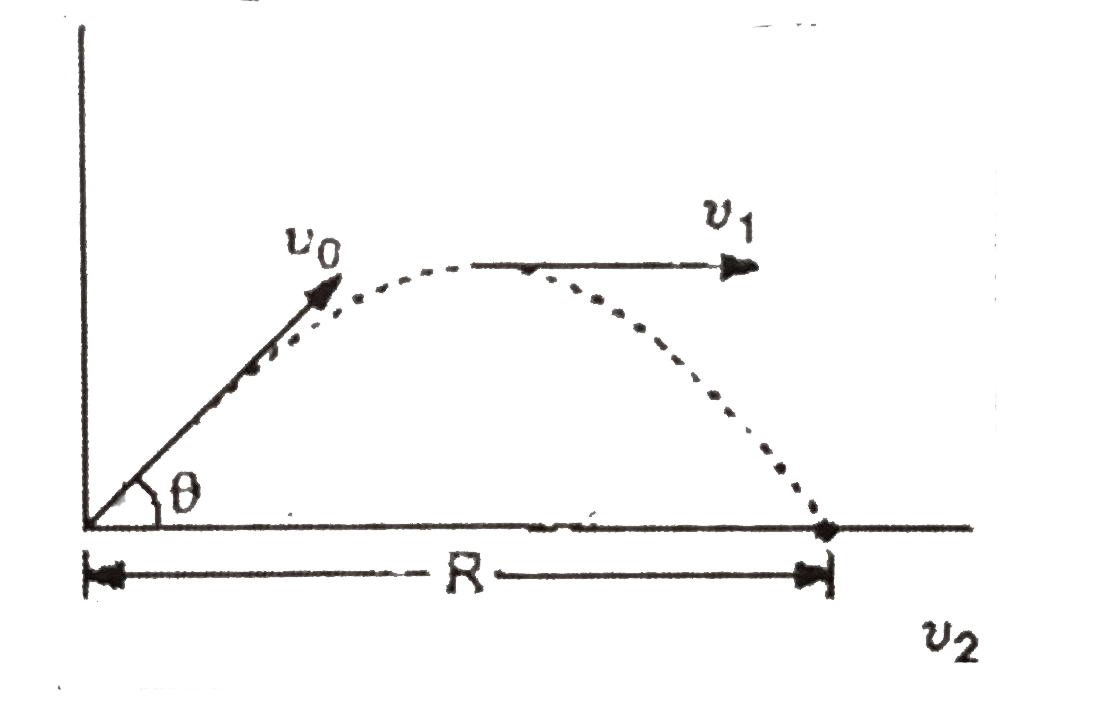
View SolutionA projectile is projected with a velocityv0 at an angle θ with the horizontal as shown in figure. The angular momentum of particle about the origin:
 View Solution
View Solution

