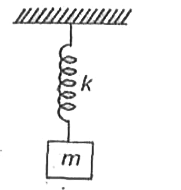
In figure, the stiffness of the spring is k and mass of the block is m. The pulley is fixed. Initially, the block m is held such that the elongation in the spring is zero and then released from rest. Find:
a. the maximum elongation in the spring.
b. the maximum speed of the block m. Neglect the mass of the spring and that of the string. Also neglect the friction.
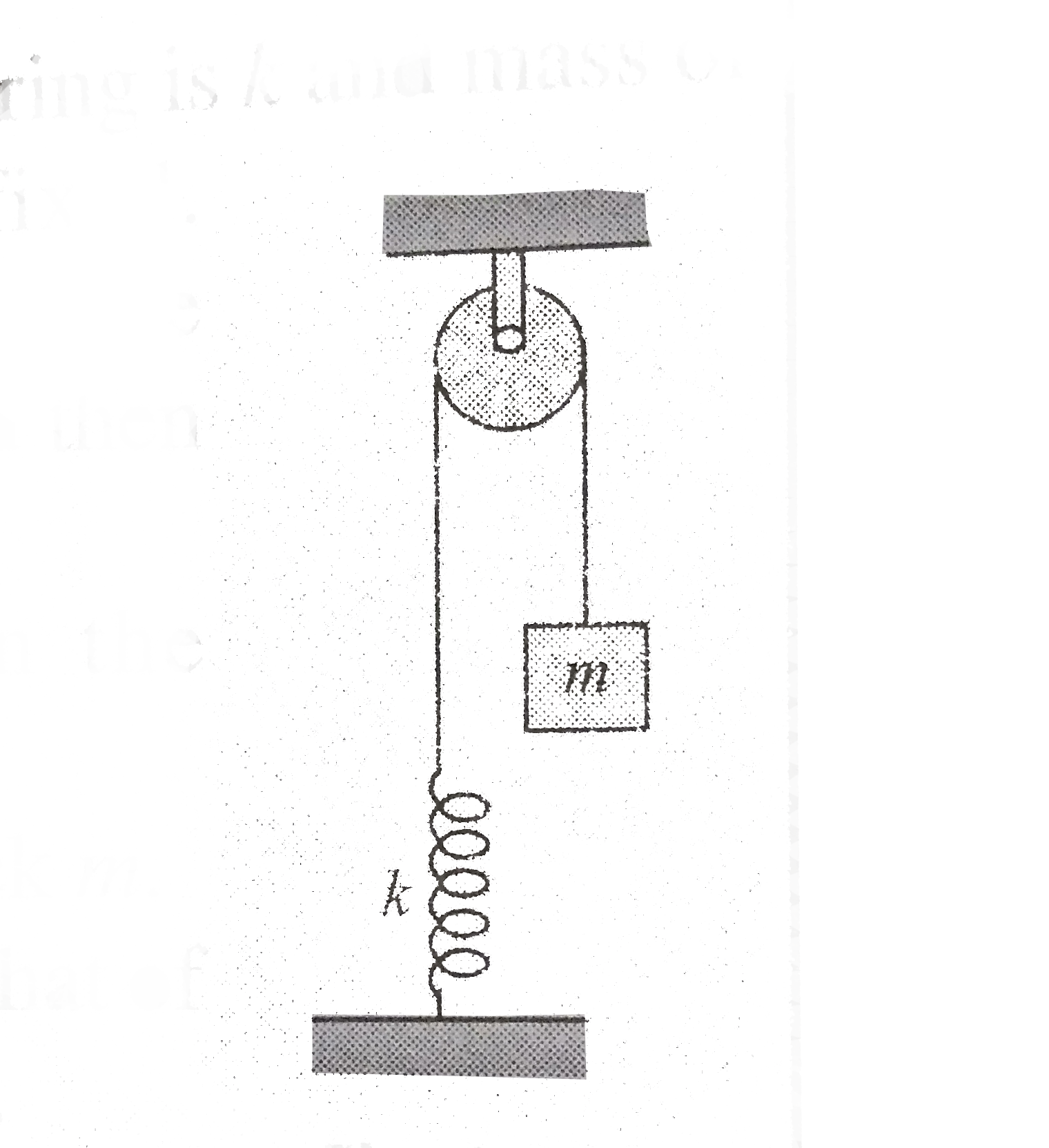
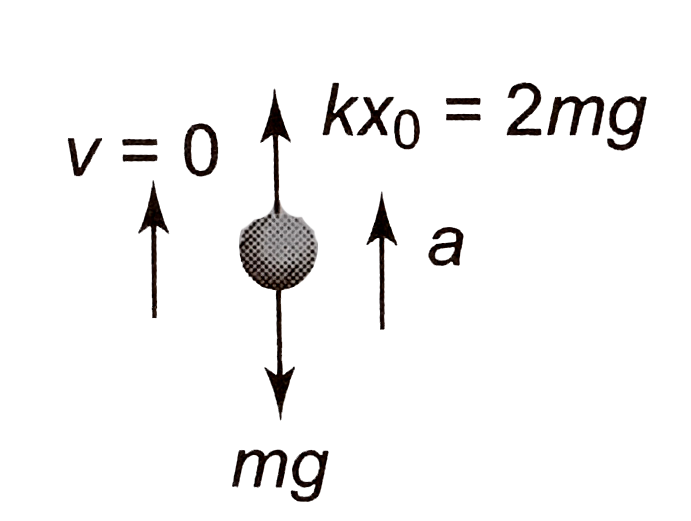
When the ball goes down by x0, spring extends by x0,
between (1) and (2)
Loss in gravitaion potential of ball = gain in energy of spring
mgx0=12kx20⇒x02mgx0
maximum extension of spring =2mgx0
Most of the students answer maximum extension x0=mgk0
Which is wrong
When the ball is released, the spring extends. Let at some instant is x.
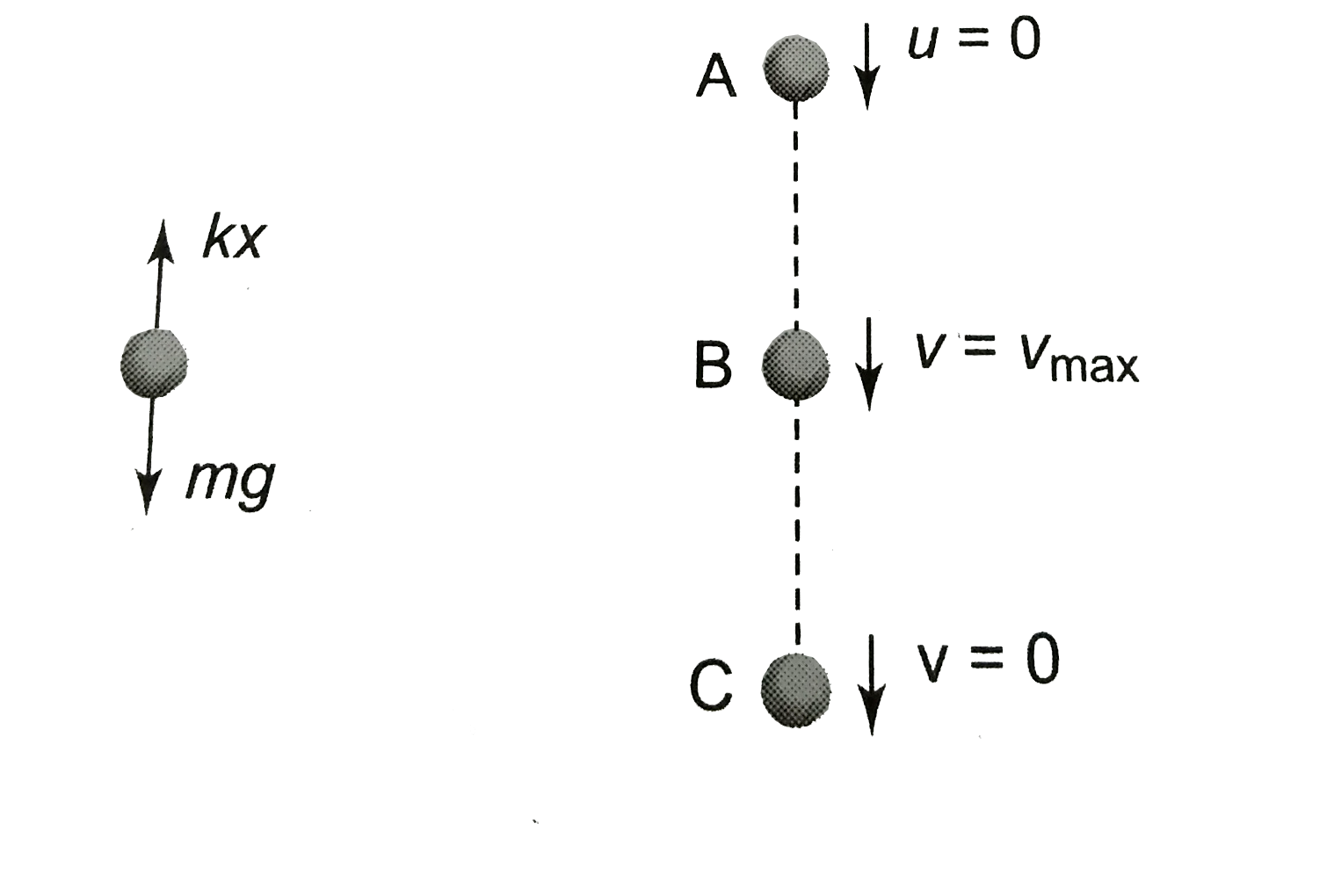
Now analyze the motion of ball:
A to B mg>kx, velocity of ball increases from 0 to vmax ltbRgt At B: mg=kξmpliesx=mgk=x0 the ball is in equilibrium,
velocity of the ball is maximum
B to C: mg<kx, velocity of the ball decreases to zero, the ball comes rest for a moment.
At C:

From C, the ball goes up and so on.
Topper's Solved these Questions
Similar Questions
The block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionBlock of mass 2m is given v0 towards the right. If L is the natural length of spring constant k, find the maximum elongation of the spring.

View Solution
Knowledge Check
For shown situation find the maximum elongation in the spring. Neglect friction everywhere. Initiallthe blocks are at rest and spring is unstreched.
 A4F3KB3F4KC4FKD2FK
A4F3KB3F4KC4FKD2FKA system of wedge and block as shown in figure, is released with the spring in its natural length. All surfaces are frictionless. Maximum elongation in the spring will be
 . A2mgsinθKBmgsinθKC4mgsinθKDmgsinθ2K
. A2mgsinθKBmgsinθKC4mgsinθKDmgsinθ2KFind the maximum tension in the spring if initially spring at its natural length when block is released from rest.
 AmgBmg/2C3mg/2D2 mg
AmgBmg/2C3mg/2D2 mg
A block of mass m held touching the upper end of a light spring of force constant K as shown in figure. Find the maximum potential energy stored in the spring if the block is released suddenly on the spring.

View SolutionThe block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
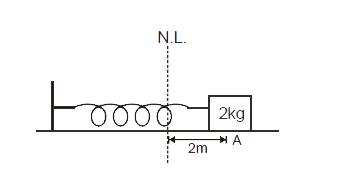
View SolutionIn the figure shown masses of the blocks A, B and C are 6kg, 2kg and 1kg respectively. Mass of the spring is negligibly small and its stiffness is 1000 N//m. The coefficient of friction between the block A snd the table is μ=0.8. Initially block C is held such that spring is in relaxed position. The block is released from rest. Find (g=10m/s2).
(a) the maximum distance moved by the kinetic C.
the acceleration of each block, when clongation in the spring is maximum.
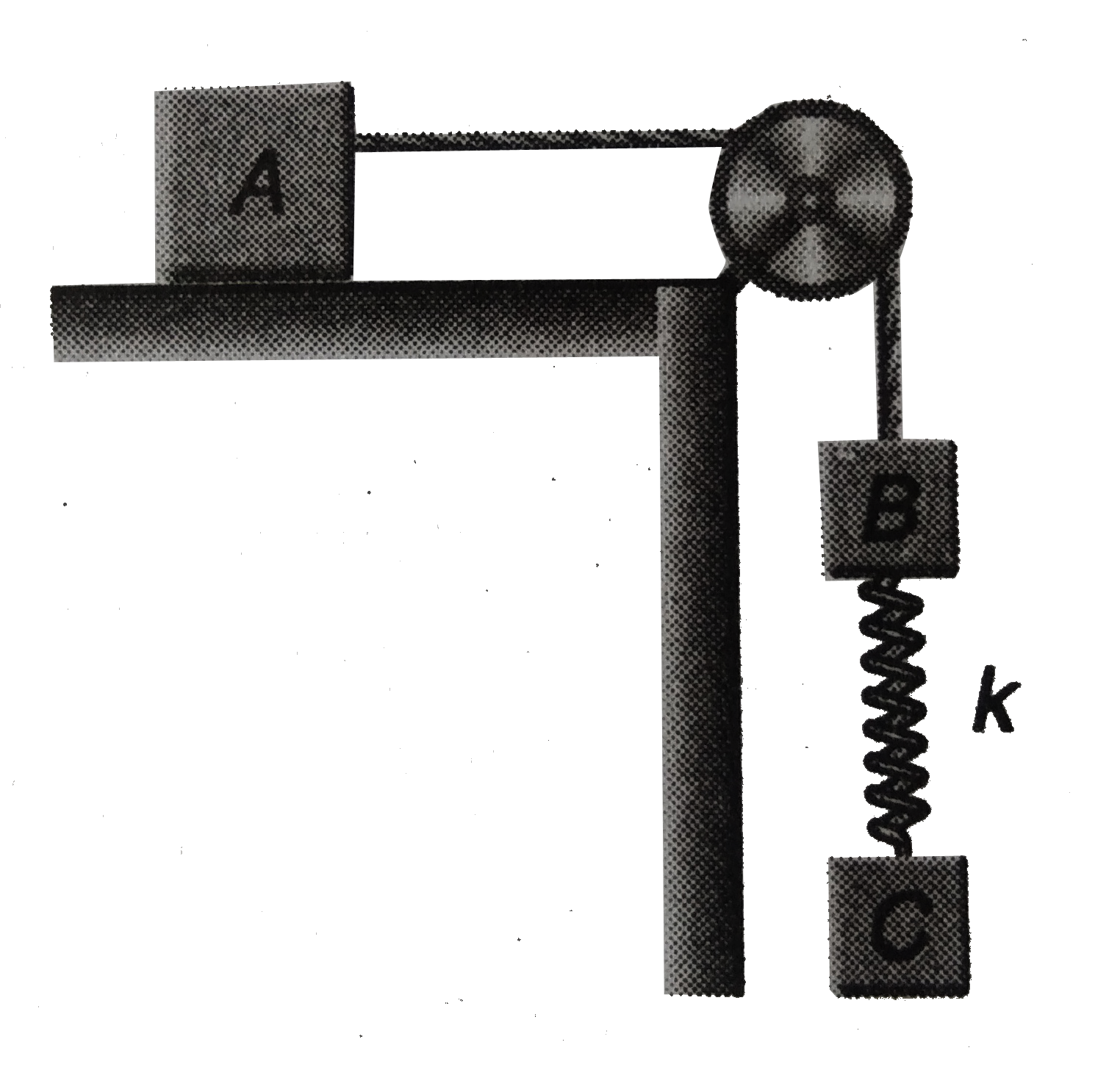
View SolutionInitially mass m is held such that spring is in relaxed condition. If mass m is suddenly released, maximum elongation in spring will be
View SolutionA disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:

View Solution
