Two satellites S1 and S2 are revolving around the earth in coplanar concentric orbits in the opposite sense. At t=0, the position of satellites are shown in the diagram. The periods of S1 and S2 are 4h and 24h, respectively. The radius of orbit of S1 is 1.28×104km. For this situation, mark the correct statement (s).
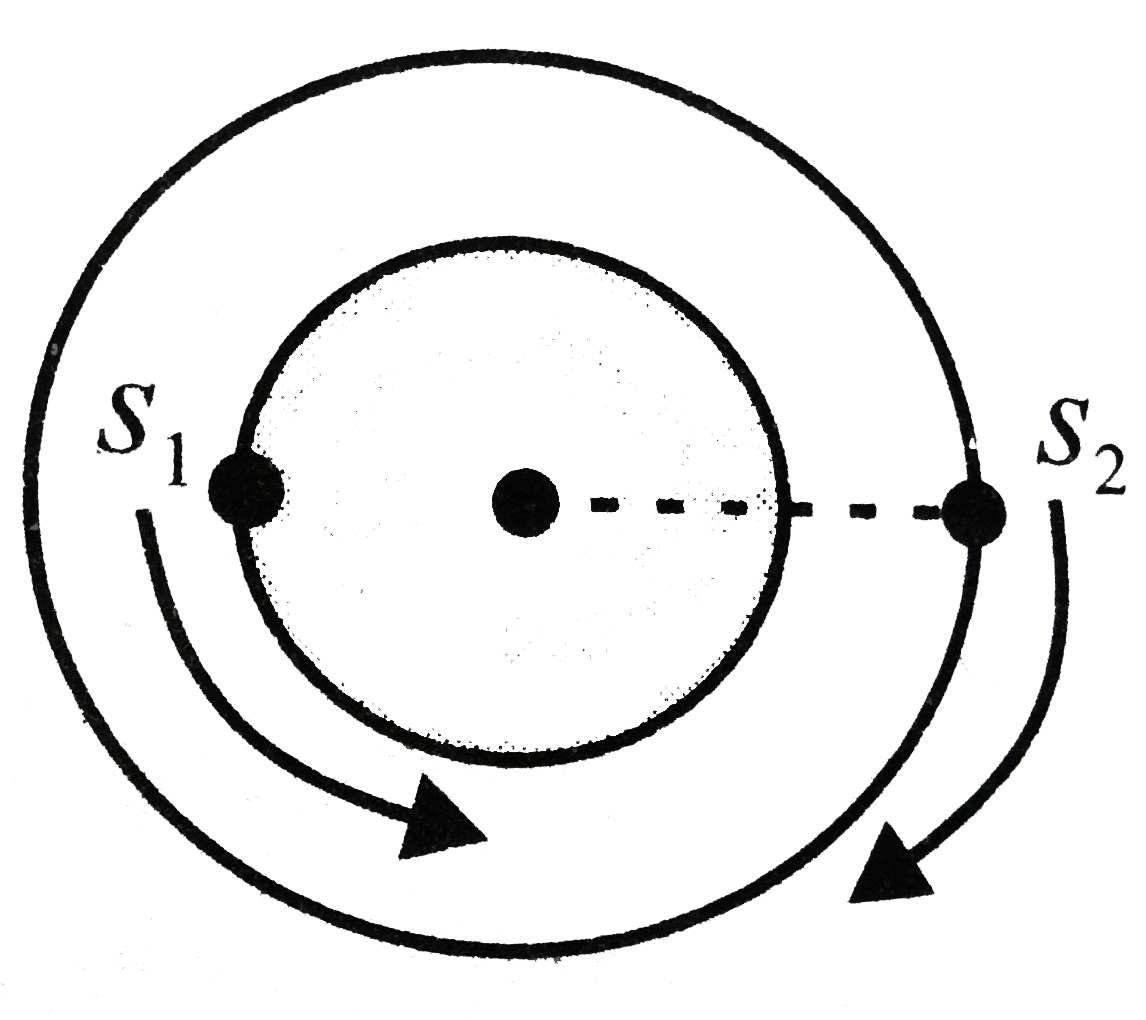
The angular velocity of S2 as observed by S1 at t=12h is 0.486πrads−1
The two satellites are closest to each other for the first time at t=12h and then after every 2h they are closest to each other
The orbital velocity of S1 is 0.64π×104km
The velocity of S1 relative to S2 is continuously changing in magnitude and direction both
The correct Answer is:A, B, C, D
From Kepler's law T2∝R3. So, T1T2=(R1R2)32
R2=(T2T1)23×R1=(246)23×1.28×104km
=3.22×104km orbital velocity of S1 is v1=2πR1T1=2π×1.28×1044=0.64π×104km
Orbital velocity of S2 is
v2=2πR2T2=2π×1.28×1044=0.64π×104km. At t=12h the two satellites are closest to each other and after every 24h they come at same position relative to each other. it is clear that direction of v2 w.r.t v1 is changing continuously in both magnitude and direction.
Angular velocity of S2 w.r.t. S1 at t=12h is ω=v1+v2/R2−R1=0.469πrads−1. this can be easily proved by writing the basic equations.
Related Playlists
- BOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL-VI42videos
GRAVITATION
- BOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - ILLUSTRATION49videos
GRAVITATION
- BOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL-II (H.W)32videos
GRAVITATION
- BOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I6videos
FRICTION
- BOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)52videos
KINETIC THEORY OF GASES
Similar Questions
Two satellites S1 and S2 are revolving around a planet in the opposite sense in coplanar circular concentric orbits. At time t = 0, the satellites are farthest apart. The periods of revolution of S1 and S2 are 3 h and 24 h respectively. The radius of the orbit of S1 is 3×104 km. Then the orbital speed ofS2 as observed from
View SolutionTwo satellites S1 and S2 are revolving around a planet P in circular orbits of radii 4R and 9R respectively. If the speed of satellite S1 is 2V, then calculate the speed of satellite S2.
View SolutionA satellite of mass Ms is revolving around the earth (Mass M) in a orbit of radius R. Find its angular momentum.
View SolutionTwo satellites S1 and S2 are revolving round a planet in coplanar and concentric circular orbit of radii R1 and R2 in te same direction respectively. Their respective periods of revolution are 1 hr and 8 hr. the radius of the orbit of satellite S1 is equal to 104km. Find the relative speed in kmph when they are closest.
View SolutionTwo satellite S1andS2 revolve round a planet in coplanar circular orbits in the same sense. Their periods of revolution are 1hr and 8hours respectively. The radius of the orbit of S1is104km. When S2 is closet to S1 (i) the speed S2 relative to S1 as actually observed by an astronaut in S1.
View SolutionTwo saetllites S1 and S2 revolve around a planet in coplaner circular orbit in the same sense. Their periods of revolutions are 1 hour and 8 hours respectively. The radius of orbit of S1 is 104km. When S2 is closed to S1, the speed of S2 relative to S1 is π×10nkm/h. what is the value of n?
View SolutionIf a satellite of mass 400 kg revolves around the earth in an orbit with speed 200 m/s then its potential energy is
View SolutionIf a satellite of mass 400 kg revolves around the earth in an orbit with speed 200 m/s then its potential energy is
View SolutionTwo satellites S1 and S2 revolve around the earth at distances 3R and 6R from the centre of the earth. Find the ratio of their (a) linear speeds and (b) angular speeds.
View SolutionTwo satellite S1 and S2 revolve around a planet in coplanar circular orbits in the opposite sense. The periods of revolutions are T and ηT respectively. Find the angular speed of S2 as observed by an astronouts in S1, are observed by an astronaut in S1, when they are closest to each other.
View Solution


